Courses

Courses for Kids

Free study material

Store

Talk to our experts

1800-120-456-456

Maths

Area of Shaded Region Explained with Examples

Area of Shaded Region Explained with Examples

Q: 1. How do you find the area of the shaded region sector?

To find the area of the shaded region sector, first calculate the area of the entire circle and then determine the area of the sector using the given central angle. The formula for the area of a sector is: $$\text{Area of sector} = \frac{\theta}{360^\circ} \times \pi r^2$$ where $\theta$ is the central angle in degrees and $r$ is the radius of the circle. If only a portion (the shaded part) of the sector is required, subtract or add relevant areas based on the problem's context. Vedantu’s expert math tutors can help you master such calculations and understand sector-based questions with step-by-step guidance.

Q: 2. What is the area of a shaded square?

The area of a shaded square depends on identifying which part of the square is shaded. For a fully shaded square, use the formula: $$\text{Area of square} = a^2$$ where $a$ is the side length. If only a portion is shaded (for example, the intersection with another shape), first find the total area of the square, then subtract or add the areas of any other shapes as needed. Vedantu’s resources provide detailed explanations and practice problems to help students accurately calculate the area of shaded squares in various scenarios.

Q: 3. What is the area of the shaded region in 3rd grade?

In 3rd grade math, students usually find the area of simple shaded regions by counting unit squares or using basic formulas. For example: If a rectangle is divided and only a portion is shaded, count the shaded squares.If the shape is regular (like a square or rectangle), multiply length by width for the shaded part. At Vedantu, interactive lessons and worksheets guide students step-by-step to develop a clear understanding of basic area concepts in shaded region problems.

Q: 4. How to find the area of a shaded region with variables?

To find the area of a shaded region with variables, use algebraic expressions to represent unknown lengths or sides. For example, if a rectangle has a length $l$ and width $w$, and the shaded area is a portion with a length $x$, the area may be: $$\text{Area} = x \times w$$ Use appropriate formulas and substitute the given variable values as needed. Vedantu’s subject experts provide personalized guidance on setting up algebraic equations and solving them for shaded region problems involving variables.

Q: 5. What is the formula for the area of a shaded region between two circles?

The area of the shaded region between two concentric circles (a ring or annulus) is found by subtracting the area of the smaller circle from the larger one: $$\text{Area of shaded region} = \pi R^2 - \pi r^2 = \pi (R^2 - r^2)$$ where $R$ is the radius of the larger circle and $r$ is the radius of the smaller circle. Vedantu offers comprehensive lessons with visuals and problem-solving strategies to help students understand annulus and other compound area problems.

Q: 6. How do you calculate the area of an irregular shaded region?

To calculate the area of an irregular shaded region, break down the region into familiar shapes such as rectangles, triangles, or circles. Compute the area of each part using standard formulas, then either sum or subtract these values according to the shading. For complex shapes, Vedantu’s interactive sessions guide learners in decomposing figures for accurate calculations and provide practice resources tailored to each student's needs.

Q: 7. Can you find the area of a shaded region using coordinate geometry?

Yes, coordinate geometry (or Cartesian geometry) can be used to find the area of a shaded region. By identifying the vertices' coordinates and applying formulas like the shoelace formula for polygons, you can determine areas precisely: $$\text{Area} = \frac{1}{2} \left| \sum_{i=1}^{n} (x_i y_{i+1} - x_{i+1} y_i) \right|$$ Vedantu’s expert tutors offer targeted guidance on using coordinate geometry concepts and solving shaded area problems step by step.

Q: 8. What real-life examples help understand area of shaded regions?

Several real-life examples make the concept of shaded area practical and relatable, such as: Measuring painted versus unpainted portions on a wallCalculating the area of a garden bed within a lawnFinding out usable space on patterned tiles or carpets Vedantu’s curriculum integrates real-world scenarios into lessons, helping learners apply area concepts to everyday situations for deeper understanding.

Reviewed by:

Rama Sharma

Latest Updates

Book Free Demo :

One-to-One Learning - Any Topic, Any Time

How to Calculate the Area of Shaded Regions in Maths

The area of the shaded region is basically the difference between the area of the complete figure and the area of the unshaded region. For finding the area of the figures, we generally use the basic formulas of the area of that particular figure. There is no specific formula to find the area of the shaded region of a figure as the amount of the shaded part may vary from question to question for the same geometric figure.

What is Area ?

Area is basically the amount of space occupied by a figure. The unit of area is generally square units; it may be square meters or square centimeters and so on.

Formula for Area of Geometric Figures :

Area of a square \[ = \] side \[ \times \] side
Area of a rectangle \[ = \] length \[ \times \] breadth
Area of a triangle \[ = \dfrac{1}{2} \times \] base \[ \times \] height
Area of a circle \[ = \] \[\pi {{\rm{r}}^2}\]
Area of a parallelogram \[ = \] base \[ \times \] height
Area of a trapezium \[ = \dfrac{1}{2}\] \[ \times \]sum of the length of the parallel sides \[ \times \] height
Area of rhombus \[ = \dfrac{1}{2}\] \[ \times \] product of the diagonals

What is the Area of the Shaded Region ?

In a given geometric figure if some part of the figure is coloured or shaded, then the area of that part of figure is said to be the area of the shaded region.

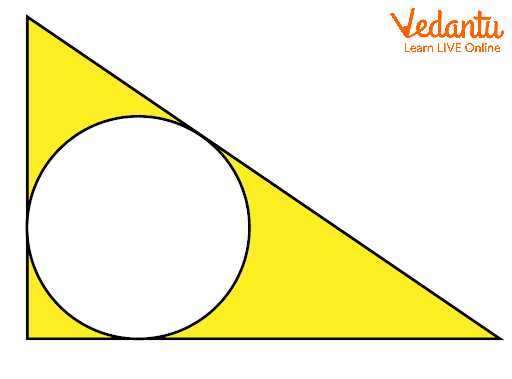
Example on how to Find Area of the Shaded Region

How to Find the Area of the Shaded Region?

So, the area of the shaded or coloured region in a figure is equal to the difference between the area of the entire figure and the area of the part that is not coloured or not shaded.

Area of the shaded region \[ = \] Area of the complete figure \[ - \] Area of the unshaded part

In the above image, if we are asked to find the area of the shaded region; we will calculate the area of the outer right angled triangle and then subtract the area of the circle from it. The remaining value which we get will be the area of the shaded region.

Solved Examples :

1. Find the area of the shaded region from the given figure :

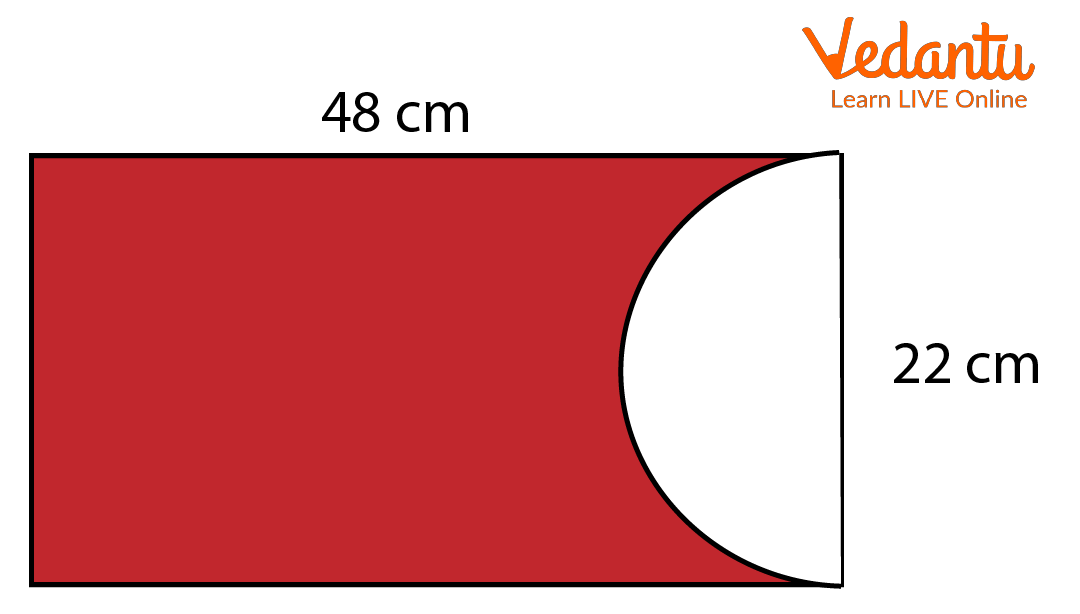
Area of the Shaded Region

Solution :

Here, the length of the given rectangle is 48 cm and the breadth is 22 cm.

We can observe that the outer rectangle has a semicircle inside it. From the figure we can observe that the diameter of the semicircle and breadth of the rectangle are common.

So from this figure we can understand that the area of the shaded region for this figure will be equal to the difference between the area of the outer rectangle and the area of the inner semicircle.

We know that, Area of rectangle = length \[ \times \] breadth

∴ Area of the given rectangle = 48 cm \[ \times \] 22 cm \[ = 1056\] sq.cm

Also, Area of a semicircle = \[\dfrac{1}{2}\pi {r^2}\]

Here, diameter \[ = 22\] cm

So, Radius \[ = \dfrac{1}{2} \times \] Diameter \[ = \dfrac{1}{2} \times 22 = 11\]cm

Area of the given semicircle \[ = \dfrac{1}{2} \times \dfrac{{22}}{7} \times 11 \times 11 = \dfrac{{2662}}{{14}} = 190.14\]sq.cm

Therefore, Area of the shaded region = Area of rectangle - Area of semicircle

So, Area of the shaded region in the given figure = 1056 - 190.14 = 865.86 sq.cm

Area of the shaded region in the given figure is 865.86 sq.cm.

2. Find the area of the shaded region from the given figure :

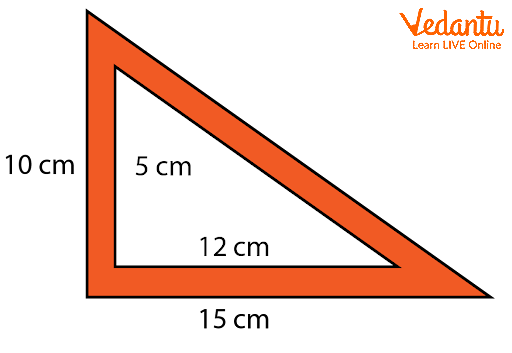
Area of the Shaded Region

Solution :

Here, the base of the outer right angled triangle is 15 cm and its height is 10 cm.

Similarly , the base of the inner right angled triangle is given to be 12 cm and its height is 5 cm.

We can observe that the outer right angled triangle has one more right angled triangle inside.

So from this figure we can understand that the area of the shaded region for this figure will be equal to the difference between the area of the outer triangle and the area of the inner triangle.

We know that, Area of a triangle \[ = \] \[\dfrac{1}{2}\] \[ \times \] base \[ \times \] height

∴ Area of the outer right

angled triangle \[ = \dfrac{1}{2} \times 15 \times 10 = \dfrac{1}{2} \times 150 = 75\] sq.cm.

Similarly, area of the inner

right angled triangle \[\dfrac{1}{2} \times 12 \times 5 = \dfrac{1}{2} \times 60 = 30\]sq.cm.

Therefore, Area of the shaded

region = Area of the outer triangle - Area of the inner triangle

We know that, Area of a triangle \[ = \dfrac{1}{2} \times {\rm{base}}\times{\rm{height}}\]

∴ Area of the outer right angled triangle \[ = \dfrac{1}{2} \times 15 \times 10 =\dfrac{1}{2}\times 150 = 75\] sq.cm.

Similarly,

area of the inner right angled triangle \[\dfrac{1}{2} \times 12 \times 5 = \dfrac{1}{2} \times 60 = 30\] sq.cm.

Therefore, Area of the shaded region \[ = \] Area of the outer triangle \[ - \] Area of the triangle. So, Area of the shaded region in the given figure \[ = 75 - 30 = 45\] sq.cm

Area of the shaded region in the given figure is 45 sq.cm.

3. Find the area of the shaded region from the given figure :

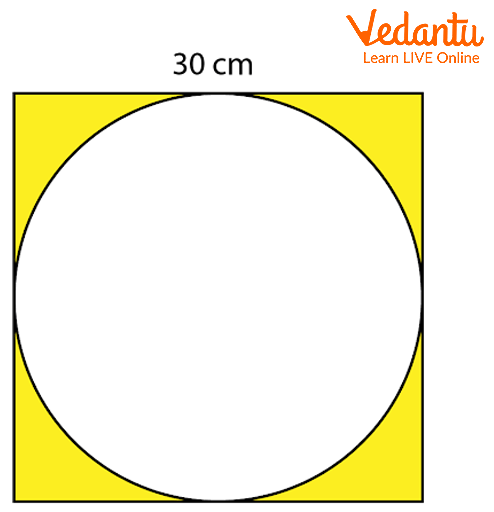
Area of the Shaded Region

Solution :

Here, the length of the side of the given square is 30 cm.

We can observe that the outer square has a circle inside it. From the figure we can see that the value of the side of the square is equal to the diameter of the given circle.

So from this figure we can understand that the area of the shaded region for this figure will be equal to the difference between the area of the outer square and the area of the inner circle. We know that, Area of square = side x side

∴ Area of the given square \[ = \] 30 cm \[ \times \] 30 cm \[ = \] 900 sq.cm

Also, Area of a circle \[ = \pi {r^2}\]

Here, diameter \[ = 30\] cm

So, Radius \[ = \dfrac{1}{2} \times \] Diameter \[ = \dfrac{1}{2} \times 30 = 15\]cm

Area of the given circle\[ = \dfrac{{22}}{7} \times 15 \times 15 = 49507 = 707.14\] sq.cm

\[ = \dfrac{{22}}{7} \times 15 \times 15 = \dfrac{{4950}}{7} = 707.14\] sq.cm

Therefore, Area of the shaded region = Area of square - Area of circle

So, Area of the shaded region in the given figure \[ = 900 - 707.14 = 192.86\] sq.cm

Area of the shaded region in the given figure is 192.86 sq.cm.

Conclusion

The area of the shaded region is in simple words the area of the coloured portion in the given figure. So, the ways to find and the calculations required to find the area of the shaded region depend upon the shaded region in the given figure.

FAQs on Area of Shaded Region Explained with Examples

1. How do you find the area of the shaded region sector?

To find the area of the shaded region sector, first calculate the area of the entire circle and then determine the area of the sector using the given central angle. The formula for the area of a sector is:
$$\text{Area of sector} = \frac{\theta}{360^\circ} \times \pi r^2$$ where $\theta$ is the central angle in degrees and $r$ is the radius of the circle. If only a portion (the shaded part) of the sector is required, subtract or add relevant areas based on the problem's context. Vedantu’s expert math tutors can help you master such calculations and understand sector-based questions with step-by-step guidance.

2. What is the area of a shaded square?

The area of a shaded square depends on identifying which part of the square is shaded. For a fully shaded square, use the formula:
$$\text{Area of square} = a^2$$ where $a$ is the side length. If only a portion is shaded (for example, the intersection with another shape), first find the total area of the square, then subtract or add the areas of any other shapes as needed. Vedantu’s resources provide detailed explanations and practice problems to help students accurately calculate the area of shaded squares in various scenarios.

3. What is the area of the shaded region in 3rd grade?

In 3rd grade math, students usually find the area of simple shaded regions by counting unit squares or using basic formulas. For example:

If a rectangle is divided and only a portion is shaded, count the shaded squares.
If the shape is regular (like a square or rectangle), multiply length by width for the shaded part.

At Vedantu, interactive lessons and worksheets guide students step-by-step to develop a clear understanding of basic area concepts in shaded region problems.

4. How to find the area of a shaded region with variables?

To find the area of a shaded region with variables, use algebraic expressions to represent unknown lengths or sides. For example, if a rectangle has a length $l$ and width $w$, and the shaded area is a portion with a length $x$, the area may be:
$$\text{Area} = x \times w$$ Use appropriate formulas and substitute the given variable values as needed. Vedantu’s subject experts provide personalized guidance on setting up algebraic equations and solving them for shaded region problems involving variables.

5. What is the formula for the area of a shaded region between two circles?

The area of the shaded region between two concentric circles (a ring or annulus) is found by subtracting the area of the smaller circle from the larger one:
$$\text{Area of shaded region} = \pi R^2 - \pi r^2 = \pi (R^2 - r^2)$$ where $R$ is the radius of the larger circle and $r$ is the radius of the smaller circle. Vedantu offers comprehensive lessons with visuals and problem-solving strategies to help students understand annulus and other compound area problems.

6. How do you calculate the area of an irregular shaded region?

To calculate the area of an irregular shaded region, break down the region into familiar shapes such as rectangles, triangles, or circles. Compute the area of each part using standard formulas, then either sum or subtract these values according to the shading. For complex shapes, Vedantu’s interactive sessions guide learners in decomposing figures for accurate calculations and provide practice resources tailored to each student's needs.

7. Can you find the area of a shaded region using coordinate geometry?

Yes, coordinate geometry (or Cartesian geometry) can be used to find the area of a shaded region. By identifying the vertices' coordinates and applying formulas like the shoelace formula for polygons, you can determine areas precisely:
$$\text{Area} = \frac{1}{2} \left| \sum_{i=1}^{n} (x_i y_{i+1} - x_{i+1} y_i) \right|$$ Vedantu’s expert tutors offer targeted guidance on using coordinate geometry concepts and solving shaded area problems step by step.

8. What real-life examples help understand area of shaded regions?

Several real-life examples make the concept of shaded area practical and relatable, such as:

Measuring painted versus unpainted portions on a wall
Calculating the area of a garden bed within a lawn
Finding out usable space on patterned tiles or carpets

Vedantu’s curriculum integrates real-world scenarios into lessons, helping learners apply area concepts to everyday situations for deeper understanding.

9. What are the common mistakes when solving area of shaded region problems?

Common mistakes include:

Incorrectly identifying which areas are shaded
Using the wrong formula for a given shape
Forgetting to subtract areas when required
Misreading dimensions or ignoring unit conversions

In Vedantu’s classes, students receive personalized feedback and tips to avoid these errors, ensuring strong conceptual and calculation skills.

10. How does Vedantu help students master shaded region area problems?

Vedantu provides:

Live interactive classes with expert tutors
Step-by-step solutions for a variety of shaded region problems
Practice worksheets and quizzes designed to solidify understanding
Visual aids and real-world application exercises

These resources allow students to develop a thorough and confident approach to calculating areas of shaded regions across different shapes and difficulty levels.