Chords in Parabola and Focal Chords

A parabola is a U-shaped plane curve where any point is equally spaced from a set point (known as the focus) and from a fixed straight line (known as the directrix). The topic of conic sections includes a parabola, and its principles are discussed here. The shape of the banana is like a parabola. A parabola is emerging from the rainbow. Antennas with a parabolic dish are like a parabola. Mirror's concave surface.

In this article, we will learn about chords in parabolas and their properties, such as focal chords, the equation of the chord of a parabola, parabola focal length, the focal distance of parabola formula, the diameter of a parabola, etc. 

Parametric Equation of Parabola

If the equation of the parabola is $y^{2}=4ax$, then the parametric Equation will be $x=at^{2},y=2at$ where t is the parameter. Any point on the parabola can be represented by the coordinates $(at^{2},2at)$.

Equation of Chord of Parabola

If we see the below diagram, we can assume the points P and Q as $(at^{2},2at)$ and $(at_{1}^{2},2at_{1})$ respectively. Now, we can calculate its equation using 2 point formula, i.e. $(2at-2at_{1})=m(at^{2}-at_{1}^{2})$ where m is the slope of the chord. $m=\dfrac{2at-2at_{1}}{at^{2}-at_{1}^{2}}=\dfrac{2}{t_{1}+t_{2}}$.

Equation of Chord of Parabola

Chord of Contact of Parabola

If the equation of the parabola is $y^{2}=4ax$ and tangents are drawn from point $P(x_{1},y_{1})$ outside the parabola then the equation of the chord of contact will be calculated using T=0 i.e $yy_{1}=4a(\dfrac{x+x_{1}}{2})$.

Focal Chord of Parabola

When a chord passes through the focus of a parabola then it is called a focal chord. As we can see in the above diagram the chord PQ is a focal chord and we know that the line PS and SQ have the same slope. So, 

$\Rightarrow\dfrac{2at-0}{at^{2}-a} =\dfrac{0-2at_{1}}{a-at_{1}^{2}}$

$\Rightarrow\dfrac{2at}{a(t^{2}-1)} =\dfrac{2at_{1}}{a(t_{1}^{2}-1)}$

$\Rightarrow\dfrac{t}{t^{2}-1}=\dfrac{t_{1}^{2}}{t_{1}^{2}-1} $

$\Rightarrow t_{1}^{2}t-t=t^{2}t_{1}-t_{1}$

$\Rightarrow t_{1}(t_{1}t+1)=t(t_{1}t+1) $

$\Rightarrow t_{1}(t_{1}+1)-t(t_{1}t+1)=0 $

$\Rightarrow (t_{1}t+1)(t_{1}-t)=0 $

$Hence, tt_{1}=-1 or t_{1}=\dfrac{-1}{t}$

Focal Distance of Parabola

Focal distance is the length of a point on the parabola to the focus of that parabola. In the below-given figure, the focal distance is PS where P is a random point on that parabola and S is the focus. Now, we know that PS=PM and $PM=at^{2}+a=a(1+t^{2})$

$\Rightarrow PM=a(1+t^{2})$= Focal distance of parabola 

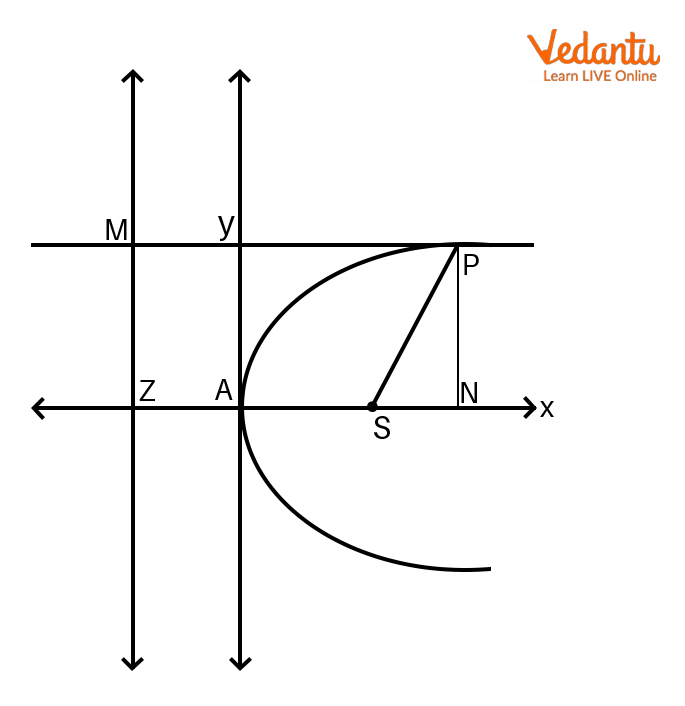
Focal Distance of Parabola

Length of Focal Chord of Parabola

Here, in the figure given below, the length of the focal chord will be PQ and it can be represented as $PS+SQ=a(1+t^{2})+a(1+t_{1}^{2})$

$\Rightarrow a(1+t^{2})+a(1+(\dfrac{-1}{t^{2}}))$ as $t_{1}=\dfrac{-1}{t}$

$\Rightarrow a(1+t^{2}) $

Equation of Chord of Parabola

How to Find the Focus of a Parabola?

If the equation of the parabola is $(x-h)^{2}=4a(y-k)$ then the focus of the parabola will be at $(h,k+a)$ and if the equation of the parabola is $(y-k)^{2}=4a(x-h)$ then the focus of parabola formulas is $(h+a,k)$.

Diameter of Parabola

The locus of the middle point of the chords of the parabola having the same slope is called the diameter of the parabola and the equation of diameter of a parabola if the equation of a parabola is $y^{2}=4ax$ is $y=\dfrac{2a}{m}$ where m is the slope of the parallel chords.

Solved Examples

Q1. Find the length of the focal chord to the parabola $y^{2}=5x$ drawn from (5.-5).

Solution: Given, $y^{2}=4ax$

$\Rightarrow y^{2}=4\times \dfrac{5}{4}\times x$

$\Rightarrow a=\dfrac{5}{4} $

We know that parametric coordinates are $(at^{2},2at)$. So, to calculate the value of t,

$2at=-5$

$\Rightarrow 2\left ( \dfrac{5}{4} \right ) t=-5$

$\Rightarrow t=-2$

So the length of the focal chord is $a(t+\dfrac{1}{t})^{2}$

$=\dfrac{5}{4}(-2+(\dfrac{-1}{2}))^{2}$

$=\dfrac{5}{4}(\dfrac{25}{4})=\dfrac{125}{16}$

Q2. Find the equation of the chord of contact from point (-5,6) to the parabola

$1)y^{2}=3x$

$2)x^{2}=-4y$

Solution: We need to use T=0 to find the chord of contact. So,

$y(6)=3(\dfrac{x-5}{2})$

$\Rightarrow 4y=x-5 $

2.$x(-5)=4(\dfrac{y+6}{2})$

$\Rightarrow -5x=2y+12$

Conclusion

The article summarizes the concept of chords and focal chords in parabolas. We understood the concepts of focal chords, the equation of the chord of parabola, parabola focal length, the focal distance of parabola formula, diameter of the parabola and learned their formulas.

Practice Questions

Q1. The length of the chord of the parabola $x^{2}=4y$ having equation $x-\sqrt{2}y+4\sqrt{2}=0$ is

Q2. If one end of a focal chord of the parabola, $y^{2}=16x$ is at (1,4) then the length of this focal chord is

Q3. Let $y=mx+c$, m>0 be the focal chord of $y^{2}=-64x$, which is tangent to $(x+10)^{2}+y^{2}=4$. Then the value of $4\sqrt{2}(m+c)$ is equal to

Answer: 

1. $6\sqrt{3}$

2. 25

3. 34

List of Related Articles

• Parabola General Equation

• Ellipse

• Conic Sections