




Subset: An Introduction
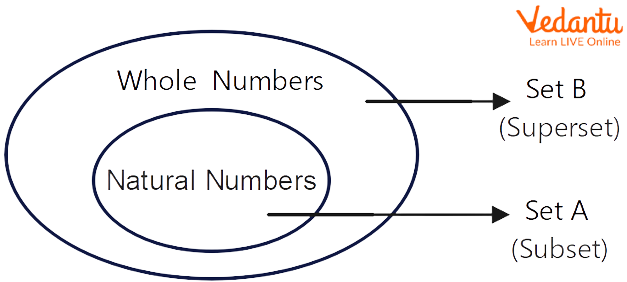
In mathematics, we define the subset as showing that all the elements of some set A are contained within some other set B. There are two types of subsets, proper subsets and normal subsets.
If all elements of set A are in another set B, then set A is said to be a subset of set B.
Subsets
What is a Subset in Maths?
Set “a” is said to be a subset of set “b” if all the elements of set “a” are present in set “b”. In alternative words, set “a” is contained within set “b”.
Example: if set “a” has {x, y} and set “b” has {x, y, z}, then “a” is the subset of “b” as elements of “a” are present in set “b”.
Subset Symbol
A subset is denoted by the symbol ⊆.
A ⊆ b;
which means set A is a subset of set b.
All Subsets of a Set
The subsets of any set contain all possible sets, including its components and the null set. Let us understand with the help of an example.
Example: find all the subsets of set a = {1,2,3,4}
Solution: given, a = {1,2,3,4}
Subsets = {}
{1}, {2}, {3}, {4},
{1,2}, {1,3}, {1,4}, {2,3} ,{2,4}, {3,4},
{1,2,3}, {2,3,4}, {1,3,4}, {1,2,4}
{1,2,3,4}.
Types of Subsets
Subsets are classified as:
Proper subset
Improper subsets
For example, if set a = {2, 4, 6}, then,
Number of subsets: {2}, {4}, {6}, {2,4}, {4,6}, {2,6}, {2,4,6} and φ or {}.
Proper subsets: {}, {2}, {4}, {6}, {2,4}, {4,6}, {2,6}
Improper subset: {2,4,6}
There is no specific formula to find the subsets. Instead, we've to list them to differentiate between proper and improper ones.
Proper Subsets
Set a is considered a proper subset of set b if set b contains a minimum of one component not present in set a.
Example: if set a has elements as {12, 24} and set b has elements as {12, 24, 36}, then set a is the proper subset of b as 36 is not present within set a.
Proper Subset Symbol
A proper subset is denoted by ⊂. We will express a proper subset for set a and set b as,
A ⊂ b
Formula
If a set has “n” components, then the number of subsets of the given set is 2n, and conjointly the number of proper subsets of the given subset is given by 2n-1.
Consider an example, if set a has the elements A = {a, b},
then the proper subset of the given set is { }, {a}, and {b}.
Here, the number of components within the set is 2.
We know that the formula to calculate the number of proper subsets is 2n – 1.
= 22 – 1
= 4 – 1
= 3
Thus, the number of proper subsets for the given set is 3 ({ }, {a}, {b}).
What is an Improper Subset?
A subset that contains all the elements of the initial set is named an improper subset of the initial set. it's denoted by ⊆.
For example:
set p ={2,4,6}
then, the subsets of p are;
{}, {2}, {4}, {6}, {2,4}, {4,6}, {2,6} and {2,4,6}.
Where, {}, {2}, {4}, {6}, {2,4}, {4,6}, {2,6} are the proper subsets and {2,4,6} is the improper subsets. Therefore, we can write {2,4,6} ⊆ p.
Power Set
The power set is said to be the collection of all the subsets. It's represented by p(A). If A is set having elements {a, b}.
Then the power set of “A” can be;
P(A) = {∅, {a}, {b}, {a, b}}.
Properties of Subsets
Some of the necessary properties of subsets are:
Every set is taken into account as a subset of the given set itself. It implies that x ⊂ x or y ⊂ y, etc.
We can say an empty set is considered a subset of each set.
X is a subset of y. It implies that x is contained in y.
If a set x could be a subset of set y, we can say that y may be a superset of x.
Solved Examples
1. How many subsets containing three elements can be formed from the set?
S = { 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 }
Ans: Number of elements within the set = 10
Number of parts within the subset = 3
Therefore, the number of possible subsets containing 3 parts= 10c3
$=\dfrac{10!}{(10-3)!.3!}$
$=\dfrac{10 \times 9 \times 8 \times 7!}{(7)!.3 \times 2 \times 1}$
$=\dfrac{720}{6}$
$=120$
Therefore, the number of possible subsets containing 3 parts from the set S = { 1, 2, 3, 4, 5, 6, 7, 8, 9, 10} is one hundred twenty.
2. Given any two real-life examples on the subset.
Ans: we can find a variety of examples of subsets in our way of life, such as:
If we consider all the books in a library as one set, then books relating to maths may be a subset.
If all the items in a grocery store form a collection, then cereals form a subset.
3. Find the number of subsets and the number of proper subsets for the given set A = {5, 6, 7, 8}.
Ans: Given: A = {5, 6, 7, 8}
The number of elements in the set is 4.
We know that,
The formula to calculate the number of subsets of a given set is 2n
= 24 = 16
The number of subsets is 16.
The formula to calculate the number of proper subsets of a given set is 2n – 1
= 24 – 1
= 16 – 1 = 15
The number of proper subsets is 15.
Conclusion
If a set has n no. of elements, then the no. of subsets of the given set is 2n, and the no. of proper subsets is 2n-1. In this article, What are a subset and its symbol, the properties of the subset, the definition of a power set and the types of subsets are explained with examples.
Practice Problems
Find the number of subsets and the number of proper subsets for the given set A = {5, 4, 2, 1, 0}.
Find the subsets for the given set A = {3, 4, 5, 6, 7}.
List of Related Articles
FAQs on Subsets
1. What are the different types of sets?
Sets are categorised into different types like an infinite set, singleton set, finite set, empty set etc. The set which has only one element is a singleton set. The set containing a finite number of elements or parts is called a finite set. Similarly, a set having infinite elements is called an infinite set.
In set theory, a set X is outlined as a subset of another set Y if all the elements of set X are present within the set Y. X ⊂ Y can symbolically represent this.
2. Define a universal set.
A universal set is a set which contains all the elements or objects of other sets, including its elements. The symbol ' U usually denotes it'.
3. What are equivalent and equal sets?
Any two sets are called equivalent sets when their cardinality or the number of components present is the same. example : A={ 2,3,6} , B={3,2,7} are equivalent sets. While in equal sets, the sets are called equal if they are equivalent and their elements are completely identical. For example, two sets A{3,6,7} and B {6,3,7} are equal, as they are equivalent sets having a same number of elements and identical elements.





































