




An Introduction to Fractions and Mixed Fractions
In Mathematics, fraction is used to show or represent a part of the entire thing. It can represent things as equal parts of the whole. In the representation of fractions, there are two parts namely numerator and denominator.
The number written on the top is known as the numerator. The number written on the bottom part is known as the denominator. The number of equal parts of a thing written on top is called the numerator. The denominator is the whole number in a fraction.
If $\dfrac{5}{10}$ is a fraction.
The number $5$ is a numerator, and $10$ is a denominator.
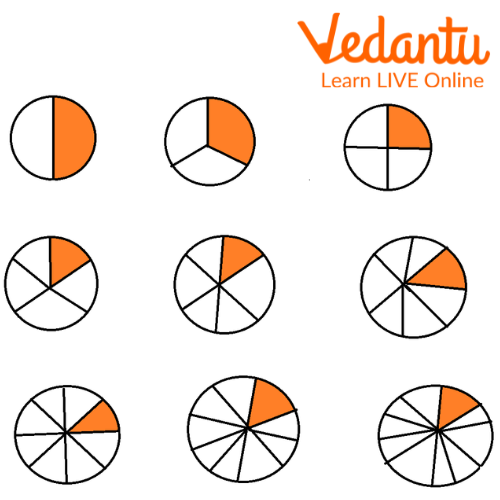
Visual Representation of a Fraction
Improper Fraction
The fractions in which the numerator value is larger than the denominator are known as improper fractions.
E.g. $\dfrac{7}{3}, \dfrac{9}{5}, \dfrac{7}{2}$
Mixed Fraction
It can be defined as the fraction made up of a combination of whole numbers and fractions.
e.g. $3 \dfrac{1}{2}, 5 \dfrac{1}{2}$
How to Convert an Improper Fraction into a Mixed Fraction?
There are certain steps to convert an improper fraction into a mixed fraction. These are discussed below.
First Step: We need to identify the improper fraction.
Second Step: Then, we need to divide the numerator by the denominator and thereby obtain the quotient and remainder.
Third Step: Now, the mixed fraction can be written as:
Quotient (Remainder/Denominator).
Simple Way to Convert Improper Fraction to Mixed Fraction
To convert an improper fraction to a mixed fraction, divide the numerator (upper portion) by the denominator in order to write an improper fraction as a mixed fraction (bottom part). The numerator is the remainder, and the quotient is the entire number.
Solved Examples
Example 1. Let us convert $\dfrac{7}{5}$ into a mixed fraction.
Ans: If in a fraction the numerator and denominator have the same number, it will make a whole.
In the case of $\dfrac{7}{5}, \dfrac{5}{5}$ can be extracted and that can be made a whole.
Therefore, the remaining fraction is $2 / 5$.
So, now we can write $\dfrac{7}{5}$ as the mixed fraction, and that is $1 \dfrac{2}{5}$
The fraction $\dfrac{7}{5}$ means that $7 \div 5$. Now we divide $7$ by $5$ and get $1$ as the quotient making $2$ as the remainder.
In order to convert an improper fraction into a mixed fraction, we need to use the quotient $1$ as the whole number and the remainder $2$ as the numerator whereas the divisor $5$ is used as the denominator of the proper fraction.
Mixed and Improper Fraction.
Example 2. Convert the improper fractions into mixed fractions:
(i) $\dfrac{17}{4}$
Ans: According to the question, the numerator is $17$, and the denominator is $4$
In order to convert the improper fraction into a mixed fraction, we divide the numerator with the denominator.
When we divide $17$ by $4$
Quotient = $4$, Remainder = $1$, Denominator = $4$.
Hence, $\dfrac{17}{4}=4 \dfrac{1}{4}$
(ii) $\dfrac{13}{5}$
Ans: According to the question, the numerator is $13$, and the denominator is $5$
In order to convert the improper fraction into a mixed fraction.
We divide the numerator with the denominator.
When we divide $13$ by $5$
Quotient = $2$, Remainder = $3$, Denominator = $5$.
Hence, $\dfrac{13}{5}=2 \dfrac{3}{5}$
(iii) $\dfrac{28}{5}$
Ans: According to the question, the numerator is $28$, and the denominator is $5$
In order to convert the improper fraction into a mixed fraction.
We divide the numerator with the denominator.
When we divide $28$ by $5$
Therefore, Quotient = $5$, Remainder = $3$, Denominator = $5$
Hence, $\dfrac{28}{5}=5 \dfrac{3}{5}$
(iv) $\dfrac{28}{9}$
Ans: According to the question, the numerator is $28$ and the denominator is $9$
In order to convert the improper fraction into a mixed fraction.
We divide the numerator with the denominator.
When we divide $28$ by $9$
The Quotient = $3$, Remainder = $1$, Denominator = $9$.
Hence, $\dfrac{28}{9}=3 \dfrac{1}{9}$
Practice Questions
Convert the following improper fractions into mixed fractions.
Q 1. Convert into mixed fractions:
(a) $\dfrac{7}{3}$
(b) $\dfrac{11}{7}$
(c) $\dfrac{13}{6}$
Ans: (a) $2^\dfrac{1}{3}$
(b) $\dfrac{14}{7}$
(c) $2^\dfrac{1}{6}$
Q 2. Write improper fraction of $\dfrac{3}{9}$
Ans: $\dfrac{1}{3}$
Summary
Fractions are representations of a single part out of many parts, and they can be in different forms either mixed or improper. Fractions can be converted into either form following a few steps. The steps include identifying an improper fraction, dividing the numerator by the denominator to get a quotient and remainder, and finally, the mixed fraction is written as Quotient (Remainder/Denominator).
Fractions are very useful in expressing a lot of things such as time, i.e. every minute is a part of an hour and every hour is a part of a day.
FAQs on Conversion of Improper Fractions Into Mixed Fractions
1. What is the need to convert an improper fraction into a mixed fraction?
Improper fractions need to be converted to mixed fractions because improper fractions are those in which the numerator value exceeds the denominator. A mixed fraction is one that combines both whole numbers and fractions. The primary purpose of this conversion procedure is to streamline calculations that call for incorrect fractions. But since mixed fractions are simpler to understand, we can use them to describe a result.
2. Convert an improper fraction into mixed fraction $\dfrac{35}{8}$
According to the question, the numerator is $35$ and the denominator is $8$.
In order to convert the improper fraction into a mixed fraction.
We divide the numerator with the denominator.
When we divide $35$ by $8$ , the Quotient = $4$, Remainder = $3$, Denominator = $8$.
Hence, the final result is $\dfrac{35}{8}=4 \dfrac{3}{8}$
3. Can mixed fractions be used in arithmetic operations?
Mixed fractions are those fractions in which a whole number is combined with a fraction. The mixed fractions can also be added, subtracted, multiplied or divided just like the integers. They need to be converted into a suitable improper fraction first and that makes the process easier to obtain. Thereafter, the operation processes are very similar to normal integer operation.











