




The Cube of A Binomial
Anything raised to the power 3 is known as a cube in arithmetic and algebraic terms which means to cube a number just multiply its three times.The cube of any number is denoted by superscript 3.The cube number is when a number is multiplied by twice itself.
A binomial is multiplied by itself three times to form the cube of a binomial.Thus we can say that the cube of any number ‘a ‘ can expressed as \[a \times a \times a\] or \[{a^3}\] is known as cube number. This binomial is an algebra expression consisting of two terms i.e., a+b or a-b and its cube can be expressed as \[(a - b) \times (a - b) \times (a - b)\] or
\[{(a - b)^3}\].
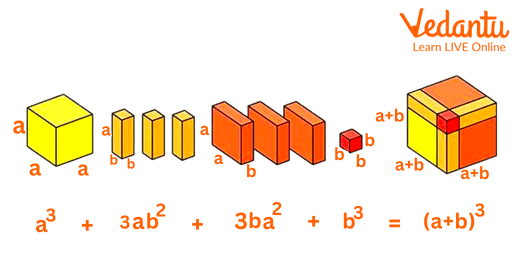
Cube of Binomial
Cube of The Difference of Two Binomials
The basic formula for the cube of difference of two binomial \[{(a - b)^3}\]is which can be expanded as follow:
\[\begin{array}{l}{(a - b)^3} = (a - b)(a - b)(a - b)\\\ = ({a^2} - 2ab + {b^2})(a - b)\\\ = {a^3} - 3{a^2}b + 3a{b^2} - {b^3}\\\ = {a^3} - 3ab(a - b) - {b^3}\end{array}\]
We can summarize the cube of a binomial with a subtraction sign in between can be expressed as \[{(a - b)^3} = {a^3} - 3{a^2}b + 3a{b^2} - {b^3}\] .
Example: \[{(x - 4)^3}\]
Solution: \[{(x - 4)^3} = {x^3} - 3{x^2}4 + {\kern 1pt} 3x{4^2} - {4^3}\]
\[{(x - 4)^3} = {x^3} - 12{x^2} + 48{x} - {64}\]
Cube of Difference
Without actually computing the cubes, the difference of cubes formula is used to determine the difference of cubes between two numbers. The cube binomials are factorized using the difference of cubes formula. The cube of the difference between two expressions is equal to the cube of the first, minus three times the product of the squares of the first and second, plus three times the product of the first and second squares of the first and second, minus the cube of the second.
Formula:\[{a^3} - {b^3} = (a - b)({a^2} + ab + {b^2})\]
Cube of Difference Examples
The following are examples of the cube of differences i.e.,
Solve it
a) \[({x^3} - 125)\]
Solution:\[ \Rightarrow {x^3} - {5^3} = (x - 5)({x^2} + 5x + 25)\]
b) Factorize the expression\[(8{x^3} - 125)\] by using the difference of cubes formula.
Solution:We can write the given expression as \[8{x^3} - 125 = {(2x)^3} - {5^3}\]
Substituting the values a=2x and b= 5 in the formula of the difference of cube\[ = (2x - 5)({(2x)^2} + (2x) \times 5 + {(5)^2})\]
\[= (2x - 5)(4{x^2} + 10x + 25)\]
The Cube of the Difference of 1 and A Variable
The cube of difference of 1 and a variable can be expressed as \[(1 - {x^3})\] where a=1 and b=x and then we solve it in the same way using the cube of difference formula.
For example:\[\begin{array}{l}(1 - 125{x^3}) = (1){}^3 - {(5x)^3}\\ \Rightarrow (1 - 5x)(1 + 5x + 25{x^2})\end{array}\]
Solved Questions
1.Expand it: \[{(4x - 3y)^3}\]
Ans: \[\begin{array}{l} \Rightarrow {(4x)^3} - 3{(4x)^2}(3y) + 3(4x){(3y)^2} - {(3y)^3}\\ \Rightarrow 64{x^3} - 144{x^2}y + 108x{y^2} - 27{y^3}\end{array}\]
2.Factorise: \[(1 - 64{x^3}{y^3})\]
Here, a=1 and b=4xy
Ans:\[\begin{array}{l} \Rightarrow {(1)^3} - {(4xy)^3}\\ \Rightarrow (1 - 4xy)[({(1)^2} + (1)(4xy) + {(4xy)^2}]\\ \Rightarrow (1 - 4xy)(1 + 4xy + 16{x^2}{y^2})\end{array}\]
3. Use the formula and evaluate (97)3
\[\begin{array}{l}{(97)^3} = {(100 - 3)^3}\\\end{array}\]
we know that \[{(a - b)^3} = {a^3} - 3{a^2}b + 3a{b^2} - {b^3}\]
a=100 and b=3
\[\begin{array}{l} \Rightarrow {(100)^3} - 3{(100)^2}(3) + 3(100){(3)^2} - {(3)^3}\\ \Rightarrow 1000000 - 90000 + 2700 - 27\\ \Rightarrow 912,673\end{array}\]
Therefore,\[{(97)^3} =912,673\]
Summary
The most widely used perfect cubes are those whose roots are integers rather than decimals. To factor the differences between the two perfect cubes, keep in mind that the variance of the cube roots of the two perfect cubes is equal to the product of their cube roots and the sum of their squares Binomial is the name for an algebraic expression with only two terms. It is a polynomial with two terms. It is also referred to as the sum or difference of two or more monomials.
FAQs on Cube of the Difference of Two Binomials
1. How many terms are contained in each binomial cube?
We are aware that the cube of any number "y" is written as \[y \times y \times y\] or \[{y^3}\] which is referred to as a cube number. Consequently, given a binomial, an algebraic expression with two terms which can be a+b or a-b respectively.
2. What is the factor of \[{a^3} - {b^3}\]?
The difference of cube can be factored as \[{a^3} - {b^3} = (a - b)({a^2} + ab + {b^2})\]
3. Expand: \[108 - 4{a^6}{b^{12}}\]
\[\begin{array}{l} \Rightarrow 4(27 - {a^6}{b^{12}})\\ \Rightarrow 4[{(3)^3} - ({a^2}{b^4})^3]\\ \Rightarrow 4(3 - {a^2}{b^4})(9 + 3{a^2}{b^4} + {a^4}{b^8})\end{array}\]











