Factors of 121

As we all know that $121$ is an odd composite number, it has some proper factors i.e., the factors other than $1$ and $121$ itself. $121$ has a total $3$ of factors: $1$, $11$ and $121$. Naturally, a prime factor of $121$. is a factor of $121$ which is also a prime number; hence from the above list of factors, we can see that $11$ is the only prime factor of $121$. Moreover, $121$ is a perfect square i.e., each prime factor of $121$ occurs an even number of times. Here, the only prime factor of $121$ is $11$ and it occurs $2$ times in the prime factorization of $121$ i.e., $121=11\times 11$. So, the square root of $121$ is $11$. Also, the pair factors of $121$ are: $(1,121)$ and $(11,11)$.

Definition of a Factor of an Integer

A factor of an integer is another integer that can divide the integer without leaving any remainder i.e., an integer $F$ is said to be a factor of another integer $N$ if $F$ completely divides $N$. For example, the integers $2$ and $3$ can divide the integer $6$ without leaving any remainder; so, $2$ and $3$ are factors of $6$.

A factor can be both a positive and a negative integer that we will understand by the same example above. As $2$ is a factor of $6$, $-2$ is also a factor of $6$ because $6÷(-2)=-3$ which is also an integer i.e., $(-2)$ completely divides $6$ without leaving any remainder. Similarly, $(-3)$ is also a factor of $6$. Thus, we can say that if $F$ is a factor of $N$, then $-F$ is also a factor of $N$. Here, in this topic, we will mostly talk about the positive factors of $121$.

Proper and Improper Factors

As $1$ divides every integer and each integer divides itself, by definition of a factor, every integer $N$ has at least $2$ factors: $1$ and $N$ itself. These are called the Improper Factors of $N$. All other factors of $N$. are called the Proper Factors of $N$. For example, $1$, $6$ are the improper factors of $6$ and $2$, $3$ are proper factors of $6$.

Prime Factors

As the name suggests, a prime factor of an integer $N$ is an integer $P$ that is a factor of $N$ as well as a prime number. For example, $2$ and $3$ are prime factors of $6$ as they are factors of $6$, and also, they are prime numbers.

Pair Factors

A pair of integers $(F_1,F_2)$ are said to be a Pair Factors of an integer $N$, if firstly, they are factors of $N$ and secondly, their product yields $N$ i.e., $F_1\times F_2=N$. For example, as $1$, $2$, $3$, $6$ are factors of $6$ and $1\times 6=6$, $2\times 3=6$; $(1,6)$ and $(2,3)$ are pair factors of $6$.

What are the Factors of 121

Factors of $121$ are the integers that completely divide it without leaving any remainder. Let us first find out the integers that fully divide $121$:

$121÷1=121$

$121÷121=1$

$121÷11=11$

Hence, by definition, $121$ has a total of $3$ factors: $1$, $11$ and $121$.

If we consider negative factors also, then $121$ has a total of $6$ factors, viz., $\pm 1$, $\pm 11$, and $\pm 121$.

Method of Finding the Factors of 121

In this section, we will learn how to find the factors of $121$. There are several methods of finding the factors of an integer. Here, we will follow the Division Method. In this method, we will divide $121$ successively by the integers starting from $1$, $2$, $3$ and so on and whenever we get the remainder $0$, then the corresponding divisor and quotient will be treated as the factors of $121$. This process continues till any one of the numbers is repeated.

In Step 11, the quotient and the divisor are the same i.e., $11$. This means that all the factors of $121$ have been obtained. So, we must stop the process.

Hence, the factors of $121$ are: $1$, $11$ and $121$.

Moreover, notice that in Step-11, $121=11\times 11$ i.e., the number $11$ repeats itself as a factor. Hence, $121$ is a perfect square.

Here, also, we can use the divisibility rule of integers to check whether $2$, $3$, …, $10$, etc. divide $121$ or not.

Prime Factorisation of 121: Factor Tree

A prime number is a positive integer $P$ that has only two factors (not considering the negative factors here). For example, $2$, $3$, $5$ etc.

One of the most useful methods of finding the factors of an integer is prime factorization. In this method, we factorize an integer only into its prime factors. Let us find the prime factorization of $121$. This can be obtained by the following few steps.

1. In the first step, we take the smallest prime number $2$ and check whether it divides $121$ or not. Obviously, as $121$ is an odd number, $2$ does not divide $121$ completely as it leaves a non-zero remainder $1$ $(121=2\times 60+1)$ while dividing $121$. Therefore, $2$ is not a prime factor of $121$.

2. Next, we proceed to the next prime number i.e., $3$, and check whether it divides $121$ or not. But $3$ does not divide $121$ as it leaves a non-zero remainder $1$ $(121=3\times 40+1)$ while dividing $121$.

3. Proceeding similarly, we get the first prime number that divides $121$ is $11$. Also, $121÷11=11$.

4. In the next step, we take the quotient $11$ obtained in the above step and check whether $11$ divides it or not. Obviously $11$ divides itself and $11÷11=1$.

As we have got $1$ as a quotient at the end, we cannot proceed further because $1$ is not a multiple of any prime numbers.

In brief, we perform the following in the above four steps:

$121÷11=11$

$11÷11=1$

Therefore, $11$ is the only prime factor of $121$. Moreover, the prime factorization of $121$ is $121=11\times 11$. This can be easily understood by the following factor tree of $121$:

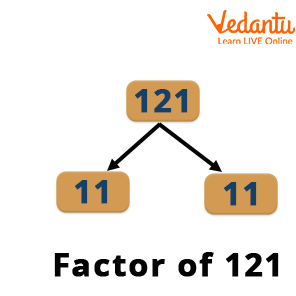
Factor Tree of 121

Now, we can find all the factors of $121$ by prime factorization as follows:

• First, write all the prime factors as many times as they occurred: $11,11$.

• Now, multiply them and get: $11\times 11=121$. Thus, the factor obtained is $121$.

• Also, $1$ is a factor.

• Now, list all the factors. Hence, all the factors of $121$ are: $1$, $11$ and $121$.

Factors of 121 in Pair

A pair factors of $121$ will be a pair of factors $(f_1,f_2)$ of $121$ whose product $f_1{f}_2$ equals to $121$.

We have,

$121=1\times 121$

$121=11\times 11$

Hence, by definition, the pair factors of $121$. are: $(1,121)$ and $(11,11)$.

Interesting Facts

• $121$is a perfect square. The square root of $121$ is $11$.

• The sum of all the factors of $121$ is $133$.

• Did you know that there are no factors of $N$ in between ${\displaystyle \frac{N}{2}}$ and $N$?

• The smallest factor of any number is $1$ and the largest factor is the number itself.

• Prime numbers have exactly two factors: $1$ and the number itself.

• Composite numbers have more than two factors.

• $1$ is neither prime nor composite as it has only one factor i.e., $1$ itself.

Solved Examples

Example 1: Find the sum of all the factors of 121.

Solution: To find the sum of all factors of $121$, we need to find all the factors of $121$ first. As we have discussed before, $121$ has only three factors: $1$, $11$, and $121$. So, the sum of all the factors of $121$ is $s=1+11+121=133$.

Example 2: Find the sum of all the prime factors of 121.

Solution: We know that 11 is the only prime factor of 121

And therefore, the sum of the prime factors is 11.

Conclusion

In this article, we learned the factors of $121$ using methods such as Division Method, Prime Factorization Method, etc. It has a total of 3 factors: $1$, $11$, and $121$ among which, there is only one prime factor which is $11$. Prime factorization of $121$ is $121=11\times 11$. $121$ is a perfect square; the square root of $121$ is $11$. We hope this article helped you understand how to factor $121$. Grab a pen and paper, then try to practice the questions from this article on your own.

Practice Questions

1. Find the smallest and the largest factor of $121$ which is a perfect square.

2. Find H.C.F. of $101$ and $121$.

3. Is $7$ a factor of $121$?

Answer

1. $1$, $121$

2. $1$

3. No.