




An Introduction to Factors of 122
In Mathematics, there are two types of numbers: prime and composite. The numbers with no factor other than 1 and the number itself are known as prime numbers. The numbers with at least one factor other than 1 are known as composite numbers. If a given number, when divided by some number, gives the whole number as a quotient and 0 as a remainder, then the divisor is the factor of the given number.
Factors of a Number
The factor of a given number is a value that divides the number completely, leaving 0 as the remainder. The factors of a number can be positive as well as negative. For example, if 2 and 3 divide 6 completely, then we can say that 2 and 3 are factors of 6.
Factorisation of a Number
Factorisation refers to writing a number as the product of different smaller numbers. The smaller numbers can be either prime numbers or composite numbers. The smaller numbers are referred to as the factor of the given number. For example, 3 and 27 are the factors of 81.
What are the Factors of 122?
Given the number is 122, it is known without a doubt that it will have at least two factors which are 1 and the number itself. So two of the factors are 1 and 122. Now to find the remaining factors of 122, we need to divide 122 by numbers starting from 2 to 121. The numbers which will give 0 as the remainder are the factors of 122. We find out that the numbers satisfying this condition are 2 and 61.
Further, no such number will divide 122 completely with 0 as the remainder. Hence, we get the factors of 122 as 1, 2, 61 and 122. Considering 122 as an integer, we can include both positive and negative numbers as the factors of 122. Thus, all the factors of 122 will be 1, -1, 2, -2, 61, -61, 122 and -122.
Prime Factors of 122
The numbers with 1 and themselves as factors are known as prime numbers. The factors of 122 are 1, 2, 61 and 122. Out of these, 2 and 61 are prime numbers. Thus, the prime factors of 122 are 2 and 61.
The prime factors of a number can also be represented in a factor tree. A factor tree is a diagram in which we find the factors of a number and then further break those numbers into smaller numbers until further factorisation is impossible. In the end, we get the prime factors of the original number.
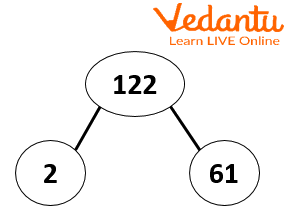
Factors tree of 122
Prime Factorisation of 122
Any composite number can be expressed as the product of primes. This process of representing 122 as a product of primes is known as prime factorisation. For prime factorisation of a number, we start dividing it with the smallest prime number, which will give 0 as the remainder. Next, we divide the quotient obtained with the next prime number to get the remainder as 0. This process is repeated till the quotient obtained is 1.
As we know that 122 is a composite number, so it will have prime numbers as its factors. So to express 122 as the product of primes, first, divide it by the smallest prime number; here, it is 2, such that the remainder is 0. It will give 61 as the quotient, that is, \[122 \div 2 = 61\].
Now, divide the quotient with the next smallest prime number such that the remainder is 0. Here we observe that 61 is itself a prime number. So the next prime number that divides 61 will be 61 itself, giving 1 as a quotient.
Thus, the prime factorisation of 122 is $2 \times 61.$
Factors of 122 in Pairs
The pair of numbers, when multiplied with each other, give 122 as products are the pair factors of 122.
As we know that \[1 \times 122 = 122\]. So, (1, 122) is a pair factor. Also \[2 \times 61 = 122\]. So, (2, 61) is a pair factor.
Considering 122 as an integer, \[\left( { - 1} \right) \times \left( { - 122} \right) = 122\] and \[\left( { - 2} \right) \times \left( { - 61} \right) = 122\]. So, (-1, -122) and (-2, -61) are also pair factors.
Number of Factors of 122
The factors of the number 122 are 1,2,61 and 122. The negative value of the factors is also a factor of 122, which are -1,-2,-61, and -122. The total number of factors of 122 are 8.
Interesting Facts
2 is the only even prime number.
1 is neither prime nor composite.
The prime numbers that are two spaces apart are called twin primes.
Products of two prime numbers are known as semiprime numbers.
Solved Examples
Q1. What is the sum of all the prime factors of 122?
Ans: The prime factor of 122 is \[2 \times 61\]. The Sum of all the prime factors is 2+61=63.
Q2. Find the common prime factors of 122 and 76.
Ans: Prime factorisation of \[122 = 2 \times 61\]
Prime factorisation of \[76 = 2 \times 2 \times 19\]
From above, we can see that the common prime factor of 122 and 76 is 2.
Q3. Which of the following is not a prime factorization?
\[25 = 5 \times 5\]
\[64 = 8 \times 4 \times 2\]
\[120 = {2^3} \times 15\]
Ans: In (a) 25 is written as the product of two 5s. As 5 is a prime number, thus 25 has been expressed as the product of primes.
In (b) 64 is written as the product of 8, 4 and 2. 2 is a prime number, but 8 and 4 are not prime numbers; they can be further expressed as smaller prime numbers as 8 = 23 and 4 = 22. Thus, 64 has not been expressed as the product of primes.
In (c), 120 is written as the product of 23 and 15. 2 is a prime number, but 15 is not a prime number; it can be expressed as \[3 \times 5\]. Thus, 120 has not been expressed as the product of primes.
Practice Questions
Q1. Which of the following is not a prime factorisation?
a. \[27 = 1 \times 27\]
b. \[100 = 2 \times 2 \times 5 \times 5\]
c. \[100 = {2^2} \times {5^2}\]
Ans. a. \[27 = 1 \times 27\]
Q2. What is the sum of all the positive factors of 122?
Ans. 186.
Key Features
Factorisation refers to breaking a given number into smaller numbers such that the product provides the original number.
We can also perform prime factorisation of a number, expressing a number as a product of primes.
The prime factorisation of 122 is \[2 \times 61\].
Given number 122 is a composite number with 1, 2, 61 and 122 as the positive factors and -1, -2, -61 and -122 as negative factors.
List of Related Articles
FAQs on Factors of 122
1. How do you find the prime factorisation of a number?
To find the prime factorisation of a number, firstly divide it with the least prime number, such that the remainder is 0. Then divide the quotient obtained with the next least prime number, such that the remainder is 0. Repeat the process until the quotient becomes 1.
2. What is the difference between factorisation and prime factorisation?
Factorization means breaking the given number into smaller factors. Prime factorisation means breaking the number into smaller numbers until all the factors are prime by nature.
3. What is the use of prime factorisation?
Prime factorisation is useful for finding square roots and calculating the HCF and LCM of different numbers.











