




The Factors of 318 - An Introduction
Factor of a number is an integer that divides the given number evenly. A prime number has at least 4 (including negative factors) factors. A composite number has more than 4 factors. Now, coming to the main topic of our article, What are factors of 318? The answer is, a factor of 318 divides 318 evenly. If a number has multiple which is equal to 318 then the number is a factor of 318.
To answer our question, What are factors of 318? we need to discuss the factor of 318, what we truly mean is all the positive and negative whole numbers that can divide 318. The result of taking the number 318 and dividing it by one of its factors would be another factor of it.
Let's examine how to identify and list each of the 318 elements.
These numbers can be written as individual factors or factor pairs and can divide 318 into equal parts. We present them both ways. This is a specific number's mathematical decomposition.
How to Find the Factors of 318
The factor of 318 can be calculated by finding all divisors of 318 that divide completely. Since 318 is an even number, it is divisible by 2 and 2 is the least prime number.
\[318 \div 2 = 159\]
Thus 2 is a factor of 318.
Now find the least prime factor that divides quotients evenly.
159 is an odd number, thus it is not divisible by 2.
The sum of the digits is 1+5+9 = 15 which is divisible by 3.
Thus 159 is divisible by 3.
\[159 \div 3 =53\]
Thus 3 is a factor of 318.
Now 53 is a prime number, it is only divisible by 53.
\[53\div 53=1\]
Thus 53 is a factor of 318.
The product of any two factors is also a factor of 318.
Thus \[2 \times 3 = 6, 2\times 53 = 106, 3 \times 53 = 159\] are also a factor of 318.
A number is divisible by 1 and 318, thus 1 and 318 are factors of 318.
The factors of 318 are 1,2,3,6,53,106,159, and 318.
Negative Factors:
A negative integer can divide a positive number, but the quotient will be a negative number.
\[318 \div (-1) = -318\]
\[318 \div (-2) = -159\]
\[318 \div (-3) = -106\]
\[318 \div (-6) = -53\]
\[318 \div (-53) = -6\]
\[318 \div (-106) = -3\]
\[318 \div (-159) = -2\]
\[318 \div (-318) = -1\]
Thus -1,-2,-3,-6,-53,-106,-159, and -318 divides 318 evenly. Thus -1, -2, -3, -6, -53, -106, -159, and -318 are the factors of 318.
Prime Factorization:
Prime factorization of a number is a process to find prime factors of a number. To find factors of 318 by prime factorization, First we will divide 318 by the least prime that divides 318 evenly. In the same manner we divide quotients by least prime.
The product of prime numbers is 318. The prime factors of 318 by prime factorisation are 2,3,53.
Factor Tree of 318
Tree diagram is a pictorial representation of prime factorization of a number. It shows all prime factors of 318.
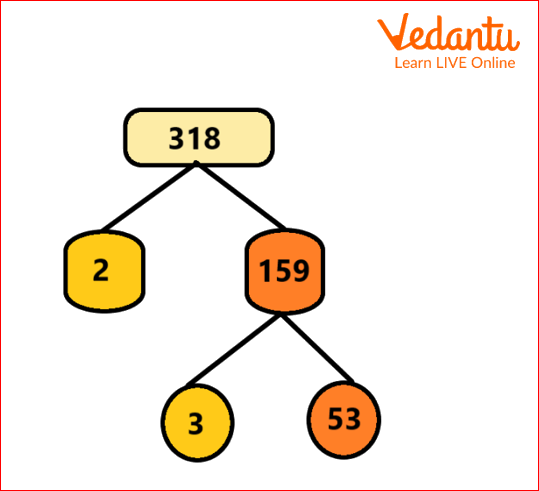
Factor tree of 318
Factor of 318 in Pairs
Factor pair looks like an ordered pair consisting of two elements. The number of factor pairs of numbers is equal to the half number of factors of a number. To find factor pairs, we will arrange the factors ascending and descending order. For the first factor pair:the first element will be the first number of ascending order and the second element will be the first element of descending order. For the second factor pair: the first element will be the second number of ascending order and the second element will be the second element of descending order. In the same manner we will find rest of them
Ascending order: 1,2,3,6,53,106,159,318
Descending order:318,159,106,53,6,3,2,1
Then the factors of 318 in pairs are (1, 318), (2, 159), (3, 106), (6, 53), (53, 6), (105, 3), (159, 2), (318, 1).
For negative factors
Ascending order:-318,-159,-106,-53,-6,-3,-2,-1
Descending order:-1,-2,-3,-6,-53,-106,-159,-318
(-318,-1),(-159,-2),(-105,-3),(-53,-6),(-6,-53),(-3,-106),(-2,-159),(-1,-318).
Order pairs are commutative. This means (a,b) = (b,a).
The order pairs are(1,318),(2,159),(3,106),(6,53), (-318,-1),(-159,-2),(-105,-3)and (-53,-6).
Number of Positive Factors:
The number of factors of a number depends on the exponent of prime factors of the given number. Assume N is a composite number and X, Y, and Z are prime factors of N such that \[N = X^aY^bZ^c\].
The formula of the number of positive factors of N is (a+1)(b+1)(c+1).
The prime factorization of 318 is \[2\times 3\times 53\].
Thus the number of positive factors of 318 is (1+1)(1+1)(1+1) = 8.
Sum of Factors:
The factors of 318 are 1,2,3,6,53,106,159,318,-1,-2,-3,-6,-53,-106,-159,-318.
Thus the sum of factors is 1+2+3+6+53+106+159+318+(-1)+(-2)+(-3)+(-6)+(-53)+(-106)+(-159)+(-318) =0
Solved Examples
Example 1: What is the sum of non prime factors of 318?
Sol: The prime factorization of 318 is
The prime factor of 318 is 2,3,53. The products of any two prime factors are \[2 \times 3 = 6, 2 \times 53 = 106, 3 \times 53 = 159\]. The negative value of the factors is also a factor of the number.
The factors of 318 are 1,2,3,6,53,106,159, 318,-1,-2,-3,-6,-53,-106,-159, -318.
The non prime factors are 6,106,159, 318,-1,-2,-3,-6,-53,-106,-159, -318.
The sum of the non prime factors are
6+106+159+318+(-1)+(-2)+(-3)+(-6)+(-53)+(-106)+(-159)+( -318) = -59.
Example 2: What is the sum of prime factors of 318?
Sol: The prime factorization of 318 is
The prime factor of 318 is 2,3,53. The sum of the prime factors is 2+3+53 = 59
Example 3: What is the highest factor of 318?
Sol: The prime factorization of 318 is
The prime factor of 318 is 2,3,53. The products of any two prime factors are \[2 \times 3 = 6, 2 \times 53 = 106, 3 \times 53 = 159\]. The negative value of the factors is also a factor of the number.
The factors of 318 are 1,2,3,6,53,106,159, 318,-1,-2,-3,-6,-53,-106,-159, -318.
The highest factor of 318 is 318.
Key Features about Number 318 and its Factors
318 is a composite number rather than a prime number.
The composite number 318 has the following prime factors:
\[318 = 2 \times 3 \times 53\]The factors 318, 159, 106, 53, 6, 3, 2, 1 and all the negatives -318, -159, -106, -53, -6, -3, -2, -1 all of these can exactly divide 318.
Conclusion
The factors of 318 are 318, 159, 106, 53, 6, 3, 2, 1,-318, -159, -106, -53, -6, -3, -2, -1. The prime factors of 318 are 2,3,53. As the number of positive factors is 8. Since the number of factors of 318 is more than 2, then 318 is a composite number.
List of Related Articles
Practice on Your Own
Q 1: What is the product of the two highest factors of 318?
Ans: 50562.
Q 2: What are the common factors in 318 and 87?
Ans: 1 and 3.
FAQs on Factors of 318
1: How many methods are there to find factors of a number?
There are two types of methods to find factors of a number: Division method and product method. If a whole number divides a given number evenly, then the divisor is a factor of the given number. If the product of two whole numbers is a given number, then the whole numbers are the factors of the given number.
2: What are the differences between multiples and factors?
The multiples of a number are the numbers that are produced by the given number with a positive integer. The factors of a given number are a number that are divisible by a given number evenly.
Suppose a given number is 12.
2 | 12 |
2 | 6 |
3 | 3 |
1 |
The factors of 12 are 1, 2, 3, \[2\times 2 = 4\], \[2\times 3 = 6\], \[2\times 2 \times 3 = 12\].
\[12\times 1 = 12\]
\[12 \times 2 = 24\]
\[12 \times 3 = 36\]
The multiples of 12 are 12,24, 36.
The number of multiples is infinite and the number of factors of a number is finite.
3: Why is 2 a prime number although it is an even number?
A prime number is a whole number which is divisible by 1 and itself. 2 is also divisible by 1 and 2. Thus 2 has only two factors. Thus 2 is the prime number.











