How to Calculate Focal Distance of an Ellipse with Step-by-Step Solutions
The focal distance of an ellipse denotes, basically, the distance of a point on the circumference of the ellipse from any of its foci. Thus, every point on the ellipse displays two focal distances as measured concerning the ellipse's two foci. The sum of the focal distances of any point on an ellipse is constant by the definition of the ellipse. The sum of the focal distances of any point on an ellipse bears a distinct relation to the length of the major axis of the ellipse.
What is the General Equation of an Ellipse?
An ellipse is a conic section with its centre midway between its two foci lying on the major axis. If the centre of an ellipse lies at the coordinate $(h,k)$ and its major and minor axes of the ellipse are parallel to the coordinate axes in the XY plane having the lengths 2a and 2b, respectively, then the equation of the ellipse is given in the following way.
$ \dfrac{(x-h)^{2}}{a^{2}} + \dfrac{(y-k)^{2}}{b^{2}} =1$
What is the Focus of an Ellipse?
The locus or the path of points for which the ratio of distances from a fixed point and the defined straight line is less than 1 is called an ellipse. The fixed point and the straight line are the focus and the directrix of the ellipse, respectively. An ellipse has two points of reference called the focus of the ellipse. These are called foci of the ellipse.
What are the Axes of an Ellipse?
An ellipse is the locus of all points for which the sum of its distances from the two fixed points (called foci) is a constant.
An ellipse has two axes of symmetry.
The two points most far from the centre of the ellipse are called the vertices.
The two points at least a distance from the ellipse's centre are called the co-vertices.
The major axis is the line segment passing through the centre, joining the vertices.
The minor axis is the line segment passing through the centre, joining the co-vertices.
The line segments joining the centre to each vertex are called the semi-major axes.
The line segments joining the centre to each co-vertex are called the semi-minor axes.
How is the Focal Distance Calculated in an Ellipse?
To calculate the focal distances of any point on an ellipse, we proceed in the following steps.
Let P(x,y) be a point on ellipse $\dfrac{x^{2}}{a^{2}} + \dfrac{y^{2}}{b^{2}} =1$.
The directrices of the ellipse are ZK, and Z'K' and its foci are S and S’.
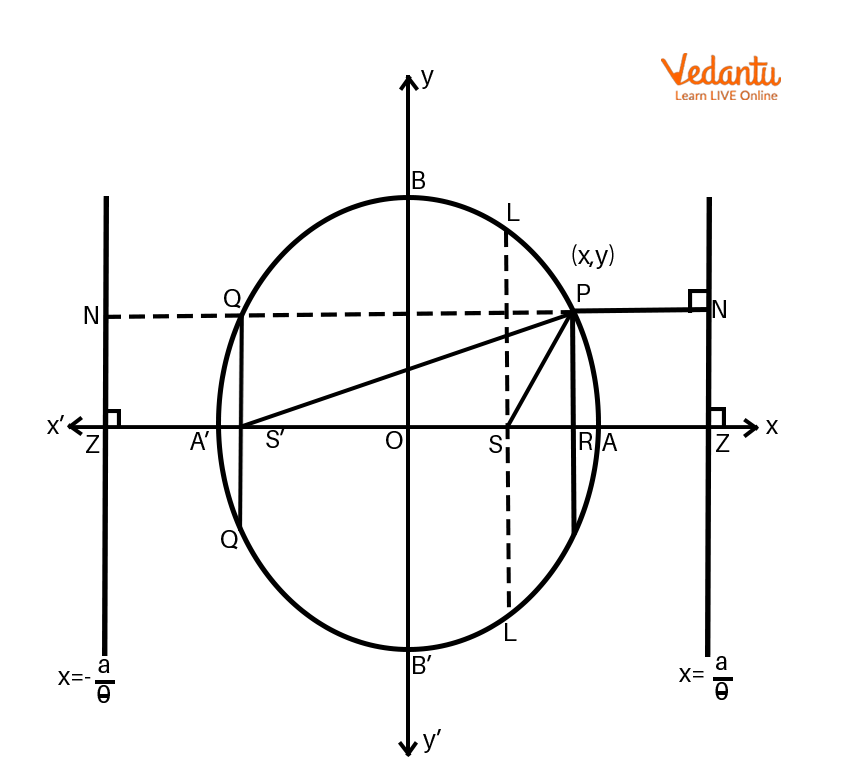
Focal Distance of Ellipse
Point P is connected to the foci S and S’ with the straight lines PS and PS’.
Let us draw NPN' as the perpendicular through the point P on directrices ZK and Z'K'.
Now by definition of the eccentricity of an ellipse, we get,
SP = e.PN
SP = e.RZ
SP = e (OZ - OR)
SP = e(a/e - x)
SP = a - ex ………… (1)
And
S'P = e ∙ PN'
S'P = e ∙ (RZ')
S'P = e (OZ' + OR)
S'P = e (x + a/e)
S'P = a + ex ………. (2)
Calculating the value of SP + S'P = a - ex + a + ex = 2a = major axis.
Hence, it is proved that the sum of the focal distances of any point on an ellipse is constant and always equal to the length of the ellipse's major axis.
What is the Focal Chord of an Ellipse?
A focal chord is a chord that passes through the focus of an ellipse. A focal chord is an essential tool to solve many problems in geometry. We can determine the major and the minor axes in an ellipse with the help of the length of the focal chord. The ellipse's focal width is the focal chord's length perpendicular to the major axis and passes through one of the foci with the endpoints on the ellipse.
Length of the Focal Chord of the Ellipse
To calculate the length of the focal chord of an ellipse, we proceed with the following steps. The foci of an ellipse are S and S’.
Length of the Focal Chord of the Ellipse
Let us suppose that the focal chord PQ is inclined to the major axis at angles t and u at the foci S and S’, respectively, where PS=r. The coordinate of the point P is p(t).
By the geometry of the figure, we get that $p(t) = [ae+ rcos(t) , rsin(t)]$
$\dfrac{\left (ae+rcos(t) \right )^{2}}{a^{2}}+\dfrac{\left ( rsint \right )^{2}}{b^{2}} =1$
$ b^{2}a^{2}e^{2}+b^{2}r^{2}cos^{2}t + 2ab^{2}ercos(t) + a^{2}r^{2}sin^{2}t-b^{2}a^{2}=0$
Simplifying we get the following.
$(a^{2}sin^{2}t + b^{2}cos^{2}t)r^{2} +2ab^{2}ecos(t)-b^{4} =0$ (as we know $b^{2}=a^{2}(1-e^{2})$)
Solving this equation, we get the two roots
$r_{1}=l_{1}, r_{2}=-l_{2} $
$ \left | l_{1} + l_{2}\right |=\left | r_{1}-r_{2} \right | =\sqrt{\left ( r_{1}+r_{2} \right )^{2}-4r_{1}r_{2}}$
$\left | l_{1}+l_{2} \right |=\sqrt{\left ( \dfrac{2abecos(t)}{a^{2}sin^{2}t + b^{2}cos^{2}t} \right )^{2}+ \dfrac{4b^{4}}{a^{2}sin^{2}t + b^{2}cos^{2}t}}$
Simplifying the above expression, we get the following.
$ \left | l_{1}+l_{2} \right |= \dfrac{2b^{2}}{a^{2}sin^{2}t +b^{2}cos^{2}t} \sqrt{a^{2}e^{2}cos^{2}t + a^{2}sin^{2}t + b^{2}cos^{2}t}$
Now substituting $b^{2}=a^{2}(1-e^{2})$ , we get the following.
$\left | l_{1}+l_{2} \right |=\dfrac{2b^{2}}{a^{2}sin^{2}t +b^{2}cos^{2}t} \sqrt{a^{2}e^{2}cos^{2}t + a^{2}sin^{2}t + a^{2}cos^{2}t - a^{2}e^{2}cos^{2}t }$
So we get length of focal chord PQ $= \dfrac{2ab^{2}}{a^{2}sin^{2}t +b^{2}cos^{2}t }$
Hence, the length of the focal chord of the ellipse $ \dfrac{x^{2}}{a^{2}} + \dfrac{y^{2}}{b^{2}} =1$ which is inclined to the major axis at a given angle θ is $\dfrac{2ab^{2}}{a^{2}sin^{2}\theta +b^{2}cos^{2}\theta }$.
Interesting Facts
The eccentricity of the ellipse will always be between 0 to 1.
An ellipse is created by a plane intersecting a double-napped right cone at some angle of its base.
The ratio of the focal length to the length of the semi-major axis of the ellipse is defined as the ellipse's eccentricity.
Solved Examples
1. Find the sum of the focal distances of a point on the ellipse $\dfrac{x^{2}}{4} + \dfrac{y^{2}}{16} =1$.
Ans: It is evident that the sum of focal distances from the point of an ellipse is 2a, where a is the length of the major axis.
For the given ellipse equation, the semi-major axis length is 4 units.
So, the sum of the focal distances of a point on the ellipse will be $= 2 \times 4 = 8 units$.
2. Find the sum of the distances of any point on the ellipse $9x^{2} + 4y^{2} =36$ from its foci.
Ans: As we know, the sum of the distances to any point on the ellipse(x,y) from the foci is equal to twice the length of the major axis.
$9x^{2} + 4y^{2} =36$
$ \dfrac{x^{2}}{4} + \dfrac{y^{2}}{9} =1 $
Comparing with the general equation of an ellipse
$ \dfrac{x^{2}}{b^{2}} + \dfrac{y^{2}}{a^{2}} =1$ with the given equation
$ \dfrac{x^{2}}{4} + \dfrac{y^{2}}{9} =1 $,
we get the length of the major axis a = 3.
Therefore, the sum of the distances of any point on the ellipse is equal to the length of the major axis $= 2 \times 3 = 6$.
3. Let P be the point on the ellipse having (3,4) and (3,−2) as the ends of the minor axis. If the sum of the focal distances of P is given equally to 10 then find the equation of an ellipse.
Ans: Endpoints of minor axis P=(3,4) and Q=(3,−2)
The Centre of an ellipse is the midpoint of PQ=(3,1)
Length PQ
$=2b=\sqrt{(-2-4)^{2}}=6$
$b=3$
Sum of the focal distance of =2a=10
$a=5$
Therefore equation of ellipse is $\dfrac{(x-3)^{2}}{25} + \dfrac{(y-1)^{2}}{9} =1$
4. What will be the sum of the distances of any point on the ellipse $\dfrac{x^{2}}{16} + \dfrac{y^{2}}{25} =1$ from its foci?
Ans: As we know, the Sum of the distances to any point on the ellipse(x,y) from the foci is twice the length of the major axis.
$\dfrac{x^{2}}{16} + \dfrac{y^{2}}{25} =1$
Comparing with the general equation of an ellipse
$\dfrac{x^{2}}{a^{2}} + \dfrac{y^{2}}{b^{2}} =1$
$\dfrac{x^{2}}{16} + \dfrac{y^{2}}{25} =1 $
We get the length of the major axis, b = 5.
Therefore the sum of the distances of any point on the ellipse = 2 \times 5 = 10.
5. Which is the equation of the ellipse with its axes equal to 5cm and 2cm, respectively?
Ans. The equation of the ellipse with its axes equal to 5cm and 2cm, respectively, is the following.
$\dfrac{x^{2}}{25} + \dfrac{y^{2}}{4} =1$
Summary
There are two axes of symmetry for the ellipse.
The foci of an ellipse always lie on the ellipse's major axis.
The focal chord passes through any focus of the ellipse.
The sum of the focal distance of any point on an ellipse is constant and equal to the length of the ellipse's major axis.
Practice Problems
1. Which is the equation of the ellipse with one of its axes twice the other?
2. Find the equation of the ellipse with its axes equal to each other.
3. Find the length of the axes from the equation $\dfrac{x^{2}}{25} + \dfrac{y^{2}}{4} =1$.
FAQs on Focal Distance of Ellipse: Definition, Formula & Examples
1. How to find focal distance in an ellipse?
To find the focal distance in an ellipse, use the formula $c = \sqrt{a^2 - b^2}$, where $a$ is the semi-major axis and $b$ is the semi-minor axis. This gives the distance between the center and each focus of the ellipse.
2. How to find the focal length of an ellipse?
The focal length of an ellipse is the distance from the center to a focus. It’s calculated by $c = \sqrt{a^2 - b^2}$, where $a$ is the length of the semi-major axis and $b$ is the length of the semi-minor axis.
3. What is the distance between the focus of an ellipse?
In an ellipse, the distance between the two foci is $2c$, where $c = \sqrt{a^2 - b^2}$. So, measure the focal distance from the center to a focus, then double it for the total distance between foci.
4. What is the product of the focal distance of an ellipse?
The product of the focal distances from any point on the ellipse to its two foci is always equal to the length of the major axis ($2a$). For points on the ellipse: $PF_1 + PF_2 = 2a$, where $PF_1$ and $PF_2$ are distances to each focus.
5. What do the terms semi-major and semi-minor axis mean in an ellipse?
In an ellipse, the semi-major axis ($a$) is the longest radius, stretching from the center to the ellipse's edge, while the semi-minor axis ($b$) is the shortest radius. These axes are essential for understanding focal distance.
6. How does focal distance relate to eccentricity in an ellipse?
The eccentricity ($e$) of an ellipse describes how stretched it is. It is calculated using $e = \frac{c}{a}$, where $c$ is the focal distance and $a$ is the semi-major axis. Higher eccentricity means foci are further apart.
7. Why are there two foci in an ellipse rather than one?
An ellipse has two foci because its shape is defined so that for any point on it, the sum of distances to each focus remains constant. This unique property distinguishes ellipses from circles, which have a single center point.
8. Can the focal distance of an ellipse ever be zero?
The focal distance becomes zero only when the ellipse is actually a circle, meaning $a = b$. In this case, both foci coincide at the center, and the eccentricity is also zero, indicating no elongation.
9. How do you measure the focal distance in a real-world ellipse?
To measure the focal distance in a real-world ellipse, first measure the longest diameter (major axis, $2a$) and shortest diameter (minor axis, $2b$). Use $c = \sqrt{a^2 - b^2}$ for the focal distance from the center to each focus.
10. Is the focal distance always less than the semi-major axis of an ellipse?
Yes, the focal distance ($c$) is always less than the semi-major axis ($a$) in an ellipse, since $c = \sqrt{a^2 - b^2}$. This means the foci are always located inside the ellipse, never beyond its longest radius.
