Limits of Trigonometric Functions Using Sandwich Theorem

We will discuss a crucial idea utilised in calculus and its limits in this topic. We'll talk about the Sandwich theorem notion. This theorem is very easy to comprehend and has numerous calculus applications. It is particularly useful for applying trigonometric functions. A thorough comprehension of the sandwich theorem will be useful not only for calculus but also for other ideas like the binomial theorem.

History of Carl Friedrich Gauss

In an effort to compute π, the mathematicians Archimedes and Eudoxus utilised the Sandwich theorem for the first time geometrically. Carl Friedrich Gauss then expressed it in more contemporary terms. Johann Carl Friedrich Gauss was a German mathematician and physicist who made a significant impact on a variety of scientific and mathematical subjects.

Carl Friedrich Gauss (30 April 1777 – 23 February 1855)

What is the Sandwich Theorem in Limits?

Let f, g, and h be real functions such that for any x in the shared definition domain, f(x) g(x) h(x) occurs. When \[a\] is a real number, then

\[\begin{array}{l}\mathop {{\rm{\;}}\lim }\limits_{x \to a} f\left( x \right)\;\; = \;\mathop {lim}\limits_{x \to a} h\left( x \right)\; = \;t\\{\rm{then}}\;\mathop {\lim }\limits_{x \to a} g\left( x \right)\; = \;t\end{array}\]

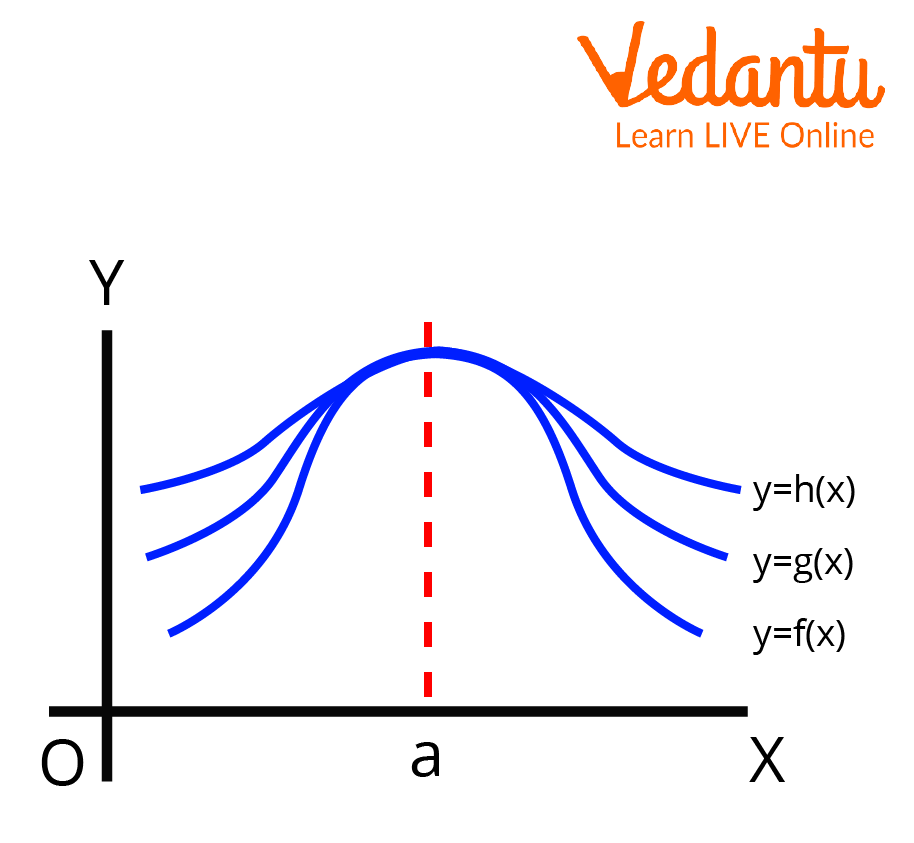
Sandwich Theorem in Limits

Sandwich Theorem Proof

Let's examine the geometric proof for the aforementioned claim using a trigonometric inequality.

Think of the disparity as

\[\cos x\; < \;\sin x\;/\;x\; < 1\]

Sandwich Theorem Proof

We can see the triangles ΔABE, ΔADF, ΔADB, and sector ADB in the above diagram.

\[\begin{array}{l}Area\;\left( {\Delta ABD} \right)\; < \;Area\;\left( {sector\;ADB} \right)\; < \;Area\;\left( {\Delta ADF} \right)\\\; \Rightarrow 1/2.AD.EB\; < \;x/2\pi .\pi .A{D^2}\; < \;1/2.AD.DF\end{array}\]

By eliminating the common terms from all sides,

\[{\rm{EB}} < x.AD < {\rm{ DF}}\]

Since angle A = angle X,

\[EB\; = \;AB\; \sin x\] (from Δ ABE),

and \[\sin A\; = \;EB/AB\].

\[DF\; = \;AD \tan X\] because \[\tan A\; = \;DF/AD\].

However, \[\tan A\; = \;\sin X/\cos x\;\] and \[AB\; = \;AD\].

Consequently,

\[\begin{array}{l}AD.\sin A\; < x.AD < \;AD.\sin A\;/\;\cos A\\ \Rightarrow \; = \;1\; < x\;/\;\sin X < \;1\;/\;\cos X\end{array}\]

By taking reciprocals,

\[\cos x\; < \;\sin x\;/\;x\; < 1\]

Hence proved.

We can simply demonstrate some other trigonometric identities using the above theory, including

\[\begin{array}{l}li{m_{x - > 0}}\sin x/x\; = 1\;\\li{m_{x - > 0}}\;\left( {1\;--\;\cos x} \right)/x\; = 0\end{array}\]

Limitations of Sandwich Theorem

• The Sandwich theorem is not applicable if left and right function limits are not equal.

• The Sandwich Theorem does not apply if we know the limits of any of two functions other than both extreme functions.

Applications of Sandwich Theorem

• To determine the limits of specific trigonometric functions, we can utilise the Sandwich rule.

• It can be applied to link certain sequences between other known sequences that also converge to the same place in order to demonstrate the convergence of those sequences.

Interesting Fact

If \[f\left( x \right) \le g\left( x \right)\] when x is close to \[\alpha \] (unless perhaps at an \[\alpha \]), then $\underset{x\to \alpha }{\mathop{\lim }}\,f\left( x \right)~\underset{x\to \alpha }{\mathop{\lim }}\,g\left( x \right)$.

Solved Examples of Limits of Trigonometric Functions Using Sandwich Theorem

1. Evaluate the limit for the following tangent function \[lim{_{\;x - > 0}}\;\tan x\;/\;x\].

Ans: Using the trigonometric identity,

\[\begin{array}{l}\tan x\; = \dfrac{{\;\sin x}}{{\cos x}}\\\therefore \mathop {\lim }\limits_{x \to 0} \;\dfrac{{\sin x}}{{x\cos x}}\; = \;\mathop {\lim }\limits_{x \to 0} \;\dfrac{{\sin x}}{x}\cdot\mathop {\lim }\limits_{x \to 0} \;\dfrac{1}{{\cos x}}\end{array}\]

And from the Sandwich theorem, we know,

\[\begin{array}{l}li{m_{x - > 0}}\sin x/x\; = 1\\\;li{m_{x - > 0}}\;1/\cos x\; =1\end{array}\]

Therefore,

\[1\cdot\,1\; = \;1\]

2. Prove \[\mathop {\lim }\limits_{x \to 0} \sin x\; = \;0\].

Ans. As known,

$-x\leqslant\sin x\leqslant x\, for \, all\,x \geqslant 0$

\[\begin{array}{l} \Rightarrow \mathop {\lim }\limits_{x \to 0} \left( { - x} \right)\; = \;0\;\;{\rm{and}}\\\mathop {\lim }\limits_{x \to 0} \left( x \right)\; = \;0\end{array}\]

By Sandwich Theorem,

\[\mathop {\lim }\limits_{x \to 0} \left( {Sinx} \right)\; = \;0\]

3. Evaluate \[\mathop {\lim }\limits_{x \to 0} x\dfrac{{secx - 1}}{x}\]

Ans. As we know,

\[\sec x\; = \dfrac{{\;1}}{x}\]

So,

\[\begin{array}{l}\mathop {\lim }\limits_{x \to 0} x\dfrac{{secx - 1}}{x} = \mathop {\lim }\limits_{x \to 0} x\dfrac{{\dfrac{1}{{\cos x}} - 1}}{x}\\\mathop {\lim }\limits_{x \to 0} x\dfrac{{1\; - \cos x}}{{x\cos x}}\\\mathop { \Rightarrow \lim }\limits_{\;\;\;\;\;\;x \to 0} x\left( {\dfrac{1}{{\cos x}}} \right)\left( {\dfrac{{1\; - \cos x}}{x}} \right)\\ \Rightarrow \mathop {\lim }\limits_{x \to 0} x\left( {\dfrac{1}{{\cos x}}} \right)\mathop {\lim }\limits_{x \to 0} x\left( {\dfrac{{1\; - \cos x}}{x}} \right)\\ \Rightarrow 1 \times 0\\ \Rightarrow 0\end{array}\]

Conclusion

In this article, we have thoroughly discussed the Sandwich theorem and its proof. From the discussion above, about the Sandwich theorem we can conclude that the sandwich theorem allows us to determine the limit of a single function by using the limits of the two other functions that it is "sandwiched" between as a starting point. When a function gets complex or intricate, or perhaps when we are unable to identify the limit using other techniques, we apply the Sandwich theorem to determine its limit. The limits of trigonometric functions are most frequently found using this method.

Important Points

• This theorem is probably utilised to establish the limit of the function in question.

• All the functions considered must be real.

Important Formula From the Theorem

According to the Sandwich theorem,

If \[\begin{array}{l}\mathop {{\rm{\;}}\lim }\limits_{x \to a} f\left( x \right)\;\; = \;\mathop {lim}\limits_{x \to a} h\left( x \right)\; = \;t\\{\rm{then}}\;\mathop {\lim }\limits_{x \to a} g\left( x \right)\; = \;t\end{array}\]