How to Solve Polynomial Division Using Long Division Method with Steps and Examples
A generalised version of the well-known arithmetic operation known as long division, polynomial long division is an algorithm for dividing a polynomial by another polynomial of the same or lower degree in algebra. The long division method of polynomials is one of the most common methods of dividing polynomials. In this method, there is a divisor, a dividend (which is to be divided), a quotient, and a reminder. This is a very interesting method of dividing polynomials. The image given below describes the different terms used in the long division method of polynomials.
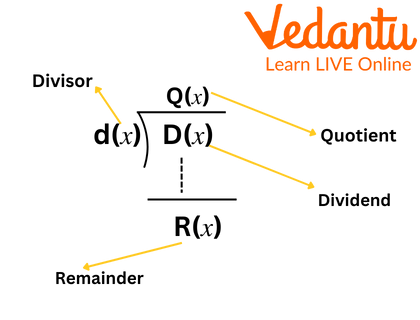
Polynomial Division
What is a Polynomial?
When exponents, constants and variables are combined using mathematical operations like addition, subtraction, multiplication and division, the result is a polynomial. The kind of the polynomial expression may be monomial, binomial or trinomial, depending on how many terms are included in it.
Example: \[9x,2x + 3y,3x + 4z - 9z + 5xyz\] and so on.
Monomial, Binomial and Trinomial
The number of terms in a polynomial determines which of three different sorts of polynomials it is. There are three different kinds of polynomials:
Monomial
Binomial
Trinomial
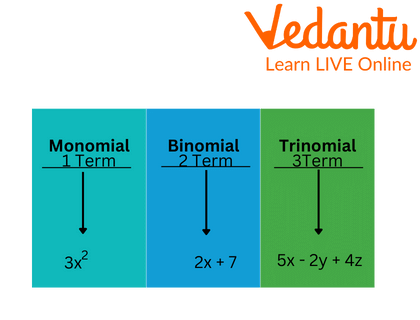
Types of Polynomials
Monomial
Monomial is an expression that contains only one member. There must be only one term which is nonzero. Here are some examples of monomials:
\[3x,5y\].
Binomial
A binomial expression is a polynomial expression containing exactly two terms. A binomial can be thought of as the sum or difference of two or more monomials. Here are some examples of binomials:
\[3x + 4y,6a + 5b\].
Trinomial
A trinomial is an expression with exactly three terms. Here are some examples of trinomial:
\[2{x^2} + 3x + 4\]
Long Division Method of Polynomial
Polynomial Division is the process of dividing one polynomial into another. You can perform division between different types of polynomials. Between two monomials, between a polynomial and a monomial, or between two polynomials. A polynomial is an n-algebraic expression with expressive variables, terms and coefficients.
Steps of Long Division Method of Polynomial
This method of Long Division Polynomials is as follows:
Sort the terms in descending order of degree.
Write the missing terms using 0 as the coefficient.
For the first term of the quotient, divide the first term of the dividend by the first term of the divisor.
Multiply this quotient by the divisor to get the product.
Subtract this product from the dividend and omit the next section (if any).
The difference and the shortened period form a new dividend.
Follow this process until you get the rest. The remainder can be zero or have an index less than the divisor.
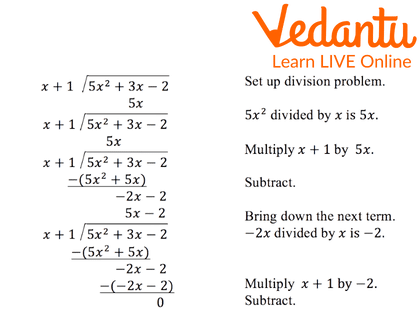
Long Division of Polynomials
Solved Examples on Division Polynomials
1. Solve \[\left( {24{x^2} + 2x + 4} \right) \div \left( {2x + 1} \right)\] using a long division method.
Ans: The image below will give a better understanding on how to divide Polynomials.
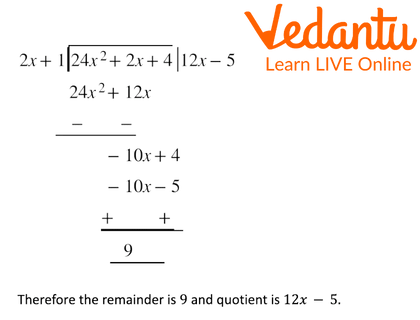
Answer: Remainder: 9
Quotient: 12x - 5
2. How are Polynomials Divided?
Ans: In mathematics, there are two ways to divide polynomials. These are the synthetic approach and lengthy division. The long division method is the most challenging and time-consuming to master, as its name suggests. The synthetic technique, on the other hand, is a "fun" way to divide polynomials.
FAQs on Long Division Method of Polynomials Explained Clearly
1. What is the long division method of polynomials?
The long division method of polynomials is a step-by-step process used to divide one polynomial by another polynomial of equal or lower degree. It is similar to numerical long division and helps find the quotient and remainder.
- Arrange both polynomials in descending powers.
- Divide the leading term of the dividend by the leading term of the divisor.
- Multiply the entire divisor by this result.
- Subtract and bring down the next term.
- Repeat until the degree of the remainder is less than the degree of the divisor.
2. How do you do long division of polynomials step by step?
To perform polynomial long division, divide the leading terms, multiply, subtract, and repeat until the remainder’s degree is smaller than the divisor’s degree.
- Step 1: Arrange terms in descending order of powers.
- Step 2: Divide the first term of the dividend by the first term of the divisor.
- Step 3: Multiply the divisor by this result.
- Step 4: Subtract and simplify.
- Step 5: Bring down the next term and repeat.
- 2x² ÷ x = 2x
- After repeating steps, the final result is 2x + 1 with remainder 0.
3. What is the formula for polynomial long division?
The general formula for polynomial long division is Dividend = (Divisor × Quotient) + Remainder.
- If P(x) is the dividend and D(x) is the divisor, then:
P(x) = D(x) × Q(x) + R(x)
- Here, Q(x) is the quotient.
- R(x) is the remainder.
- The degree of R(x) is less than the degree of D(x).
4. Can you give an example of long division of polynomials?
Yes, an example of long division of polynomials is dividing (x³ − 2x² + 4) by (x − 1).
- x³ ÷ x = x²
- Subtract to get −x²
- −x² ÷ x = −x
- Continue the process
5. What is the difference between synthetic division and long division of polynomials?
The main difference is that synthetic division is a shortcut method used only when dividing by a linear binomial of the form (x − a), while long division works for all types of polynomial divisors.
- Synthetic division is faster and uses coefficients only.
- Long division shows all algebraic steps.
- Long division works for divisors like x² + 1, while synthetic division does not.
6. What happens if the remainder is zero in polynomial long division?
If the remainder is zero, it means the divisor is an exact factor of the dividend polynomial.
- The division is exact.
- The divisor completely divides the polynomial.
- The polynomial can be written as a product of the divisor and quotient.
7. Why do we arrange polynomials in descending order before long division?
Polynomials are arranged in descending order of powers to ensure correct alignment of like terms during division.
- It helps divide leading terms correctly.
- Prevents calculation errors.
- Ensures proper subtraction of matching powers.
8. Can you divide a polynomial by a quadratic using long division?
Yes, you can divide a polynomial by a quadratic polynomial using the long division method.
- The divisor can be linear, quadratic, or higher degree.
- The process remains the same: divide leading terms, multiply, subtract, repeat.
9. What are common mistakes in long division of polynomials?
Common mistakes in polynomial long division include sign errors and incorrect subtraction.
- Not arranging terms in descending order.
- Forgetting to distribute the divisor fully.
- Making errors while subtracting polynomials.
- Ignoring missing terms with zero coefficients.
10. How is long division of polynomials used in algebra?
The long division method of polynomials is used in algebra to simplify rational expressions, factor polynomials, and solve equations.
- Helps rewrite improper rational expressions.
- Used in proving the Remainder Theorem and Factor Theorem.
- Assists in graphing polynomial functions.
