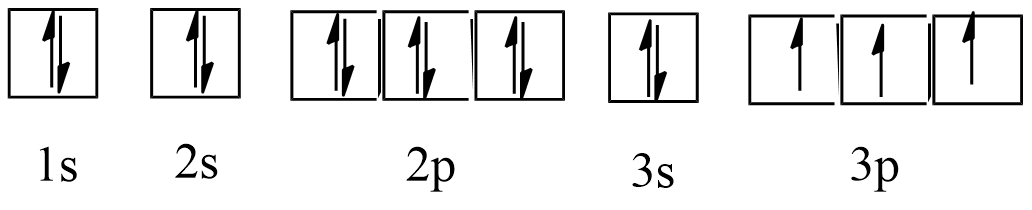Class 11 Chemistry Chapter 2 Structure of Atom NCERT Solutions FREE PDF Download






FAQs on NCERT Solutions for Class 11 Chemistry Chapter 2 Structure of Atom
1. What are the important topics of Structure of Atoms?
The topics covered are
1. Subatomic Particles
Discovery Of Electron
Charge To Mass Ratio Of Electron
Charge On The Electron
Discovery Of Protons And Neutrons
2. Atomic Models
Thomson Model Of Atom
Rutherford’s Nuclear Model Of Atom
Atomic Number And Mass Number
Isobars And Isotopes
Drawbacks Of Rutherford Model
3. Developments Leading To The Bohr’s Model Of Atom
Wave Nature Of Electromagnetic Radiation
Particle Nature Of Electromagnetic Radiation: Planck’s Quantum Theory
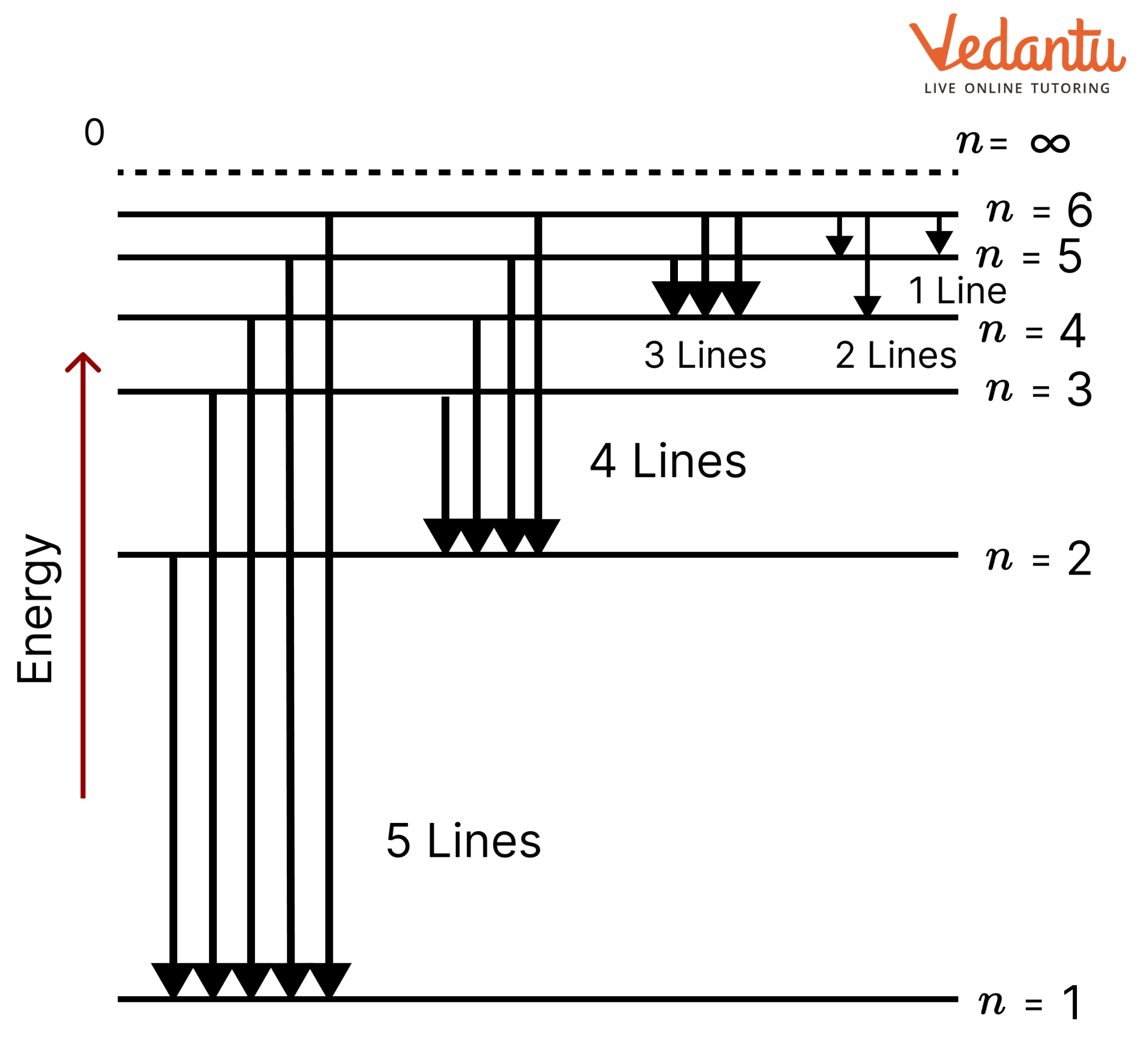
The Quantized* Electronic Energy Levels: Atomic Spectra
4. Bohr’s Model For Hydrogen Atom
Explanation Of Line Spectrum Of Hydrogen
Limitations Of Bohr’s Model
5. Towards Quantum Mechanical Model Of The Atom
Dual Behaviour Of Matter
Heisenberg’s Uncertainty Principle
6. Quantum Mechanical Model Of Atom
Orbitals And Quantum Numbers
Shapes Of Atomic Orbitals
Energies Of Orbitals
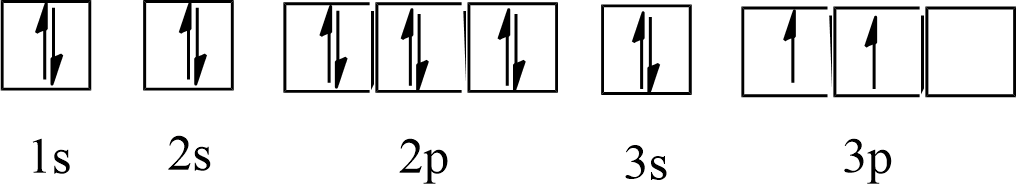
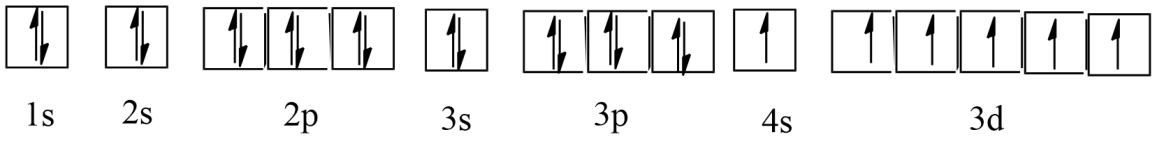
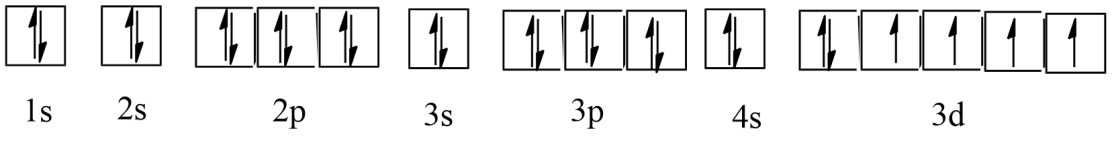
Filling Of Orbitals In Atom
Electronic Configuration Of Atoms
Stability Of Completely Filled And Half Filled Subshells.
2. What is discussed in the Chapter -2 Structure of Atom class 11 NCERT Solutions?
ch 2 chemistry class 11 NCERT Solutuions introduces the concept of atoms, electrons, protons, and neutrons. Also, the students will understand the concepts of isotopes, atomic number, and isobars. These concepts are important for them to understand, as these will build a strong foundation of Chemistry. This chapter will also introduce the students to advanced theories like Rutherford's model, Thomson's model and Bohr's model, their uses and their limitations.
3: What is the photoelectric effect in simple words?
The photoelectric effect is a physical phenomena. The outcome will be based on the notion that electromagnetic radiation is made up of photons, a type of subatomic particle. When an electron collides with a photon on a metal surface, electron emission can be detected. The electrons that are emitted are known as photoelectrons.
The "photoelectric effect" describes how light causes electron emission or ejection from a surface, most commonly a metal. Understanding the photoelectric effect may help one understand the quantum nature of electrons and light, as well as how the concept of wave-particle duality is generated.
4. How to prepare for Chemistry with Vedantu’s Class 11 Chapter -2 NCERT Solutions?
Preparing in a step by step and structured manner will help in understanding the concepts in Chemistry easier. The most scoring and difficult concept in this subject is the Structure of the Atom. The weightage of this topic is also high. You may think of Qualitatives analysis towards the end, as it needs very less time.
Our Class 11 Chapter -2 NCERT Solutions gives you an in-depth knowledge of the conceptual topics. NCERT Solutions have been drafted as per the latest CBSE Class 11 Science Syllabus. Students will feel that the solutions are in a simple language and can understand the difficult topics easily.
5. What type of questions is present in NCERT Solutions for class 11 chemistry chapter 2 exercise solutions?
Class 11 chemistry chapter 2 exercise solutions: Structure of Atoms, you will learn about the existence of atoms, fundamental theories about atoms, their characteristics, features of the quantum mechanical model of atoms, the nature of electromagnetic radiations, Planck's quantum theory, describing the photoelectric effect, features of atomic spectra, and much more. You will be asked questions on the topics covered in the chapter.
6. Why should I opt for NCERT Solutions for Class 11 Chemistry Chapter 2?
Chemistry is a scoring subject where if you understand the concept and the type of questions you can come across during the exam, you can easily answer. Having deep knowledge of the subject is very important. It is equally important to format your answers to score good marks. NCERT Solutions for Class 11 Chemistry Chapter 2 will give you well-structured solutions for each question. You will get a proper idea of how to prepare your responses for better marks. class 11 chemistry chapter 2 exercise solutions pdf are available on the Vedantu Mobile app hence accessible anywhere and anytime.
7. What topics are covered in NCERT Solutions for Class 11 Chemistry Chapter 2?
The topics covered in NCERT Solutions for Class 11 Chemistry Chapter 2 Structure of Atoms are Discovery of Subatomic Particles, Electron, Protons and Neutrons, Charge to Mass Ratio on Electrons, Atomic Models, Atomic Number and Mass Number, Wave Nature of Electromagnetic Radiation, Photoelectric Effect, Emission and Absorption Spectra and much more.
8. What is the importance of understanding NCERT Class 11 Chemistry Chapter 2?
Structure of Atom class 11 NCERT solutions is the most scoring subject if understood properly. Chapter 2, Structure of Atoms is the base of Chemistry, where you will learn and understand about the smallest unit of any substance. Hence, it is very important to understand the chapter and practice thoroughly. You can practice various types of questions to understand every tangent of the chapter.
9. Where can I find accurate solutions for NCERT Class 11 Chemistry Chapter 2?
You can find the most accurate and reliable solutions for NCERT Class 11 Chemistry Chapter 2 by following these steps:
Click on the link NCERT Solutions for Class 11 Chemistry Chapter 2 Structure of Atom The Solutions page of Vedantu for Class 11 Chemistry Chapter 2 Structure of Atoms will open.
At the top of the page, you will find the link to download the solutions for chemistry class 11 chapter 2 NCERT solutions . The solutions are free of cost.
You can also find important questions to practice several types of questions to deeply understand the chapter.
10. Why is the atomic structure chapter so hard in NCERT Class 11 Chapter - 2?
The atomic structure chapter appears challenging due to its intricate concepts like quantum mechanics and atomic models.Exploring the complexities of atomic structure is facilitated by Class 11 Ch 2 Chemistry NCERT solutions.
11. Who discovered the atomic structure?
The discovery of the atomic structure is attributed to J.J. Thomson, who unveiled it through experiments on cathode rays.Unlock the mysteries of atomic structure with NCERT solutions Class 11 Chemistry Chapter 2.
12. What are all the principles of structure of atoms according to Class 11 Chapter - 2?
The absolute principles governing the structure of atoms encompass quantum mechanics, electron configuration, and atomic models.Delve into the Class 11 structure of atom NCERT solutions for comprehensive understanding.
13. What was Rutherford's model of the atom Class 11 Chapter - 2?
Rutherford's class 11 model illustrates atoms as primarily empty space, housing a dense, positively charged nucleus, as per his gold foil experiment.Class 11 Chemistry NCERT solutions provide clarity on Rutherford's model and other atomic theories in Chapter 2.