NCERT Solutions for Maths Class 12 Chapter 12 Exercise 12.1 - FREE PDF Download
FAQs on NCERT Solutions for Class 12 Maths Chapter 12 Linear Programming Ex 12.1
1. What are the steps to solve linear programming?
Here are the steps to solve linear programming:
Determine the factors that influence decisions.
Compose the objective function.
Determine the constraints
Select the approach to the linear programming issue.
Construct the graph
Identify the feasible region
Find the optimum point
2. What do you mean by Linear programming?
When a linear function is exposed to various constraints, it is maximised or reduced using the mathematical modelling technique known as "linear programming." In commercial planning and industrial engineering, this approach has worked well for guiding quantitative decisions in the physical and social sciences, though to a lesser extent.
3. How many questions are there in Class 12 Maths Chapter 12 Linear Programming (Ex 12.1) Exercise 12.1?
Class 12 Maths Chapter 12 Linear Programming (Ex 12.1) Exercise 12.1 consists of a total of ten conceptual questions.
4. Why should I practice class 12 maths NCERT chapter 12 Linear Programming (Ex 12.1) Exercise 12.1?
The NCERT book and solutions have been prepared by the best and most highly skilled educators and scholars and have created the content in such a way that all the maths concepts could be understood by each student. Practising exercise 12.1 will help you in clearing your basics about the concept of Linear programming and will help as you go further through the chapter. The NCERT math book has explained the concept of linear programming, on which the first exercise is based, with the help of solved examples that are written in the easiest way.
5. Where can I find the NCERT solutions for Class 12 Maths chapter 12 Linear Programming (Ex 12.1) Exercise 12.1?
NCERT solutions for class 12 maths chapter 12 linear programming (Ex 12.1) exercise 12.1 are free to download in pdf format from Vedantu. Teachers at Vedantu are highly skilled and experts in their subjects, and they have curated these NCERT solutions according to the latest CBSE pattern and guidelines.
6. What is the main focus of Class 12 Maths ex 12.1 solutions?
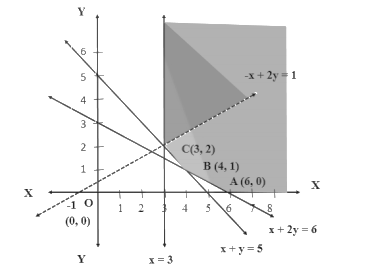
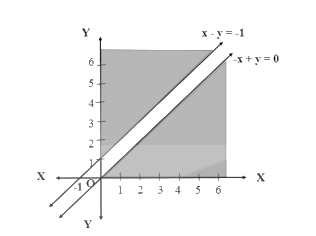
Class 12 Maths ex 12.1 solutions focuses on the basics of linear programming, including formulating linear programming problems, understanding constraints, and using the graphical method to find feasible and optimal solutions.
7. How does the graphical method help in solving linear programming problems in class 12 maths chapter 12 exercise 12.1?
The graphical method helps by visually representing constraints on a graph, identifying the feasible region, and determining the optimal solution by evaluating the objective function at the corner points of the feasible region in class 12 linear programming exercise 12.1.
8. What are the key components of a linear programming problem in class 12 exercise 12.1?
The key components include the objective function to be optimized, constraints represented by linear inequalities, and the feasible region where these constraints overlap, forming the solution space.
9. Why is understanding constraints important in linear programming in class 12 maths ch 12 ex 12.1?
In linear programming class 12 maths ch 12 ex 12.1 understanding constraints is crucial because they define the feasible region, which is the set of all possible solutions that satisfy the conditions of the problem. Only within this region can the optimal solution be found.
10. How do Vedantu’s NCERT Solutions assist with ex 12.1 class 12?
Vedantu’s NCERT Solutions of linear programming class 12 exercise 12.1 provide detailed, step-by-step explanations and graphical representations, making it easier for students to understand the concepts and effectively solve linear programming problems.