NCERT Solutions for Class 12 Physics Chapter 3 Current Electricity - FREE PDF Download









FAQs on NCERT Solutions for Class 12 Physics Chapter 3 Current Electricity
1. Which Topics are Covered in Current Electricity?
This chapter primarily deals with the first motion laws, second motion laws, Newton's third motion laws, pseudo forces, the horse and cart and inertia. Moreover, students will also become familiar with electric current, Ohm's law, electric current in conductors, the origin of resistivity, the drift of electrons, Ohm's law limitations, mobility, the resistivity of different materials, electrical power and energy and temperature vulnerability of resistivity.
By studying Current Electricity, students will also get to learn about the resistor combination in both parallel and series, cells, internal resistance, emf, Kirchhoff’s rule, Wheatstone bridge, potentiometer and meter bridge. Some other topics included in this chapter are non-conservation and conservation force field, work-energy theorem, kinetic energy, types of power and equilibrium, the law of conservation of mechanical energy, etc.
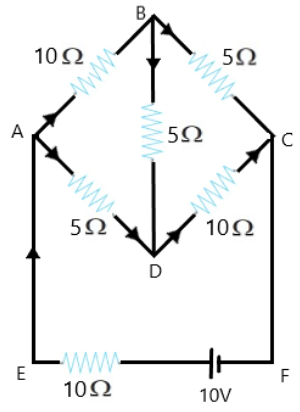
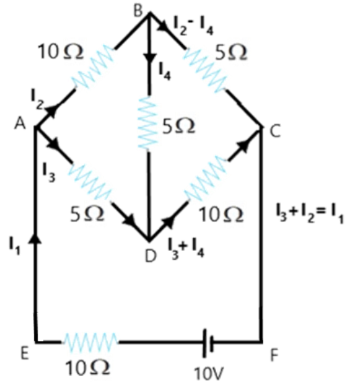
2. What is Meant by a Wheatstone Bridge?
This device is utilised to observe mechanical and electrical quantities. In other words, Wheatstone Bridge is a tool for light measurement with the help of a photo resistive instrument. A light-dependent resistor is a type of sensor which is passive-resistive. It is used to convert light levels to change in resistance, and eventually to voltage.
The circuit of the Wheatstone bridge has many applications today. Along with the current functional amplifiers, the Wheatstone Bridge can be used to interface different sensors and transducers into amplifier circuits.
3. How to Obtain Full Marks in Class 12 Physics?
In order to achieve the full score in the Class 12 Physics exam, the primary requirement is to study the NCERT thoroughly. Also, students must practise multiple choice questions from NCERT and recommended books.
Preparing a formula chart is vital for the learners to practise the numerical problems, and diagrams must also be practised with proper labelling. In the case of long answer questions, students must refrain from writing long paragraphs; instead, they must write in bullet points. Further, solving previous years question papers is also mandatory to score well.
4. How to prepare Class 12 Physics Chapter 3 well for exams?
Current Electricity in Physics for Class 12 can often confuse students due to the many laws and numerical in the chapter. To prepare this chapter well for exams, first, go through all the topics thoroughly. Clear the concepts and solve the numerical problems. Solve the exercises in the books and then re-check them with NCERT Solutions so you can get an idea of how to answer questions in the exams. Make notes so that the revision becomes easier.
5. How can I make a study plan for Class 12 Physics Chapter 3?
Class 12 Chapter 3 can be stressful for the students, so forming a good study plan is very important. To make a study plan for Physics, follow these steps:
Mark the topics you find difficult and give them more time.
Solve some numerical problems every day.
Study at odd hours of the day.
Take breaks in between to soak in all you have studied.
Make notes as you go through the chapters.
6. Where can I download Physics NCERT Solutions for Class 12 Chapter 3 PDF?
You can download the PDF for Physics NCERT Solutions for Class 12: Chapter 3 using the official website of Vedantu or the Vedantu app. Vedantu provides all the study material free of cost. Visit NCERT Solutions chapter 3 Current and Electricity and you will land on the page with chapter-wise solutions. Choose Chapter 3: Current Electricity. Thereafter, you will be able to download the NCERT Solution and other study material for the chapter. These solutions can be a student's best study buddy as they help in providing the best guidance and make learning fun.
7. Why are the Class 12 Physics Chapter 3 NCERT Solutions important?
NCERT Solutions for Chapter 3 “Current Electricity” in Class 12 Physics is essential for the exams and can often confuse the students. Using the solutions will help you clear all the concepts and guide you in solving the numerical problems. They also give you a fair idea about the type of questions in exams and how to answer them. It is essential to be well-prepared for the exams and NCERT Solutions are the best guidance you can find online.
8. Do I need to practice all the questions provided in Class 12 Physics NCERT Solutions of Chapter 3?
Solving all the questions provided in Chapter 3 of Class 12 Physics NCERT Solutions will help you comprehend the concepts in the chapter intensively and clear your doubts. The answers to the questions from the NCERT textbook in the solutions are designed following the CBSE board guidelines by experts in each subject. Therefore, solving and practising all of them regularly will bring you a step closer to achieving good marks in the exams.
9. Why is current electricity important mentioned in Chapter 3 Physics Class 12?
Current electricity is essential because it forms the foundation of modern electrical and electronic systems. It is crucial for the functioning of household appliances, industrial machinery, communication devices, and medical equipment. Understanding current electricity helps in designing circuits, troubleshooting electrical issues, and developing new technologies.
10. What initiates current in Current Electricity Class 12 NCERT Solutions?
Electric current is initiated when there is a potential difference (voltage) across a conductor, causing the free electrons in the conductor to move. This movement of electrons constitutes an electric current. The potential difference can be created using a power source such as a battery or a generator.
11. How is current electricity produced that is mentioned in Current Electricity Class 12 Exercise Solutions?
Current electricity is produced when electrons move through a conductor due to a potential difference. This can be achieved by connecting a conductor to a power source (like a battery) that provides the necessary voltage to drive the electrons from one point to another, creating a flow of electric charge.
12. What is the SI unit of current according to Class 12 Physics Chapter 3 NCERT Solutions?
In Class 12 Physics Chapter 3 NCERT Solutions, the SI unit of electric current is the ampere (A). One ampere is defined as the flow of one coulomb of charge per second.
13. What is the theory of Current Electricity in Class 12 Solutions?
The theory of Current Electricity involves the study of how electric charges move through a conductor and the laws governing this movement. Key concepts include Ohm’s Law, Kirchhoff’s laws, and the relationship between voltage, current, resistance, and power. It also covers the behaviour of materials under the influence of an electric field and the properties of conductors, insulators, and semiconductors.
14. What is the symbol for the current in Class 12 Physics Ch 3 NCERT Solutions?
In physics chapter 3 class 12 exercise solutions, the symbol for electric current is I. It is typically represented in equations and circuit diagrams to denote the flow of electric charge.
15. How does current electricity work as described in Current Electricity NCERT Solutions?
Current electricity works by the movement of electrons through a conductor. When a potential difference is applied across a conductor, it creates an electric field that exerts a force on the free electrons, causing them to move. This flow of electrons constitutes an electric current. The current flows from the negative terminal to the positive terminal of the power source, although conventionally, it is considered to flow from positive to negative.
16. What is the formula for current electricity?
The basic formula for electric current is: I=
where
I is the current,
Q is the charge, and
t is the time.
Another important formula is Ohm’s Law:
V=IR
where
V is the voltage,
I is the current, and
R is the resistance.
17. What is the gist of Current Electricity in Chapter 3 Physics Class 12 NCERT Solutions?
The gist of ch 3 physics class 12 current electricity is the study of how electric charges flow through conductors and the principles that govern this flow. It involves understanding the relationship between voltage, current, and resistance, and applying laws such as Ohm's Law and Kirchhoff's Laws to analyse electric circuits. This knowledge is fundamental for designing, operating, and troubleshooting electrical and electronic systems.