Patterns In Mathematics Class 6 Questions and Answers - Free PDF Download
NCERT Solutions for Class 6 Maths Chapter 1, Exercise 1.3, "Visualising Number Sequences," are designed to help students understand and work with number sequences effectively. This exercise aligns with the CBSE Class 6 Maths Syllabus and offers clear explanations with step-by-step guidance. It helps students recognise and follow different number sequences, making it easier to handle patterns systematically.
Table of Content
Students can download these NCERT Solutions for Maths Class 6 as a FREE PDF, which makes it simple for students to practise and improve their problem-solving skills. The solutions include helpful tips and shortcuts to make learning more interesting and effective. These resources support students in understanding number sequences and preparing for more advanced maths topics.
Glance on NCERT Solutions Maths Chapter 1 Exercise 1.3 Class 6 | Vedantu
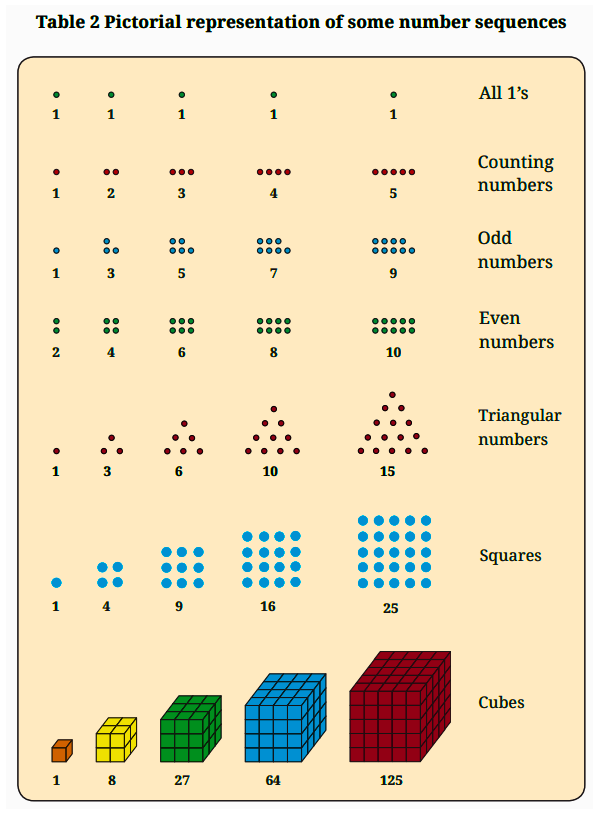
NCERT Solutions for Chapter 1, Exercise 1.3, focuses on visualising number sequences.
This exercise helps students learn how to identify and understand various number sequences, such as arithmetic sequences and patterns.
The solutions offer step-by-step explanations, making it easier to follow and practise these sequences.
By working through this exercise, students can improve their ability to see and predict how numbers progress in a sequence.
The clear guidance provided in the solutions supports students in developing a strong foundation in recognising and using number sequences.
Figure it Out
1. Copy the pictorial representations of the number sequences in Table 2 in your notebook, and draw the next picture for each sequence!
Ans:
2. Why are 1, 3, 6, 10, 15, … called triangular numbers?
Why are 1, 4, 9, 16, 25, … called square numbers or squares?
Why are 1, 8, 27, 64, 125, … called cubes?
Ans: 1, 3, 6, 10, 15, … called triangular numbers: These numbers are called triangular numbers because they can be arranged in the shape of an equilateral triangle. For example, 3 can be arranged as a triangle with 2 dots in the base and 1 dot at the top. Each number in the sequence represents the total number of dots that form a triangle when arranged in increasing rows.
1, 4, 9, 16, 25, … called square numbers or squares: These numbers are called square numbers because they represent the area of a square. For example, 4 is the area of a square with sides of length 2 (2 × 2 = 4). Each number in this sequence is the product of a number multiplied by itself, which forms the area of a square.
1, 8, 27, 64, 125, … called cubes: These numbers are called cubes because they represent the volume of a cube. For example, 8 is the volume of a cube with each side of length 2 (2 × 2 × 2 = 8). Each number in the sequence is the result of multiplying a number by itself twice, which gives the volume of a cube.
3. You will have noticed that 36 is both a triangular number and a square number! That is, 36 dots can be arranged perfectly both in a triangle and in a square. Make pictures in your notebook illustrating this! This shows that the same number can be represented differently, and play different roles, depending on the context. Try representing some other numbers pictorially in different ways!
Ans: Three other numbers that can be both triangular and square are 1, 1225, and 41616.
For instance, 1225 is the 49th triangular number, meaning it can be arranged in a triangular pattern with 49 rows.
Additionally, 1225 can also be arranged in a square, with each side containing 35 dots (since 35 × 35 = 1225).
This shows how certain numbers can be represented in multiple ways, demonstrating their versatility in both triangular and square formations.
The fact that a number like 1225 fits perfectly into both shapes highlights the fascinating connections between different geometric and mathematical properties.
4. What would you call the following sequence of numbers?
That’s right, they are called hexagonal numbers! Draw these in your notebook. What is the next number in the sequence?
Ans: Let's break down the pattern in this sequence:
1st number = 1
2nd number = 1 + 6 = 7 (This is found by adding 6 × 1 to the 1st number)
3rd number = 7 + 12 = 19 (This is found by adding 6 × 2 to the 2nd number)
4th number = 19 + 18 = 37 (This is found by adding 6 × 3 to the 3rd number)
5th number = 37 + 24 = 61 (This is found by adding 6 × 4 to the 4th number)
Thus, the pattern involves adding multiples of 6, with each multiple increasing by 6. So, to find the next number in the sequence, you would add 6 × 5 = 30 to the 5th number (61). Therefore, the next number in the sequence would be 61 + 30 = 91.
This sequence demonstrates a regular pattern of growth, with each term increasing by a progressively larger multiple of 6.
5. Can you think of pictorial ways to visualise the sequence of Powers of 2? Powers of 3?
Here is one possible way of thinking about Powers of 2:
Ans: Pictorial Representation for powers of 3:
Benefits of NCERT Solutions for Class 6 Maths Chapter 1 Exercise 1.3 Patterns in Mathematics
The solutions help students see and understand different number sequences and patterns.
They enhance student’s ability to identify and predict number patterns, which is essential for solving related maths problems.
Detailed explanations and methods make it easier to follow and learn how to work with number sequences.
The exercise builds a solid base for understanding more advanced mathematical concepts involving sequences and patterns.
The engaging practice through practical exercises helps keep students motivated and interested in maths.
Available as a FREE PDF download, the solutions provide convenient access for practice and review anytime.
Class 6 Maths Chapter 1: Exercises Breakdown
Important Study Material Links for Class 6 Maths Chapter 1 - Patterns in Mathematics
Conclusion
NCERT Solutions for Class 6 Maths Chapter 1 Exercise 1.3, "Visualising Number Sequences," helps students understand different number sequences with clear explanations. Practising these exercises enhances their ability to recognise and predict patterns, supporting their overall learning and problem-solving skills. These solutions make studying and practising easier, helping students feel more confident in their understanding of sequences. Available as a FREE PDF download, they offer a convenient way for students to study and review at their own pace.
Chapter-Specific NCERT Solutions for Class 6 Maths
The chapter-wise NCERT Solutions for Class 6 Maths are given below. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
Related Important Links for Maths Class 6
Along with this, students can also download additional study materials provided by Vedantu for Maths Class 6.
FAQs on NCERT Solutions For Class 6 Maths Chapter 1 Patterns In Mathematics Exercise 1.3 - 2025-26
1. What concepts are covered in NCERT Solutions for Class 6 Maths Chapter 1 Exercise 1.3?
The NCERT Solutions for Class 6 Maths Chapter 1 Exercise 1.3 focus on recognising, visualising, and analysing different types of number sequences, including triangular numbers, square numbers, cubes, and hexagonal numbers. The exercise strengthens problem-solving by teaching students to spot mathematical patterns and understand how sequences evolve as per CBSE 2025–26 guidelines.
2. How do the solutions for Class 6 Maths Patterns in Mathematics Exercise 1.3 support CBSE exam preparation?
These NCERT Solutions follow the CBSE methodology, providing step-by-step explanations for identifying and extending number patterns. Practicing these builds the analytical skills needed for CBSE-style questions, ensuring students can tackle related concept-based questions confidently in the 2025–26 exams.
3. Why are some numbers called triangular, square, or cube numbers in Chapter 1 Maths Class 6 solutions?
- Triangular numbers: Formed by arranging objects in a triangle. For example, 6 can make a triangle with 3 rows.
- Square numbers: Result from multiplying a number by itself (2 × 2 = 4). Represent areas of squares.
- Cubes: Found by multiplying a number by itself twice (2 × 2 × 2 = 8). Represent volumes of cubes. These definitions, given in NCERT Solutions, help students visually relate to number patterns in Class 6 Maths.
4. What is the significance of visualising number sequences in NCERT Solutions for Class 6 Maths Chapter 1?
Visualising number sequences helps students recognise how numbers progress, predict future terms, and understand deeper mathematical connections. The ability to see these patterns is critical for problem-solving and for grasping advanced maths concepts as outlined in the CBSE Class 6 2025–26 syllabus.
5. How can incorrect pattern recognition affect solving problems in Class 6 Maths Chapter 1?
If students misidentify a pattern (for example, mistaking an arithmetic sequence for a geometric one), they may apply the wrong rule and get incorrect answers. The NCERT Solutions demonstrate the correct methodology, helping students avoid such misconceptions in pattern-based questions.
6. What strategies are suggested in NCERT Solutions for solving number pattern questions in Exercise 1.3?
- Look for a consistent rule or relationship between numbers (add, multiply, etc.).
- Check if the change between numbers is constant (arithmetic) or a factor (geometric).
- Pictorially represent sequences to spot patterns visually.
- Use stepwise reasoning as shown in solutions to ensure logical accuracy.
7. What type of number patterns are classified as hexagonal numbers in Class 6 NCERT Solutions?
Hexagonal numbers are a special sequence where the difference between terms increases by multiples of 6. For example: 1, 7, 19, 37, etc. Each term is built by adding 6 more than was added to get the previous term. NCERT Solutions guide students to identify and extend such patterns.
8. How do the NCERT Solutions help in mastering skipped logic or missing terms in number sequences?
NCERT Solutions highlight how to identify the underlying logic even when sequence terms are missing. By focusing on consistent steps or differences, students learn to predict missing numbers and explain their reasoning, which is crucial for full marks in the CBSE 2025–26 format.
9. How can students avoid common errors in Exercise 1.3 of Class 6 Maths Chapter 1?
- Always check the rule for every part of the sequence before answering.
- Draw pictorial or item-wise representations as suggested in NCERT Solutions.
- Verify answers by reconstructing the pattern from the identified rule.
- Read each question carefully to determine the type of pattern (triangular, square, etc.).
10. How do NCERT Solutions for Class 6 Maths Chapter 1 build a foundation for higher mathematics?
By consistently working through different number sequences and patterns, students develop analytical thinking and reasoning skills. These are the building blocks for advanced topics such as algebra and series, forming a strong base for future learning as per the Class 6 Maths curriculum for 2025–26.
11. What should a student do if they struggle with visualising number sequences in Exercise 1.3?
Students should revisit the pictorial representations provided in the NCERT Solutions, try drawing each sequence step-by-step, and compare their approach with the methods shown. Practicing with the given solved examples and referring to the reasoning will help improve pattern recognition.
12. How are practice problems structured in Class 6 Maths Chapter 1 NCERT Solutions to reinforce learning?
The practice problems are arranged in increasing order of difficulty. Each problem encourages logical deduction, proper application of sequence rules, and offers detailed, stepwise solutions to strengthen understanding of Patterns in Mathematics for CBSE Class 6.
13. What are some common CBSE exam questions based on Class 6 Maths Chapter 1 number sequences?
CBSE often asks students to:
- Identify the next term in a given pattern.
- Explain why a number is triangular, square, or a cube.
- Draw representations for number patterns.
- Find missing terms in a sequence using proper logic.
14. How often should students practise number pattern problems from NCERT Solutions for best results?
Regular practice is advised—working through a few problems daily or every alternate day helps solidify understanding, supports long-term retention, and ensures readiness for CBSE 2025–26 Maths exams.
