NCERT Solutions for Class 8 Maths Chapter 3 Understanding Quadrilaterals - FREE PDF Download
FAQs on NCERT Solutions for Class 8 Maths Chapter 3 Understanding Quadrilaterals
1. What is the Area of a Field in the Shape of a Rectangle with Dimensions of 20 Meters and 40 Meters?
We know that the field is rectangular. Hence, we can apply the area of a rectangle to find the field area.
Length of the field = 40 Metre
Width of the field = 20 Metre
Area of the rectangular field = Length × Width = 40 × 20 = 800 Sq. Meters.
We know if the length of the rectangle is L and breadth is B then,
Area of a rectangle = Length × Breadth or L × B
Perimeter = 2 × (L + B)
So, the properties and formulas of quadrilaterals that are used in this question:
Area of the Rectangle = Length × Width
So, we used only a specific property to find the answer.
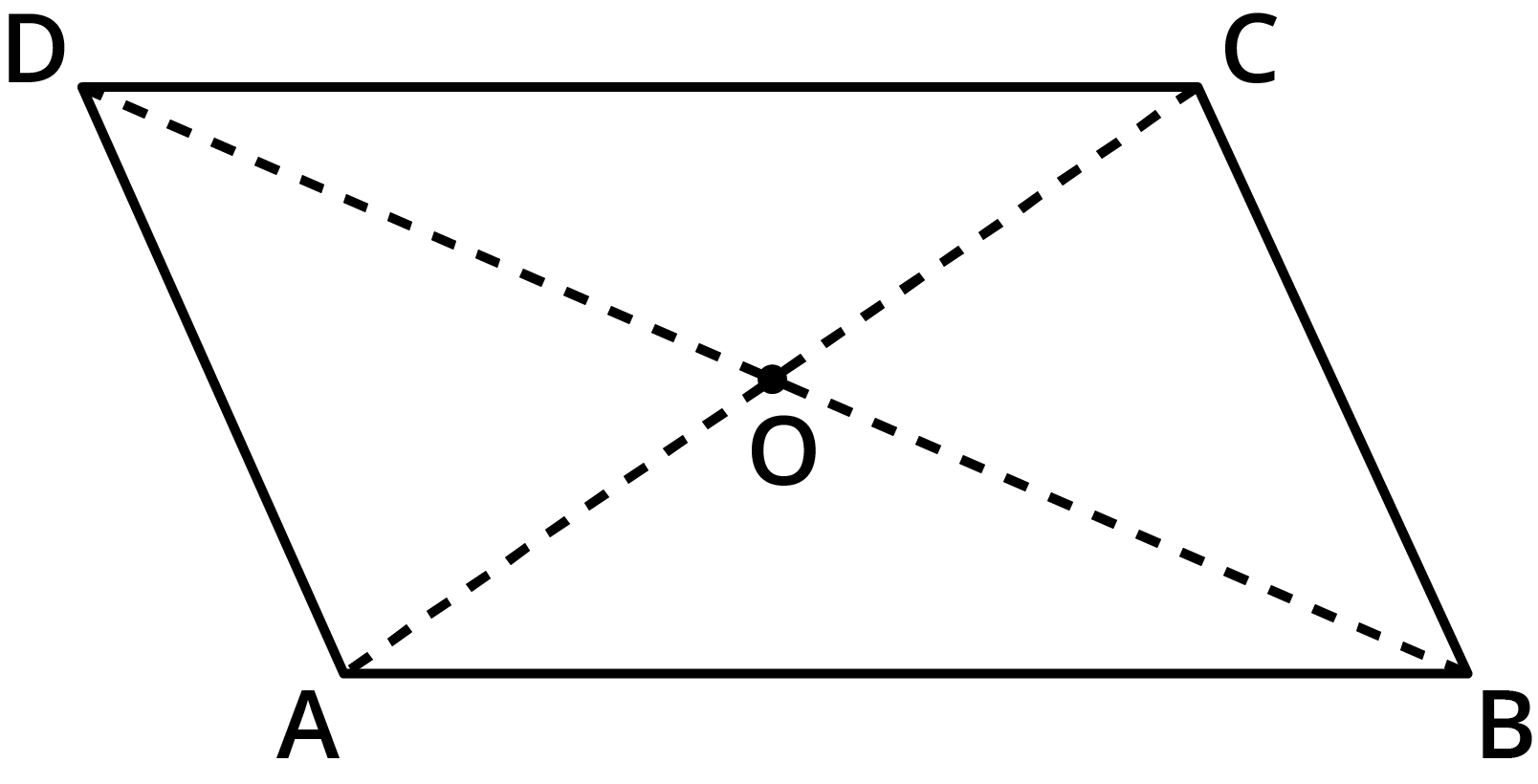
2. Find the Rest of the Angles of a Parallelogram if one Angle is 80°?
For a parallelogram ABCD, as we know the properties:
Opposite angles are equal.
Opposite sides are equal and parallel.
Diagonals bisect each other.
The summation of any two adjacent angles = 180 degrees.
So, the angles opposite to the provided 80° angle will likewise be 80°.
Like we know, know that the Sum of angles of any quadrilateral = 360°.
So, if ∠A = ∠C = 80° then,
Sum of ∠A, ∠B, ∠C, ∠D = 360°
Also, ∠B = ∠D
Thus,
Sum of 80°, ∠B, 80°, ∠D = 360°
Or, ∠B +∠ D = 200°
Hence, ∠B = ∠D = 100°
Now, we found all the angles of the quadrilateral, which are:
∠A = 80°
∠B = 100°
∠C = 80°
∠D = 100°
3. Why are the NCERT Solutions for Class 8 Maths Chapter 3 important?
The questions included in NCERT Solutions for Chapter 3 of Class 8 Maths are important not only for the exams but also for the overall understanding of quadrilaterals. These questions have been answered by expert teachers in the subject as per the NCERT (CBSE) guidelines. As the students answer the exercises, they will grasp the topic more comfortably and in a better manner.
4. What are the main topics covered in NCERT Solutions for Class 8 Maths Chapter 3?
All the topics of the syllabus of Class 8 Maths Chapter 3 have been dealt with in detail in the NCERT Solutions by Vedantu. The chapter is Understanding Quadrilaterals and has four exercises. All the important topics in Quadrilaterals have also been carefully covered. Students can also refer to the important questions section to get a good idea about the kind of questions usually asked in the exam.
5. Do I need to practice all the questions provided in the NCERT Solutions Class 8 Maths “Understanding Quadrilaterals”?
It helps to solve as many questions as possible because Mathematics is all about practice. If you solve all the practice questions and exercises given in NCERT Solutions for Class 8 Maths, you will be able to score very well in your exams comfortably. This will also help you understand the concepts clearly and allow you to apply them logically in the questions.
6. What are the most important concepts that I need to remember in Class 8 Maths Chapter 3?
For Class 8 Maths Chapter 3, you must remember the definition, characteristics and properties of all the quadrilaterals prescribed in the syllabus, namely, parallelogram, rhombus, rectangle, square, kite, and trapezium. Also know the properties of their angles and diagonals. Regular practise will help students learn the chapter easily.
7. Is Class 8 Maths Chapter 3 Easy?
Class 8 chapter 3 of Maths is a really interesting but critical topic. It's important not only for the Class 8 exams but also for understanding future concepts in higher classes. So, to stay focused and get a good grip of all concepts, it is advisable to download the NCERT Solutions for Class 8 Maths from the Vedantu website or from the Vedantu app at free of cost. This will help the students to clear out any doubts and allow them to excel in the exams.
8. In Maths Class 8 Chapter 3, how are quadrilaterals used in everyday life?
Quadrilaterals are everywhere! Here are some examples:
Shapes in your house: Doors, windows, tabletops, picture frames, book covers, even slices of bread are quadrilaterals (mostly rectangles).
Construction and design: Architects use rectangles and squares for walls, floors, and windows. Roads and bridges often involve trapezoids and other quadrilaterals for support.
Everyday objects: Stop signs, traffic signals, and many sports fields (like baseball diamonds) are quadrilaterals.
9. How many quadrilaterals are there in Class 8 Chapter 3 Maths?
There are many types of quadrilaterals mentioned in Class 8 Understanding Quadrilaterals, but some of the most common include:
Rectangle (all four angles are 90 degrees, opposite sides are equal and parallel)
Square (a special rectangle with all sides equal)
Parallelogram (opposite sides are parallel)
Rhombus (all four sides are equal)
Trapezoid (one pair of parallel sides)
10. What are real examples of quadrilaterals in Class 8 Chapter 3 Maths?
Some real examples of quadrilaterals are:
Rectangle: Doorway, window pane, sheet of paper, tabletop, chocolate bar, playing card (most common)
Square: Dice, coaster, napkin, wall tiles (when all sides are equal)
Parallelogram: Textbook cover, kite (when opposite sides are parallel), solar panel
Rhombus: Traffic warning sign (diamond shape with all sides equal)
Trapezoid: Slice of pizza, roof truss (one pair of parallel sides)
Irregular Quadrilateral: Flag (many flags like the US flag are not perfectly symmetrical quadrilaterals)
11. How do you identify a quadrilateral in Maths Class 8 Chapter 3?
A quadrilateral has the following properties:
Four straight sides
Four angles (interior angles add up to 360 degrees)
Four vertices (corners where two sides meet)