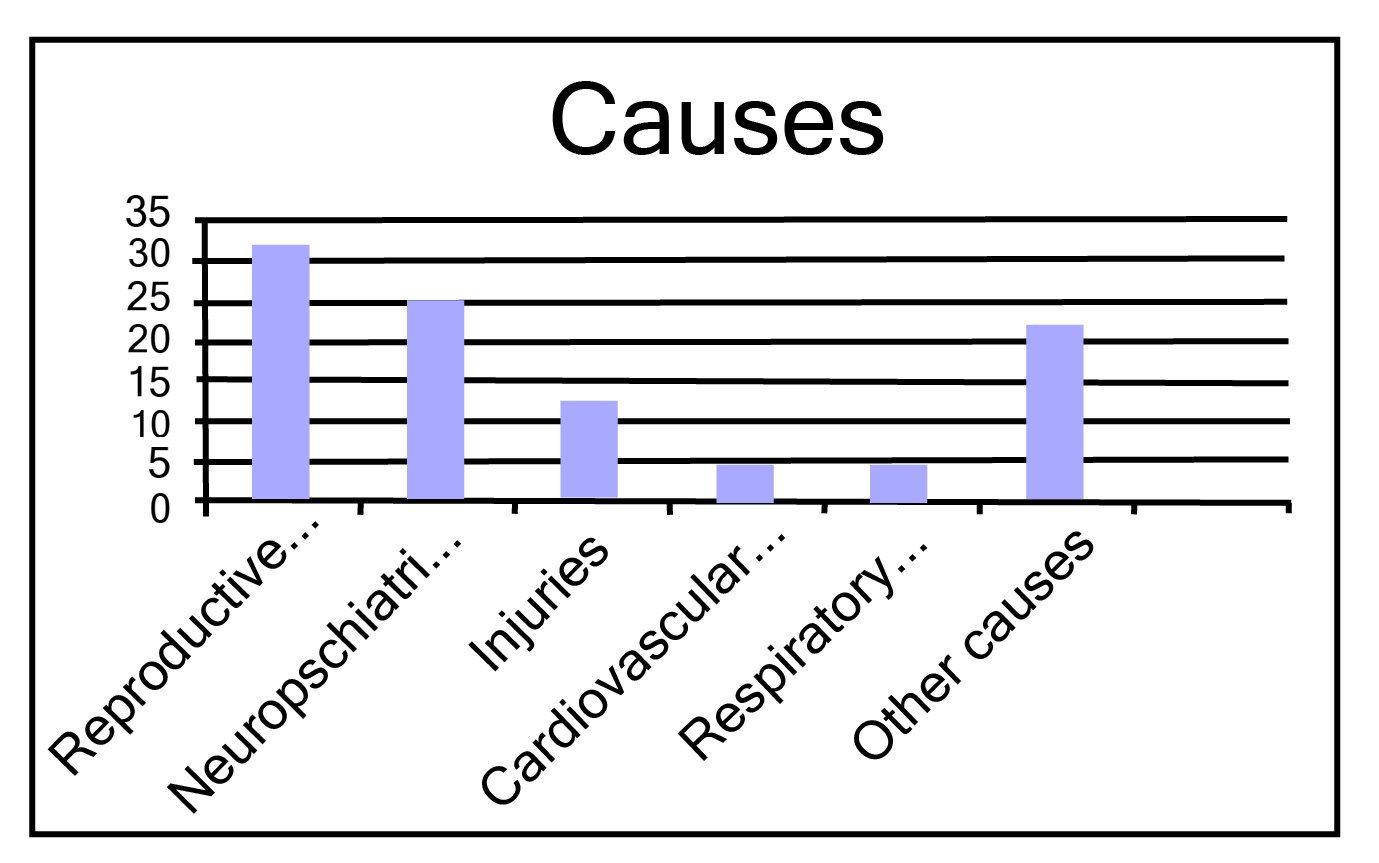
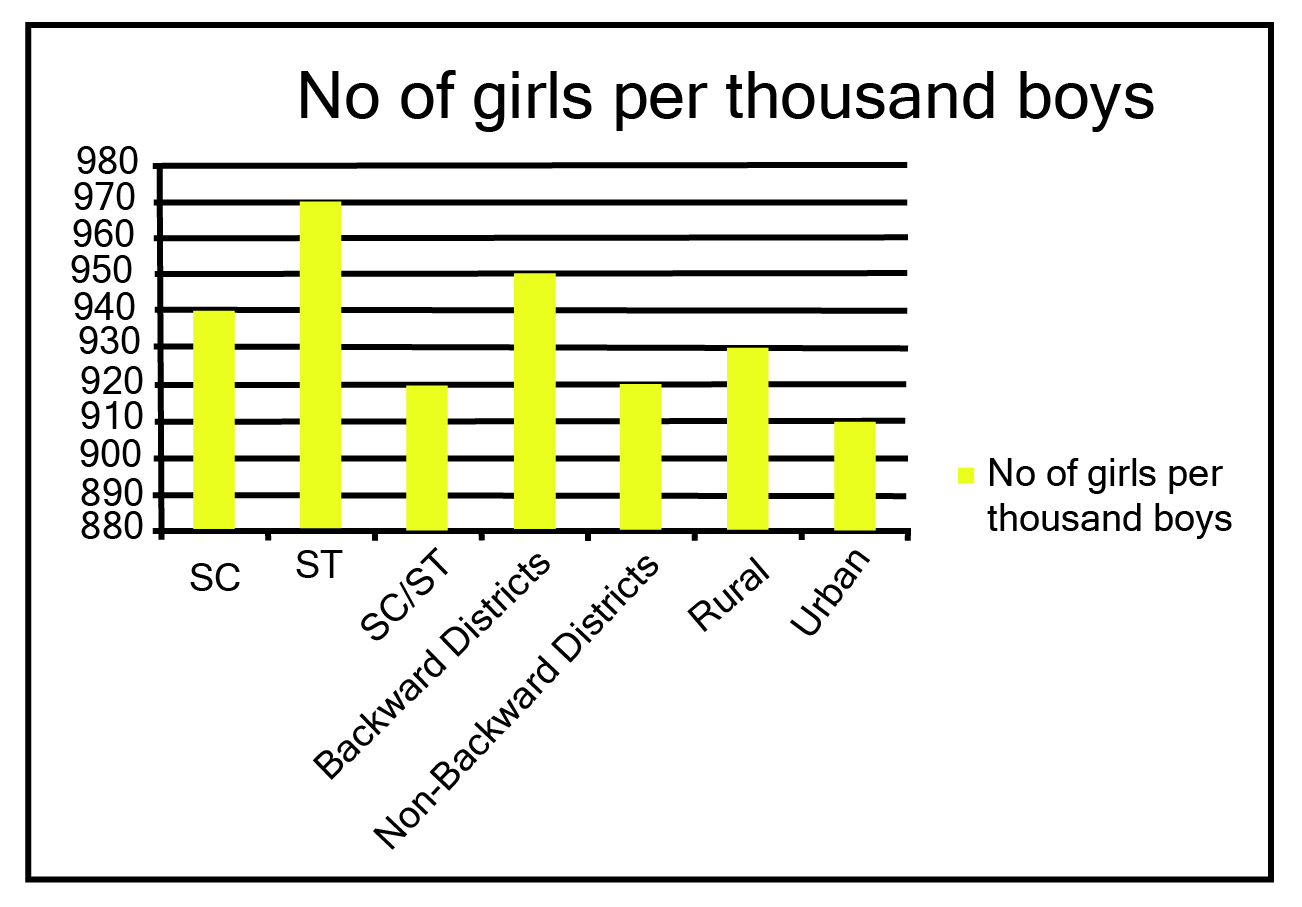
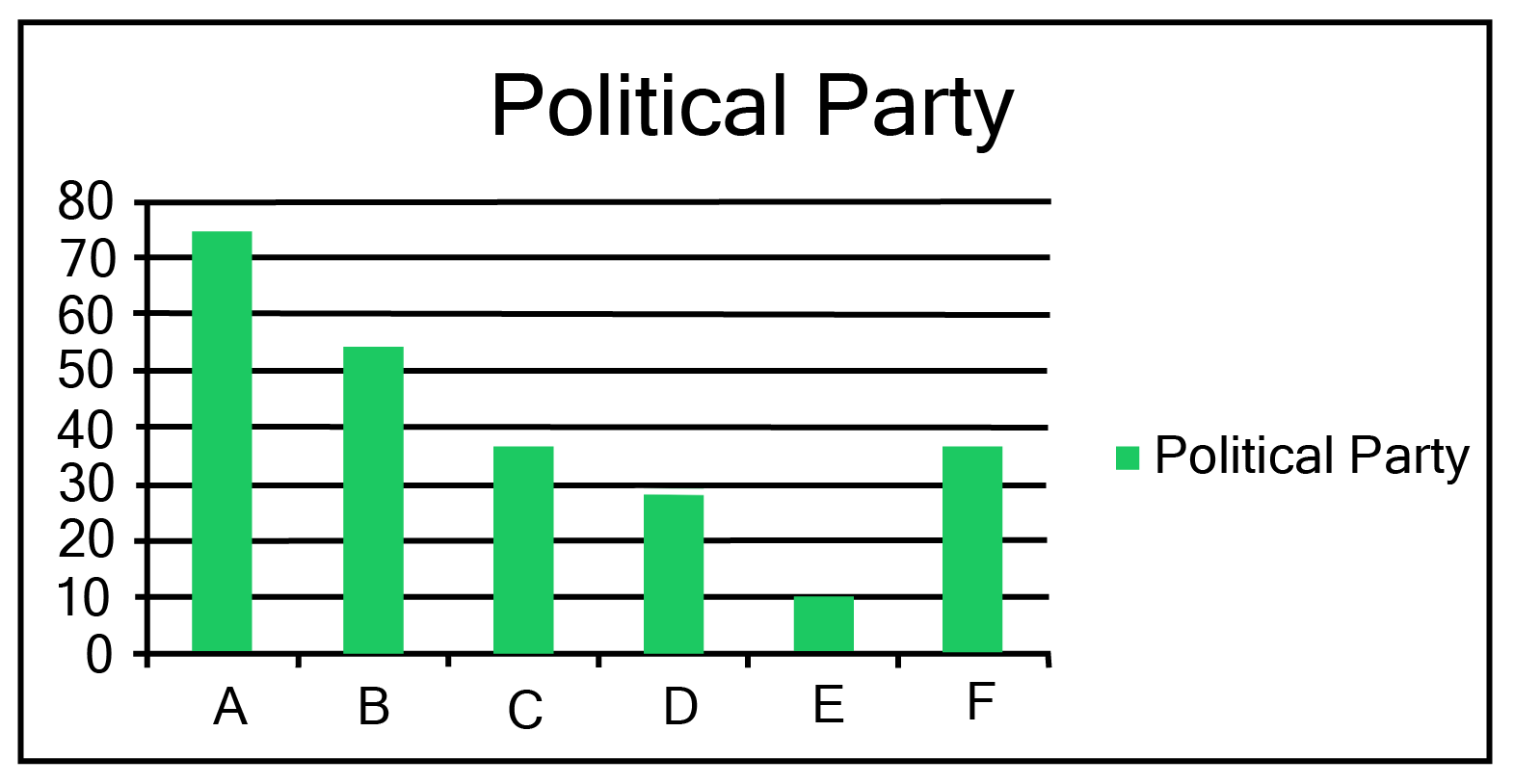
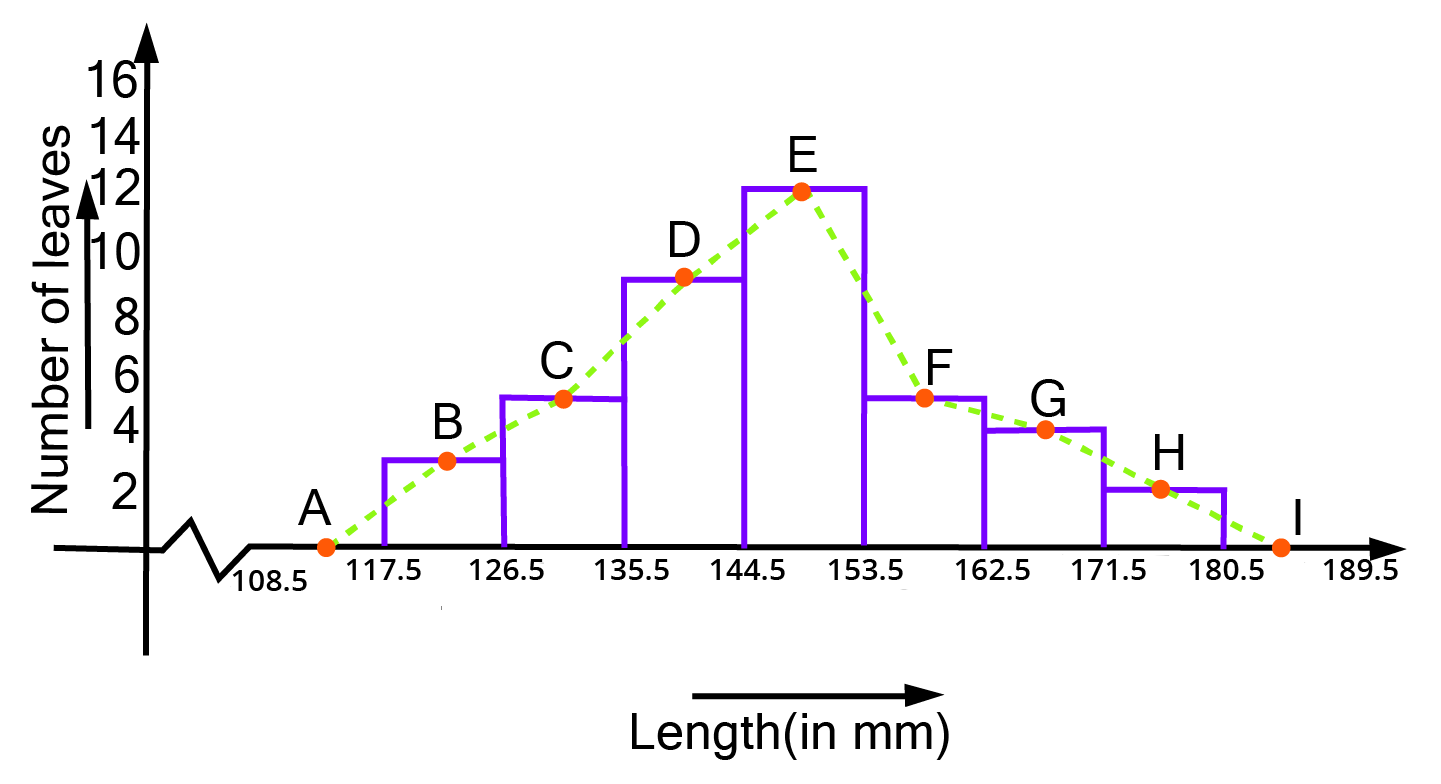
NCERT Solutions for Class 9 Maths Exercise 12.1 Chapter 12 Statistics - FREE PDF Download






NCERT Solutions for Class 9 Maths Chapter 12 Statistics Ex 12.1
FAQs on NCERT Solutions for Class 9 Maths Chapter 12 Statistics Ex 12.1
1. What is Statistics? Explain its Significance?
Statistics is one of the mathematical disciplines. It helps to organize the data. It analyzes and quantifies the data using different measures. It also helps to represent data in various forms and can draw conclusions from them.
Statistics play a vital role in all kinds of industries. Because, irrespective of the type of data, data is mandatory for firm's and it is important to analyze it. It has a great career too.
2. Define the Mean, Median, and Mode?
The basic statistical measures are mean, median, and mode. So to get enough knowledge, it is important to understand these three definitions and the difference between them.
Mean: The term means refers to average. Mean is defined as the average of the given set of data. We can calculate by dividing the sum of all values by several values.
Median: Simply, the middle term of the set is known as the median of the set. If it has an even number of terms in the set, the average of two middle terms is the median.
Mode: The highest repetitive term of the set is called the mode of the set.
3. Which chapter is Exercise 12.3 a part of?
Exercise 12.3 of Class 9 Maths is a part of the chapter on Statistics. It deals with the practice of collecting, analyzing, and surveying numerical data that are present in large proportions. Furthermore, it is the study of this data that includes gathering, reviewing, and ultimately arriving at a conclusion on the given data. You can get all the study resources related to the chapter on the Vedantu app and website.
4. What will students learn in NCERT Class 9 Maths Chapter 12 Exercise-12.3?
The NCERT Class 9 Maths Chapter 12 Exercise-12.3 help students decipher all the basic concepts, formulas, and equations related to Statistics. The sums in this exercise elucidate every concept from an applicational point-of-view, making it easier to understand for students. Moreover, there are solved examples that help students to get hold of the different question patterns asked in the examination.
5. How many sums are given in NCERT Class 9 Maths Chapter 12 Ex-12.3?
There are a total of 9 questions given in Class 9 Maths Chapter 12 Ex-12.3. The sums of this exercise require understanding and solving survey, data, and graph-related questions and practicing these with the help of graph plotting. The graphs are a pictorial representation of the statistical or functional relationships between variables and help in summarizing sets of data and give solutions for practical data. Practicing these sums will help students learn the basic concepts and techniques while working with graphs, which will help them in learning advanced mathematics as well.
6. Can I download NCERT Solutions for Class 9 Maths Chapter 12 Ex-12.3 PDF for free?
Yes, NCERT Solutions for Class 9 Maths Chapter 12 Ex-12.3 are available on Vedantu in the PDF format. Students can download the NCERT Solutions PDF for free from the Vedantu. Also, they can download the ebook of NCERT Class 9 Maths from Vedantu for their practice purpose. The solution PDF for NCERT Solutions Class 9 Maths Chapter 12 Exercise 12.3 can be downloaded for free of cost from the Vedantu website or mobile app. Students can also take a hard copy of the solutions PDF and refer to it for their practice.
7. Are the sums in Chapter 12 Exercise 12.3 of Class 9 Maths easy to solve?
Students need to be thorough in their concepts before solving the sums of Chapter 12 Exercise 12.3 of Class 9 Maths. By solving the sums given in Exercise 12.3 students will be able to strengthen their understanding of the concepts. Also, with good practice, they will be able to solve the sums swiftly during the examination, and have ample amount of time to revise their solutions. Thus, it boosts their confidence during the examination and helps them solve questions without any doubt.
8. What topics are covered in Class 9 Maths 12.1 of Chapter 12 (Statistics) in NCERT?
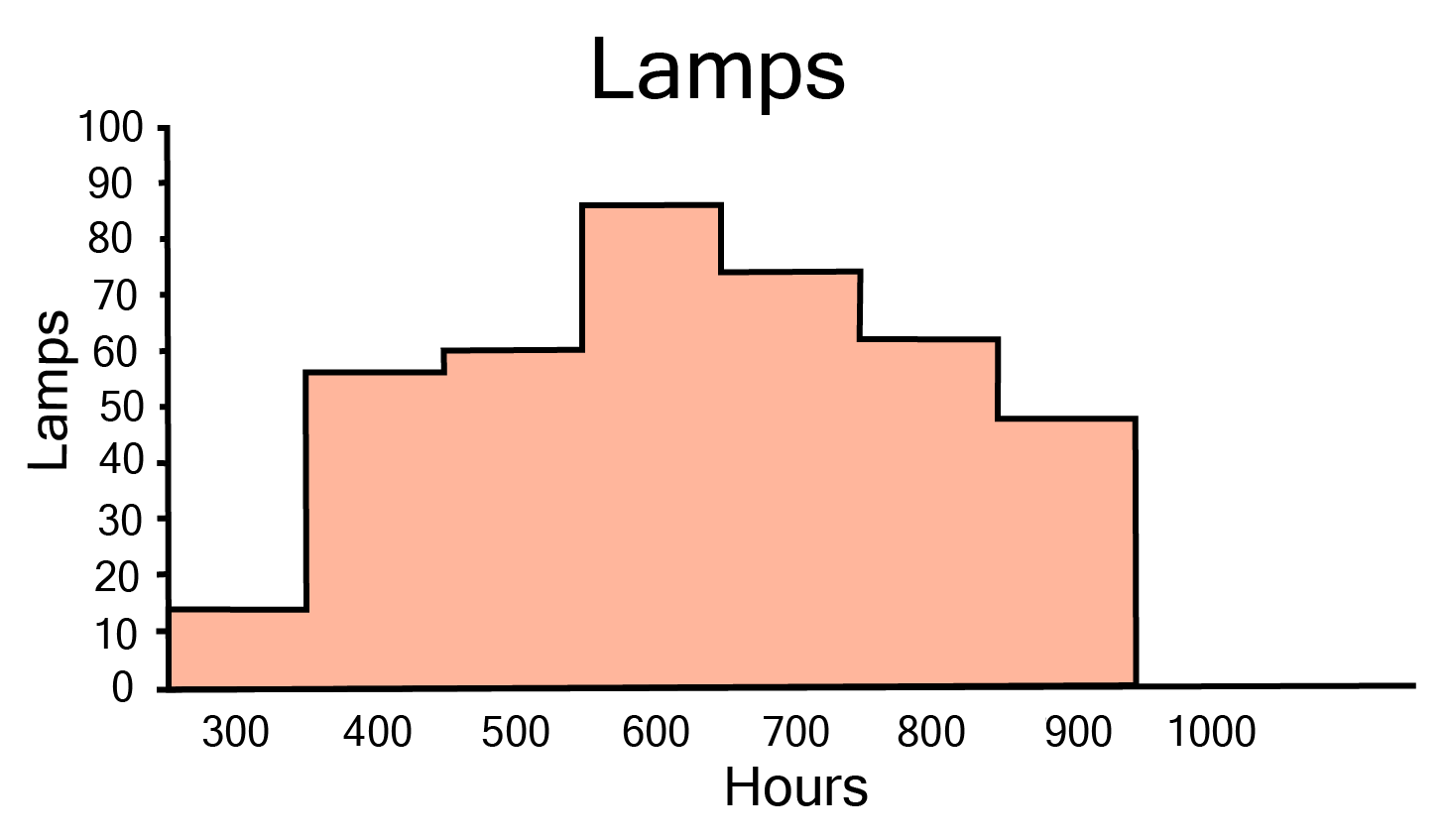
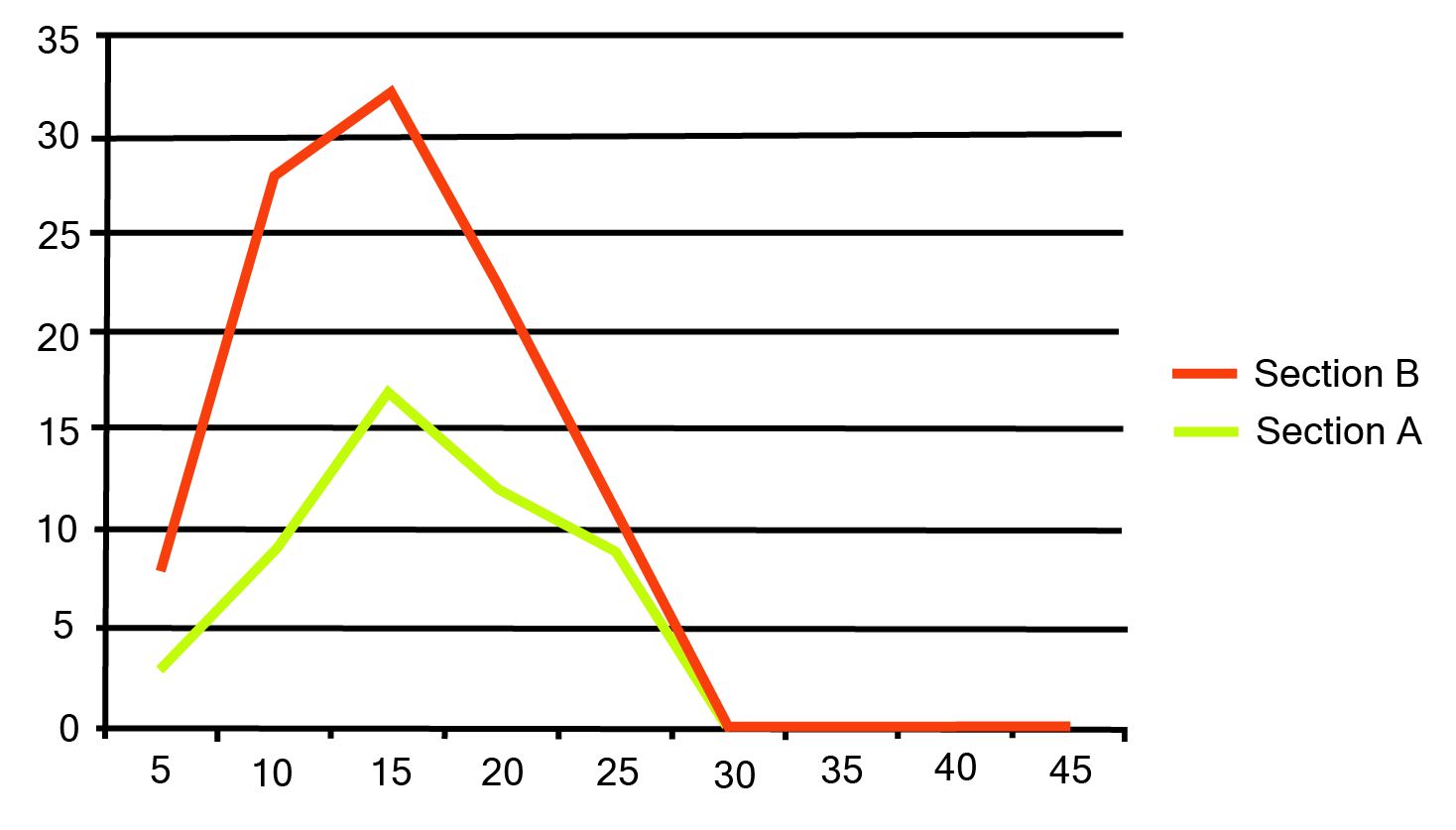
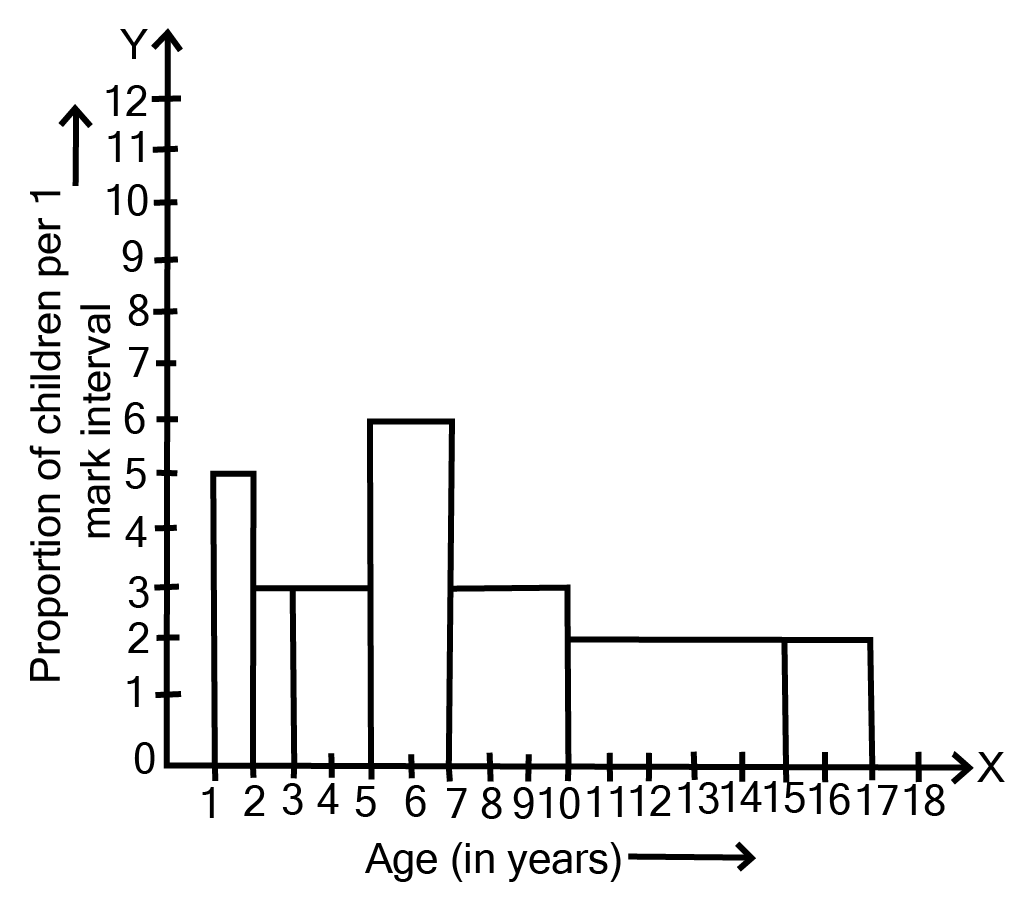
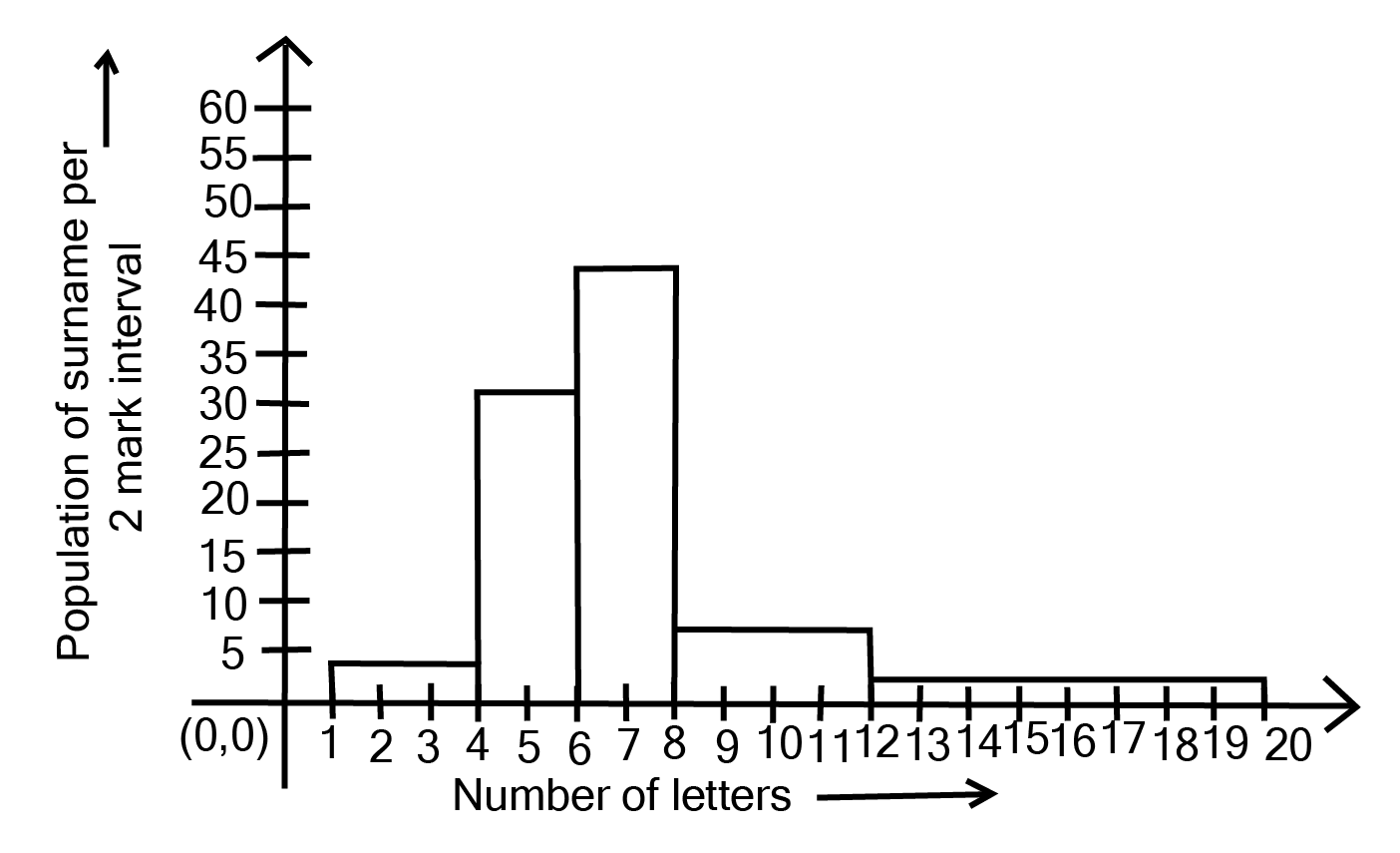
Exercise 12.1 focuses on the graphical representation of data, particularly histograms, frequency polygons, and cumulative frequency graphs. These graphical methods are crucial for visually interpreting and analyzing data.
9. Why are graphical representations of data important in Class 9 Maths Chapter Statistics Exercise 12.1?
Graphical representations are important because they:
Provide a visual summary of data.
Make it easier to identify patterns, trends, and outliers.
Facilitate comparison between different data sets.
10. What types of questions are commonly asked in exams from Class 9th Maths Chapter 12 Exercise 12.1?
Commonly asked questions in exams from Exercise 12.1 of Class 9 Maths Statistics include:
Drawing and interpreting histograms.
Constructing and analyzing frequency polygons.
Creating and using cumulative frequency graphs (ogives).
Finding statistical measures like median using graphical methods.














 Watch Video
Watch Video