NCERT Solutions for Maths Class 9 Chapter 5 Exercise 5.1 - FREE PDF Download
NCERT for Exercise 5.1 Class 9 Maths solutions Chapter 5 on Introduction to Euclid's Geometry by Vedantu, introduces valuable resources to solidify your understanding of fundamental concepts in Euclidean geometry. This exercise lays the groundwork for your journey through geometric shapes, their properties, and proofs. Recognizing points, lines, and planes in diagrams. Distinguishing between true and false statements related to geometric properties (e.g., only one line can pass through a single point).
This exercise focuses on foundational concepts in Euclidean geometry. With Vedantu's Class 9 Maths NCERT Solutions, you'll find step-by-step explanations of all the exercises in your textbook, ensuring that you understand the concepts thoroughly.
Glance on NCERT Solutions Class 9 Maths Chapter 5 Exercise 5.1 | Vedantu
A Point is a location in space without any dimensions. A point indicates a location in space. It has no length, width, or thickness. Example: A, B, C.
Line: A breadthless length that extends infinitely in both directions.
A Line Segment is a part of a line with two endpoints.
Ray is a part of a line that starts at one point and extends infinitely in one direction.
Plane: A flat, two-dimensional surface that extends infinitely in all directions.
A straight line segment can be drawn joining any two points.
A terminated line can be extended indefinitely.
NCERT Solutions for Maths Exercise 5.1 Class 9 Chapter 5 contains 7 Questions with Solutions.
1. Which of the following statements are true and which are false? give reasons for your answers.
(i) Only one line can pass through a single point.
Ans: False
Because an endless number of lines can pass through a single point \[\text{ }\!\!'\!\!\text{ P }\!\!'\!\!\text{ }\] below. There exists an infinite number of lines travelling through a single point \[\text{P}\], as shown in the diagram below.
(ii) There are an infinite number of lines which pass through two distinct points
Ans: False
Only one line can pass through two points. There is only one single line that can travel between two separate points \[\text{P}\] and \[\text{Q}\], as shown in the following diagram.
(iii) A terminated line can be produced indefinitely on both the sides
Ans: True
On both the sides, a terminated line can be produced indefinitely.
Assume that \[\text{AB}\] is a terminated line. It can be seen that it can be produced indefinitely on both the sides.
(iv) If two circles are equal, then their radii are equal.
Ans: True
If two circles are equal, then the centers and circumferences of the two circles are the same, the radii will be equal.
(v) In the following figure, if $\text{AM=PQ}$ and $\text{PQ=XY}$ ,then $\text{AB=XY}$
Ans: True
It is assumed that \[\text{AB}\] and \[\text{XY}\] are two terminated lines (Line segments) and that they are both equal to \[\text{PQ}\], a third line.
Euclid's first axiom stats that things which are equal to the same thing are equal to one another.
Therefore, the lines \[\text{AB=PQ}\] and \[\text{PQ=XY}\] , hence $AB=XY$ will be equal to each other.
2. Give a definition for each of the following terms. Are there other terms that need to be defined first ? what are they, and how might you define them ?
Parallel lines
Perpendicular lines
Line segment
Radius of a circle
Square
Ans:
The following terms are required for the desired definition:
Point:
A point can be approximated by a little dot formed with a sharp pencil on a sheet of paper.
A point does not have any dimensions; it simply has a position.
Line:
A straight line made by folding a piece of paper, a straight string pulled at both ends, and the edge of a ruler are all examples of geometrical lines.
The basic concept about a line is that it should be straight and that it should extend in definitely in both the direction
Plane:
Close examples of planes include the smooth surface of a wall or the smooth surface of a piece of paper.
Ray:
A ray\[\text{AB}\] is a segment of line l that has only one end point \[\text{A}\] and contains the point \[\text{B}\].
A point can be approximated by a little dot formed with a sharp pencil on a sheet of paper.
A point does not have any dimensions; it simply has a position.
A straight line made by folding a piece of paper, a straight string pulled at both ends, and the edge of a ruler are all examples of geometrical lines.
The fundamental principle of a line is that it should be straight and continue indefinitely in both directions.
Close examples of planes include the smooth surface of a wall or the smooth surface of a piece of paper.
A ray is a segment of line l that has only one end point and contains the point.
The union of two non-collinear rays with a common beginning point is called an angle .
Circle:
In a plane, a circle is the collection of all points whose distance from a fixed point is constant.
The fixed point is called the centre of the circle
Quadrilateral:
A closed figure made of four lines segment is called quadrilateral.
(i) Parallel lines
Ans:
Parallel lines are those in which the perpendicular distance between two lines is always the same.
To put it another way, parallel lines are lines that never cross one other.
To define parallel lines, we need to know about the point, the lines, and the distance between the lines and the intersection point.
(ii) Perpendicular lines
Ans:
If two lines intersect each other at \[\text{90 }\!\!{}^\circ\!\!\text{ }\] then these are called perpendicular lines.
Before defining perpendicular lines, we must first define the line and the angle.
(iii) Line segment
Ans:
A line segment is a straight line drawn from one point to another point.
To define a line segment, we must first understand what a point and a line segment are.
(iv) Radius of a circle
Ans:
It is distance between the center of a circle to any point lying on the circle.
We must understand point and circle in order to define the radius of a circle.
(v) Square
Ans:
A square is a quadrilateral with all sides equal in length and all angles measuring \[\text{90 }\!\!{}^\circ\!\!\text{ }\].
To define square, we must know about quadrilateral, side, and angle.
3. Consider the two 'postulates' given below:
Given any two distinct points A and B, there exists a third point C, which is between A and B
There exists at least three points that are not on the same line.Do these postulates contain any undefined terms ? Are these postulates consists ? Do they follow from Euclid's postulates\[\text{?}\]Explain.
Ans:
In the given postulates, there are several undefined terms.
Because the above postulates pertain to two different situations, they are consistent.
Furthermore, any assertion that contradicts a well-known axiom or postulate is impossible to infer.
The postulates of Euclid do not lead to these conclusions.
They follow from the axiom, "Given two distinct points, there is a unique line that passes through them"
4. If a point C lies between two points A and Bsuch that AC=BC then prove that \[\text{AC=}\frac{\text{1}}{\text{2}}\text{AB}\] Explain by drawing the figure.
Ans:
From the Figure,
Given that \[\text{AC=BC}\]
And point $C$ lies between two points \[\text{A}\] and \[\text{B}\]
Consider c
Adding \[\text{AC}\] on both sides we get
\[\text{AC+AC=BC+AC}\]
\[\Rightarrow \text{2AC=BC+AC}\]
Here we have \[\text{BC+AC=AB}\]
\[\Rightarrow \text{2AC=AB}\]
\[\Rightarrow \text{AC=}\frac{\text{1}}{\text{2}}\text{AB}\]
5. In above question, point C is called a midpoint of line segment AB, prove that every line segment has one and only mid point
Ans:
From the Figure,
Easier solution
Now to we will prove line \[\text{AB}\] has only one midpoint such that
Consider we have two midpoint \[\text{C}\] and \[\text{D}\] of line segment \[\text{AB}\]
Thus
\[\text{AD=DB}\] ......\[\left( \text{1} \right)\]
\[\text{AC = CB}\] ...... \[\left( \text{2} \right)\]
Now subtracting equation \[\left( \text{1} \right)\text{-}\left( \text{2} \right)\] we get
\[\text{AD - AC = DB - CB}\]
Using figure we have
\[\Rightarrow \text{CD = -DC}\]
\[\Rightarrow \text{2CD = 0}\]
\[\Rightarrow \text{CD = 0}\]
Therefore \[\text{C}\] and \[\text{D}\] coincides.
Hence required is proved.
Lengthy solution
\[\text{AC = CB}\] ...... \[\left( \text{1} \right)\]
Now adding \[\text{AC}\] on both sides of equation \[\left( \text{1} \right)\] we get
\[\text{AC+AC = AC+CB}\] ...... \[\left( \text{2} \right)\]
From the figure we have
\[\text{AC+CB=AB}\]
Now from equation \[\left( \text{2} \right)\] we have
\[\text{2AC = AB}\] ...... \[\left( \text{3} \right)\]
Similarly we have
\[\text{2AD = AB}\] ...... \[\left( \text{4} \right)\]
Now equalizing equation \[\left( \text{3} \right)\] and \[\left( \text{4} \right)\] we get
\[\text{2AC = 2AD }\]
\[\Rightarrow \text{AC = AD }\]
Therefore \[\text{C}\] and \[\text{D}\] coincides.
Hence required is proved.
6. In the following figure, if AC = BD, then prove that AB = CD
Ans:
We are asked to prove \[\text{AB = CD}\]
Let
\[\text{AB = CD}\] ...... \[\left( \text{1} \right)\]
Now adding \[\text{BC}\] on both sides of equation \[\left( \text{1} \right)\] we have
\[\text{AB+BC = CD+BC}\]
From the figure we have
\[\text{AC = BD}\] ...... \[\left( \text{2} \right)\]
Now from the figure we have
x\[\text{AC = AD - CD}\] ...... \[\left( \text{3} \right)\]
\[\text{BD = AD - AB}\] ...... \[\left( \text{4} \right)\]
Using above equation\[\left( \text{2} \right)\], \[\left( \text{3} \right)\] and \[\left( \text{4} \right)\] we have
\[\text{AD - CD = AD - AB}\]
\[\Rightarrow \text{- CD = - AB}\]
\[\Rightarrow \text{AB = CD}\]
Hence required is proved
7. Why is Axiom in the list of Euclid's axioms, considered a 'universal truth'\[\text{?}\](Note that the question is not about the fifth postulate.)
Ans:
Axiom $\text{5}$ states that the whole is greater than the part.
This axiom is known as a universal truth because it holds true because it holds true in any field, and not just in the field of mathematics
Let us take two cases one in the field of mathematics, and one other than that
Case one
Let $\text{t}$ represent a whole quantity and only $\text{a,b,c}$ are parts of it.
$\text{t = a + b + c}$
Clearly $\text{t}$ will be greater than all its parts $\text{a,b,c}$.
As a result, it is correct to say that the whole is greater than the part
Case two
Let us consider the continent Asia.
Now consider the country of India, which is located in Asia.
Although India is a part of Asia, it is also true that Asia is larger than India.
As a result, we might conclude that the whole is greater than the part.
This holds true in any corner of the world, making it a universal truth.
Conclusion
NCERT Solutions for Class 9 Math Exercise 5.1 Introduction to Euclid's Geometry is essential for building a strong foundation in geometry. Exercise 5.1 explores the definitions put forward by Euclid to give the students a clear understanding of the concept. This exercise lays the foundation for understanding geometric principles through the work of Euclid, an ancient Greek mathematician known as the "father of geometry". To excel in this topic, students should practice solving different types of problems and understand the underlying principles. Regular practice and thorough understanding will help students tackle any question related to comparing quantities confidently.
CBSE Class 9 Maths Chapter 5 Other Study Materials
Chapter-Specific NCERT Solutions for Class 9 Maths
Given below are the chapter-wise NCERT Solutions for Class 9 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
Important Study Materials for CBSE Class 9 Maths
FAQs on NCERT Solutions for Class 9 Maths Chapter 5 Introduction To Euclid'S Geometry Ex 5.1
1. What are parallel lines and perpendicular lines?
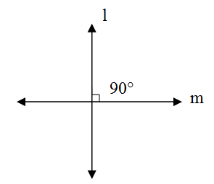
Parallel lines are a set of lines (two or more) on a plane that never meets. These lines do not intersect each other and are always at the same distance apart from each other. Perpendicular lines are lines that are at right angles to each other. If certain lines intersect each other in a plane at right angles, the lines are said to be perpendicular to each other. Students will be able to clearly understand the definitions of parallel lines and perpendicular lines by solving the 5th chapter of Class 9 Mathematics.
2. Where Can I find solved Exercise 5.1 of Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry?
Vedantu caters to NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry. Vedantu is an e-learning platform known to provide accurate NCERT Solutions to help students in clarifying their doubts. Class 9 Maths NCERT Solutions for Chapter 5 (Exercise 5.1) can be availed online or downloaded from Vedantu’s site. Vedantu provides a free PDF download of the solutions which include stepwise solutions to the exercise questions. These solutions are provided by subject matter experts and are designed as per the latest NCERT guidelines and syllabus.
3. Why should I refer to NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry Exercise 5.1?
NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclid’s Geometry is designed by Vedantu to ensure complete coverage of the exercise questions. These solutions are prepared by expert teachers as per the latest NCERT guidelines. Students must download it as it will allow them to solve the chapter effectively. All the important tips and tricks are given to solve the problems of the exercise. NCERT Solutions for Class 9 Maths for Chapter 5 Exercise 5.1 as well as other exercises are a great resource to score well in the Maths exam.
4. How to utilize NCERT Solutions for Class 9 Chapter 5 Introduction to Euclid’s Geometry to score well?
Students can go through exercise-wise NCERT Solutions for Class 9 Maths Chapter 5 as well as other chapters available on Vedantu. This will improve their understanding of the chapter. Students must try to solve all the problems given in the exercise and refer to the solutions in case of any doubts. This will save their time which students can utilize in the revision of the chapter. Students can also register for online interactive classes provided by experts at Vedantu and can learn all the necessary tips to score well in the exam.
5. What are Euclid's important axioms?
Euclid’s theorems remain the most important theorems in mathematics. The most important Euclid’s axioms are -
The whole is equal if the equals are added with equals.
The whole is always greater than the past.
The remainder is equals if the equals are subtracted from equals.
Many more of these important axioms are explained in the NCERT Solutions provided by Vedantu. These solutions help students solve questions given in the chapter Euclid’s Geometry.
6. How many questions do the exercise on Euclid’s Geometry dispense?
There are a total of seven questions that this exercise offers, ranging from problem-solving questions to the understanding of basic concepts of Geometry. For instance, questions based on understanding the difference between parallel lines, perpendicular lines, line segments, planes etc are given in this exercise. These exercises have detailed descriptions and answers. Students can get a better and easy understanding of the concepts by referring to these solutions provided by Vedantu.
7. What are the five postulates of Euclid’s Geometry?
There are five important postulates of Euclid’s Geometry. These postulates of Euclid’s Geometry include -
A straight line is usually drawn from one point to any other point.
Any centre and radius can be used to draw a circle.
All right angles are always equal.
A terminated line can be constructed indefinitely.
If a straight line falls on two straight lines and the interior angles of both sides of it taken together are less than two right angles, the two straight lines produced indefinitely meet on that side where the sum of angles is less than two right angles.
8. Why are NCERT Solutions for Chapter 5 “Euclid’s Geometry” of Class 9 preferable?
Vedantu’s NCERT Solutions are designed by subject matter experts. These solutions serve the curiosity of students and help clear doubts and confusions that may arise while solving the exercise. This is filled by the detailed explanations and answers that are given with each question, leaving no space for doubt in the student’s mind. Moreover, these questions are designed following the pattern of the CBSE curriculum exclusively, which explains the importance that is placed on procuring the most fundamental ideas before jumping into complex questions.
9. Why are practice questions important to score marks?
To score satisfactory marks in any examination, students need to have extensive practice in their field of study. This is because practice enhances one’s retaining powers that will help them in solving these questions quickly and effectively in exams. Moreover, it also enhances the existing skills and knowledge of the student. The NCERT Solutions are one such resource that a student can always turn to for the practice and understanding of the topics in their syllabus. These Solutions have numerous exercises that the student can work on to get a better grasp of the topic. To add the cherry on top, these exercises have detailed and explanatory answers helping students in every step of their learning and they are available at free of cost on the Vedantu website and on the Vedantu app.
10. What topics are covered in Class 9 Ch 5 Maths Ex 5.1?
Exercise 5.1 focuses on establishing a strong foundation in fundamental Euclidean geometry concepts. Here's a breakdown:
Identifying Basic Elements: Recognizing points, lines, and planes, the building blocks of geometry.
Understanding True/False Statements: Analyzing statements related to geometric properties and determining their validity (true or false).
Applying Geometric Definitions: Using precise definitions of terms like angles, rays, segments, and perpendicular lines to solve problems.
11. What is the focus of Introduction to Euclid's Geometry in Class 9th Maths Chapter 5 Exercise 5.1 Solution?
In class 9th maths chapter 5 exercise 5.1 solution focuses on the foundational concepts and principles of geometry introduced by Euclid. This includes basic definitions, axioms, and postulates that form the basis for geometric reasoning and proofs.
12. What are Euclid's definitions, and why are they important in Class 9 Maths Chapter 5 Exercise 5.1 Solutions?
In Class 9 Maths Ex 5.1 Euclid's definitions provide the basic terminology for geometric concepts, such as points, lines, line segments, rays, and planes. These definitions are essential for understanding and solving geometric problems.
13. How does Ex 5.1 Class 9 help in understanding Euclid's Geometry?
Class 9 Maths Chapter 5 Exercise 5.1 Solutions includes questions that test the comprehension of basic definitions, axioms, and postulates introduced by Euclid. It helps students understand and apply these fundamental concepts through various problems and examples.
