NCERT Solutions for Class 9 Maths Chapter 8 Quadrilaterals Exercise 8.2 - FREE PDF Download
NCERT Solutions for Class 9 Maths Chapter 8 Quadrilaterals Ex 8.2
FAQs on NCERT Solutions for Class 9 Maths Chapter 8 Quadrilaterals Ex 8.2
1. Where do we use Quadrilaterals in real life?
Quadrilaterals are used in electronic devices like mobiles, laptops, computers, TVs, etc.In stationery items like books, copies, chart-papers, etc. The list goes countless as we can't imagine the world without quadrilaterals. Wherever you see four sides, the quadrilateral is involved there.
A quadrilateral is a four-sided polygon which has four angles. Which includes square, trapezium, parallelogram, kite, etc within it. Quadrilaterals play a very special role in the work of architects as it helps them to design any building by making effective utilization of space. Quadrilaterals are the second most popular shape used in architectural designs.
2. What are the properties of the quadrilateral?
There are two properties of quadrilaterals: A quadrilateral should be closed shape with 4 sides. All the internal angles of a quadrilateral sum up to 360°
Parallelogram:
A quadrilateral satisfying the below-mentioned properties will be classified as a parallelogram. A parallelogram has four properties:
Opposite angles are equal.
Opposite sides are equal and parallel.
Diagonals bisect each other.
Sum of any two adjacent angles is 180°
Rhombus:
A rhombus is also a quadrilateral which has the following four properties:
Opposite angles are equal
All sides are equal and, opposite sides are parallel to each other
Diagonals bisect each other perpendicularly
Sum of any two adjacent angles is 180°
3. How many questions are there in exercise 8.2?
There are a total of 7 questions in this exercise. In this exercise, question 1 is based on the quadrilateral of a parallelogram. In which, you have to prove the given three statements. Question 2 is based on rhombus, in which you have to prove that the given quadrilateral is a rectangle. Question 3 asks you to prove the given quadrilateral is a rhombus.
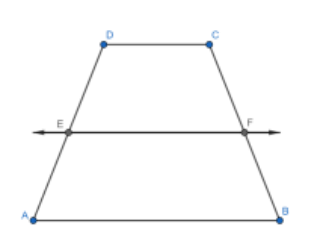
Question 4 is a figure based question, in which, you have to prove one point is the midpoint of a line in the mentioned trapezium. Question 5 is based on a parallelogram, where you need to show that the trisection of two lines is through a diagonal line. In question 6 you have to show the line segment joining the midpoint of a quadrilateral bisect each other. Question 7 is based on the right-angled triangle, where you need to prove all the three given statements.
4. Why should I choose Vedantu for preparation?
All our NCERT solutions are formulated by our experienced faculty and they have covered every part of the chapter along with the exercise questions which are stated at the end of the chapter. These comprehensive solutions to the questions are to the point which will make you understand the chapter and this will improve your score in the examination.
All the chapters are explained in detail to make it more simple and clear for you. We make sure that all the topics and sub-topics are covered from every chapter and we also have designed these solutions in such a way to make your learning process more fun, fascinating and enjoyable. Learning from here will make your revision task simpler.
5. What are the Key Features of Vedantu NCERT Solutions for Exercise 8.2 of Chapter 8 of Class 9 Maths?
The key features of using Vedantu NCERT Solutions for Exercise 8.2 of Chapter 8 of Class 9 Maths are:
NCERT solutions have been framed by expert teachers in a logical and simple language.
A pictorial presentation is provided for all the questions making it easier for the students to understand.
Our aim is to make learning fun in the form of activity-based and knowledge-driven.
All the solutions are explained in a detailed and well-organised way.
Step by step format is used to solve all NCERT questions to help the students understand the methods effortlessly.
6. How do Vedantu NCERT Solutions for Exercise 8.2 of Chapter 8 of Class 9 Maths help the students in preparing for CBSE exams?
The NCERT Solutions provided by the Vedantu will help the students in their exam preparation as it will help them to improve their foundation in topics like mid-point theorem. These solutions will help the students to speed up their exam preparation and save time. Students can refer to these to verify their answers and steps are right or wrong. All the solutions are accurate and are explained in a detailed way helping the students to score high marks. It will help in boosting the confidence level among students and increase the efficiency to solve difficult problems in a shorter duration.
7. Can I download the PDF of NCERT Solutions for Exercise 8.2 of Chapter 8 of Class 9 Maths for free?
Yes, Students can download the PDF of NCERT Solutions for Exercise 8.2 of Chapter 8 of Class 9 Maths for free from the Vedantu study portal. It helps the students boost their exam preparation. All the solutions are designed in a step by step manner by the maths experts and are strictly based on the textbook prescribed by the CBSE board. These solutions will help the students to improve their problem-solving skills which are very important for the exam. The solutions are also available on the Vedantu Mobile app.
8. Are the NCERT solutions for Exercise 8.2 of Chapter 8 of Class 9 Maths important from the exam point of view?
Yes. To score high marks in the board exams, all the questions from the CExercise 8.2 of Chapter 8 of Class 9 Maths are important. So, students must practise all the questions from the exercise without skipping any questions. These stepwise solutions provided by the Vedantu are very accurate and are detailed. It will help the students to understand the concepts, methods and analyse the types of questions that would appear in the exam.
9. Do we need to practice extra questions for Exercise 8.2 of Chapter 8 of Class 9 Maths?
There is no need to practice extra questions for the Exercise 8.2 of Chapter 8 of Class 9 Maths from resources other than the NCERT Solutions by Vedantu. The NCERT textbook questions and Exemplar questions as well as examples from the book are sufficient to practice. It depends on the student to practice extra questions or not though it is not mandatory. Students can refer to NCERT solutions to clear their doubts instantly.
10. What topics are covered in class 9th maths exercise 8.2?
Exercise 8.2 focuses on the properties and criteria of parallelograms. It includes identifying the conditions that make a quadrilateral a parallelogram, such as opposite sides being equal and opposite angles being equal. The exercise also explores theorems related to parallelograms, such as the diagonals bisecting each other. Understanding these properties is essential for solving various geometric problems. Additionally, it covers the application of these properties in different scenarios and problem-solving exercises.
11. How do you prove a quadrilateral is a parallelogram in exercise 8.2 class 9th?
You can prove a quadrilateral is a parallelogram by demonstrating that both pairs of opposite sides are equal. Another method is to show that one pair of opposite sides is both equal and parallel. You can also prove it by showing that the diagonals bisect each other. Each of these criteria can be used independently to establish that a quadrilateral is a parallelogram. Using geometric proofs and logical reasoning is crucial in this process. Practicing these proofs helps strengthen your understanding of geometric properties.
12. What is the importance of proving theorems in class 9 quadrilateral solutions?
Proving theorems helps solidify understanding of geometric properties and improves problem-solving skills. It builds a strong foundation for advanced mathematical concepts. Additionally, it enhances logical reasoning and analytical thinking abilities.
13. How many questions from class 9 maths 8.2 solutions were asked in previous year exams?
Two questions from Quadrilateral Class 9 Ex 8.2 appeared in the previous year's exams. These questions often test understanding of key properties of quadrilaterals. Practicing these ensures better exam preparation and confidence.
14. Why is the Midpoint Theorem important for class 9 ex 8.2 maths solutions?
The Midpoint Theorem helps to prove that a line segment joining the midpoints of two sides of a triangle is parallel to the third side and half its length. This theorem is crucial for solving many geometric problems. It simplifies complex proofs and enhances comprehension of triangle properties.














 Watch Video
Watch Video