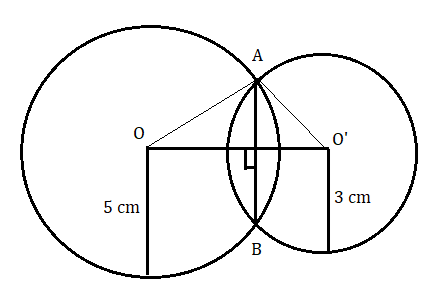
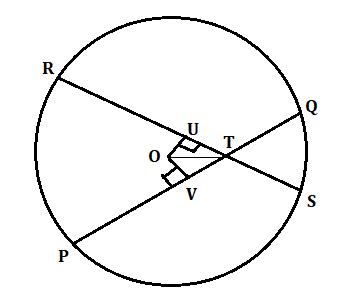
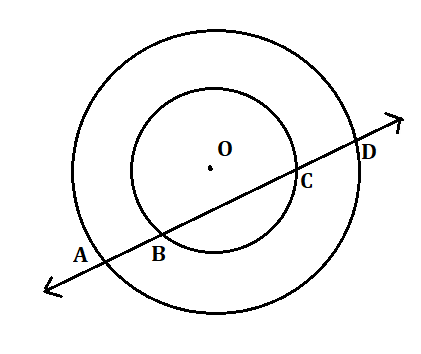
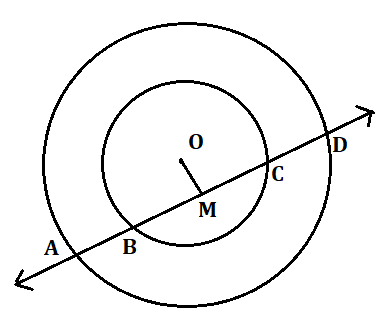
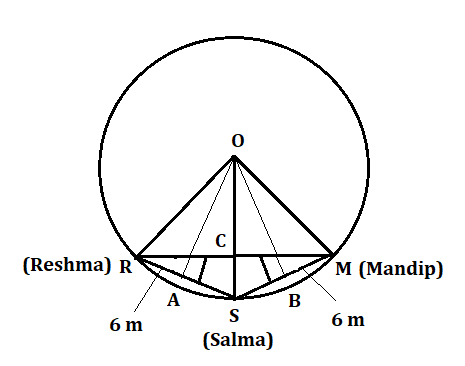
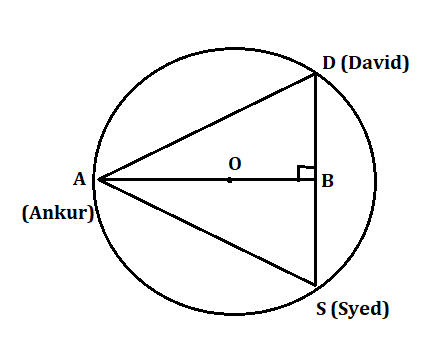
NCERT Solutions for Circles Class 9 Maths Chapter 9 Exercise 9.2 - FREE PDF Download
NCERT Solutions for Class 9 Maths Chapter 9 Circles Ex 9.2
FAQs on NCERT Solutions for Class 9 Maths Chapter 9 Circles Ex 9.2
1. Give me an overview of the topics and sub-topics from NCERT Class 9 Maths Ex 9.2.
NCERT Solutions for Chapter 9 titled Circles Class 9 Maths Ex 9.2 is available in PDF format, which students can download easily. These problems have been solved by our in house experts from the respective background, keeping the student's understanding level in mind. All the solutions for Class 9th Maths subjects are created as per the latest NCERT syllabus and guidelines, which proved to be beneficial for the students to score the best possible marks.
The topics and sub-topics included in NCERT Textbook Class 9 Maths Ex 9.2 Circles are:
Circles
Introduction
Circles And Its Related Terms: A Review
Angle Subtended By A Chord At A Point
Perpendicular From The Centre To A Chord
Circle Through Three Points
Equal Chords And Their Distances From The Centre
Angle Subtended By An Arc Of A Circle
Cyclic Quadrilaterals
Summar
2. How many questions are there in Class 9 Maths Ch 9 Ex 9.2?
NCERT Solutions for Class 9 Maths Ch 9 Ex 9.2 consists of six questions in total. Answers of all these questions are provided accurately in the solutions which are available on Vedantu website PDF format. Any Class 9 student can download the Maths NCERT Solutions for self-study purpose as per their convenience.
3. What are the benefits of referring to Class 9 Maths Ch 9 Ex 9.2 NCERT Solutions?
NCERT Solutions for Class 9 Maths Ch 9 Ex 9.2 Circles are provided in PDF format, which can be easily downloaded for absolutely free of cost. The solutions for the chapter have been solved by our subject-matter experts and teachers as per the CBSE curriculum with 100 percent accuracy. These solutions serve as the important study material for the Class 9 students. There are multiple benefits for using it.
These are -
Class 9 Maths 9.2 solutions are written in a concise manner and easy-to-understand language, considering the understanding ability of every kind of the students.
All the answers in the solutions are updated as per the latest NCERT (CBSE) curriculum and guidelines. These cover all the questions asked in the exercises of the entire syllabus.
If there is any kind of complex question, it is broken down into simple and easy steps to make it understandable for every student so that they can take command over the concept in less time.
All the answers are given in a step-by-step manner.
The content is designed in such a manner which is brief and self-explanatory.
Some answers include necessary diagrams and infographics to make the concepts easy to understand.
The NCERT Solutions are handy and serve as a good study material during revision prior to exam.
Class 9 Maths 9.2 solutions are designed in such a way that it improves the problem-solving speed of every student. So that they can solve problems in the exam hall long before the stipulated time.
4. Can I download Class 9 Maths Ch 9 Ex 9.2 NCERT Solutions for free?
Yes, you can easily download the Class 9 Maths 9.2 NCERT Solutions for absolutely free of cost. These are available on our website in PDF format.
5. How can I save Chapter 9 Of Class 9 Maths 9.2 solutions for later use?
To save Vedantu’s NCERT Solutions for Class 9 Maths Exercise 9.2.
Visit NCERT Chapter 9 of Class 9.
Scroll down and find the exercise for which you want the solutions.
Click on the link for the exercise PDF
The solutions PDF will be displayed on your screen. Click on the 'Download PDF' option to save the solutions for offline use.
6. Does Vedantu provide answers to all the questions of Class 9 Maths Exercise 9.2?
The NCERT Solutions by Vedantu are created as per the latest syllabus, taking into account the requirements of the students and the CBSE. Vedantu experts works on each solution with care making sure it is easy to understand, making sure that each and every question is answered accurately. Class 9 Maths Exercise 9.2 contains a total of six questions. Every question mentioned in the NCERT textbook is explained in detail for the ease of the students.
7. Does Vedantu’s NCERT Solutions for Exercise 9.2 Class 9 Maths provide detailed answers to the questions?
Yes, every question in Vedantu’s NCERT Solutions ex 9.2 Class 9 Maths mentions the complete details of the answer, so that students can easily understand the solution. Our experts make sure that the answer is not only explained in detail but also contains relative diagrams and representations wherever required. Vedantu’s experienced Mathematics teachers also ensure that the answers are composed in the most straightforward way to make it easy for students to comprehend the solutions.
8. Vedantu’s NCERT Solutions for Exercise 9.2 Class 9 Maths are suitable for exam preparation.
Chapter 9 Circles is an important chapter from an examination standpoint. The NCERT Solutions for Class 9th Exercise 9.2 offer accurate solutions for exams. These solutions are created based on CBSE guidelines and hence are ideal for CBSE exams. The solutions to these questions are prepared in a way to teach the students the right way to provide step-by-step answers for every question in their exams.
9. Is it necessary to practice all the questions mentioned in the NCERT textbook for 9th Class Maths 9.2 Exercise Solutions?
Yes, every question in the NCERT textbook should be practised thoroughly. Exercise 9.2 is based on the following two important theorems:
Equal chords of a circle (or of congruent circles) are equidistant from the centre (or centres).
Chords equidistant from the centre of a circle are equal in length.
Hence, all questions must be practised diligently. You may refer to Vedantu’s Solutions for Exercise 9.2 of Chapter 9 Of Class 9 Maths.
10. What are tangents in a circle, and how do they relate to geometry in 9th Class Maths 9.2 Exercise Solutions?
Tangents are lines that touch a circle at exactly one point. In geometry, they help determine angles formed by intersecting lines inside and outside the circle. Understanding tangents is essential for calculating how lines interact with circles and for solving practical problems involving circle geometry.
11. How is the radius of a circle different from its diameter in 9th Class Maths 9.2 Exercise Solutions?
In 9th Class Maths 9.2 Exercise Solutions, the radius of a circle is the distance from its centre to any point on its edge, while the diameter is the distance across the circle passing through its centre. The radius is half of the diameter, and both measurements are crucial for calculating the circle's circumference and area.
12. What is the formula for calculating the circumference of a circle, and why is it important in Class 9th Exercise 9.2?
In Class 9th Exercise 9.2, the circumference of a circle is calculated using the formula C=2πr, where 𝑟 is the radius. This formula helps determine the total length around the circle's edge. It is fundamental in various applications, such as designing circular structures, calculating distances, and understanding rotational motion in physics and engineering.














 Watch Video
Watch Video