Courses

Courses for Kids

Free study material

Store

Talk to our experts

1800-120-456-456

Optics Chapter - Physics JEE Main

JEE Main

Physics

Optics

Last updated date: 24th Apr 2024

•

Total views: 64.2k

•

Views today: 0.64k

Concept of Optics for JEE Main Physics

Optics is like the magic of light and how it interacts with the world. It helps us understand how we see things, how lenses work, and how rainbows form. Imagine it as the science of understanding light and its tricks, like a magician revealing the secrets behind the illusions. In your JEE studies, optics is an essential topic that helps you see the world through the eyes of science.

JEE Main Physics covers a wide range of topics in optics, including:

Ray optics: Ray optics is a geometrical approach to understanding the propagation of light. It is based on the principle that light travels in straight lines, and that it can be reflected and refracted at interfaces between different materials.
Wave optics: Wave optics is a physical approach to understanding the propagation of light. It is based on the principle that light is a wave, and that it can interfere and diffract.
Modern optics: Modern optics is a branch of optics that deals with the interaction of light with matter at the atomic and subatomic level. It includes topics such as lasers, fiber optics, and holography.

Now, let's move on to the important concepts and formulae related to JEE and JEE Main exams along with a few solved examples.

JEE Main Physics Chapters 2024

JEE Main Physics Chapters
1	Units and Measurement	11	Electrostatics
2	Kinematics	12	Current Electricity
3	Laws of Motion	13	Magnetic Effects of Current and Magnetism
4	Work, Energy and Power	14	Electromagnetic Induction and Alternating Currents
5	Rotational Motion	15	Electromagnetic Waves
6	Gravitation	16	Optics
7	Properties of Solids and Liquids	17	Dual Nature of Matter
8	Thermodynamics	18	Atoms and Nuclei
9	Kinetic Theory of Gases	19	Electronic Devices
10	Oscillations and Waves	20	Communication Systems

Important Topics of Optics Chapter

Reflection of light
Refraction of light
Dispersion of light
Optical instruments
Huygens principle and interference of light
Diffraction and polarisation of light

Optics Important Concepts for JEE Main

Name of the Concept	Key Points of the Concepts
1. Reflection of light	The phenomenon of light reflection is the change in the course of light without any change in the medium. The mirror formula is a relationship between the mirror's focal length and the distance between the object and image from the mirror. Magnification is defined as the ratio of the size of the image formed by the mirror to the size of the object.
2. Refraction of light	Refraction of light is the phenomenon of change in the path of light when it goes from one medium to another. The basic cause of refraction is change in the velocity of light when it goes from one medium to the other. The phenomenon of total internal reflection is the reflection of light into a denser medium from an interface of this denser medium and a rarer medium. Spherical reflecting surfaces is a part of a sphere of transparent medium and these are of two types: Convex spherical refracting surface, which is convex towards the rarer medium side. Concave spherical refracting surface, which is concave towards the rarer medium side. A lens is a section of a transparent refracting material that is joined by two spherical surfaces or one spherical surface and one plane surface. There are types of lenses: Converging or Convex lens Diverging or Concave lens Lens maker’s formula is a relation that connects focal length($f$) of the lens to radii of curvature ($R_1$ and $R_2$) of the two surfaces of the lens and refractive index($\mu$) of the material of the lens. It is written as $\dfrac{1}{f}=(\mu-1)(\dfrac{1}{R_1}-\dfrac{1}{R_2})$ Power of a lens ($P$) is defined as the ability of the lens to converge a beam of light falling on the lens and it is measured as $P=\dfrac{1}{f}$ Combination of Thin Lenses in Contact: When two or more thin lenses are in contact, they can be treated as a single lens. The power of the combination is the sum of the individual powers of the lenses.
3. Dispersion of light	The phenomenon of light dispersion is the splitting of a white light beam into its constituent colours when it passes through a prism. The ratio of angular dispersion to the mean deviation generated by a prism is known as its dispersive power. The dispersive power of a prism is solely determined by the type of prism material. However, angular dispersion and mean deviation, both depend on angle of prism in addition to the nature of material of the prism.
4. Deviation and Dispersion of Light by a Prism	A prism is a triangular optical element that refracts light, causing it to deviate. The deviation is different for different colors due to dispersion, and this phenomenon is key in understanding how rainbows form and how prisms split white light into its constituent colors.
5. Optical instruments	A simple microscope is used for observing magnified images of tiny objects and it consists of a converging lens of small focal length. Magnifying power ($m$) of a simple microscope is defined as the ratio of angles subtended by the image and the object on the eye, when both are at the least distance of distinct vision($d$) from the eye. It is given as $m=(1+\dfrac{d}{f})$ Compound microscope consists of two lenses co-axially at the free end of a tube, at a suitable fixed distance from each other. The magnifying power of the compound microscope is given as $m=\dfrac{L}{\|f_o\|}(1+\dfrac{d}{f_e})$ Here, $f_o$= focal length of objective lens, $f_e$= focal length of the eye-piece, $L$= length of the microscope tube and $d$= least distance of distinct vision. Astronomical telescope consists of two lenses i.e, an objective lens which is of large focal length($f_o$) and an eyepiece which has a small focal length($f_e$). The astronomical telescope's magnification power is given as $m=\dfrac{f_o}{-f_e}$ Reflecting type of telescope consists of a parabolic concave reflector with a narrow hole at the centre and secondary convex mirror. In normal adjustment, magnifying power of the a reflecting type telescope is given as $m=\dfrac{R/2}{f_e}$ Here, $R$= radius of curvature of concave reflector and $f_e$ = focal length of the eyepiece Refracting Telescope employs lenses to bend and focus light. The Keplerian telescope is a well-known example.
6. Huygens principle and interference of light	The continuous location of all particles of a material vibrating in the same phase is characterised as a wave front. According to Huygen’s principle, Every particle on the given wavefront acts as a fresh source of new disturbance, called secondary wavelets, which travels in all directions with the velocity of light in the medium. At any instant, a surface encountering these secondary wavelets tangentially in the forward direction yields the new wavefront at that instant. This is referred to as a secondary wave front. The process of redistribution of light energy in a medium caused by the superposition of light waves from two coherent sources is known as interference of light. Condition for constructive interference: $x=n\lambda$ Here, $x$= path difference between two waves, $\lambda$= wavelength of light and $n$= 0,1,2…….. Condition for destructive interference: $x=\dfrac{(2n-1)\lambda}{2}$ Here, $n$ = 1,2,3…………. In Young’s double slit experiment, The condition for bright fringe is given as $x=n\lambda(\dfrac{D}{d})$ Here, $n$ = 0,1,2,3……… The condition for dark fringe is $x=(2n-1)\dfrac{\lambda}{2}\dfrac{D}{d}$ Here, $D$= distance between slit and screen, $d$ = distance between two slits and $n$ = 1,2,3………. The expression of fringe width ($\beta$) is $\beta=\dfrac{\lambda D}{d}$
7. Diffraction and polarisation of light	The phenomenon of light bending around corners of a barrier or aperture in its path is known as diffraction. The $n^{th}$ secondary minima is given as $\sin\theta_n=\dfrac{n\lambda}{a}$ The $n^{th}$ secondary maxima is given as $\sin\theta_n=\dfrac{(2n+1)\lambda}{2a}$ Here, $n$ = 1,2,3……an integer, $a$ = width of the aperture, $\theta$ = angle made by secondary waves while travelling and $\lambda$ = wavelength of light. The phenomenon of restricting the vibration of light in a particular direction, perpendicular to the direction of the wave motion, is called polarisation of light. According to the Law of Malus, when a beam of completely polarised light is incident on an analyser, the resultant intensity of light ($I$) transmitted from the analyser varies directly as the square of the cosine of the angle ($\theta$) between plane of transmission of analyser and polariser.
8. Coherent Sources and Sustained Interference	For interference to occur, the sources of light must be coherent, meaning they have a constant phase relationship. Coherent sources are essential for sustained interference patterns. These patterns play a crucial role in applications such as interferometry and the study of thin films.
9. Diffraction and the Width of Central Maximum	Diffraction is a phenomenon where light waves bend around obstacles and spread out. When light passes through a single slit, it diffracts, leading to the formation of a diffraction pattern. The width of the central maximum in a single-slit diffraction pattern can be determined using the formula: W= 2λL/w Where: λ is the wavelength of light. L is the distance from the slit to the screen. w is the width of the slit.
10. Resolving Power of Microscopes and Astronomical Telescopes	The resolving power of optical instruments like microscopes and telescopes is a critical factor. It determines their ability to distinguish two closely spaced objects. The resolving power of a microscope or a telescope can be defined as: R= 1.22λ/D Where: λ is the wavelength of light. D is the diameter of the objective lens or mirror.
11. Polarization and Brewster's Law	Polarization is the phenomenon where light waves vibrate in a specific direction. Brewster's Law helps us understand the angle at which polarized light is reflected from a surface. It is expressed as: $\tan \theta_B = \dfrac{n_2}{n_1}$ Where: $\theta_B$ is the Brewster angle. n₁ and n₂ are the refractive indices of the two mediums.
12. Uses of Plane-Polarized Light and Polaroids	Polarized light has numerous applications, including glare reduction, 3D cinema, and stress analysis. Polaroid filters, which selectively transmit polarized light, are commonly used to achieve these effects.

List of Important Formulas for Optics Chapter

S.No.	Name of the Concept	Formula
	Reflection of light	Mirror formula: $\frac{1}{v}+\frac{1}{u}=\frac{1}{f}$ Linear magnification of a spherical mirror $m=-\dfrac{v}{u}$
	Refraction of light	Snell’s Law: $\frac{\mu_2}{\mu_1}=\frac{\sin i}{\sin r}$ Here, $\mu_1$ and $\mu_1$ are the refractive indices of the medium 1 and medium 2. Total internal reflection: $\mu^a_w=\frac{1}{\sin C}$ Lens maker’s formula: $\frac{1}{f}=(\mu-1)(\frac{1}{R_1}-\frac{1}{R_2})$ Magnification for concave lens: $m=\frac{v}{u}$ Equivalent focal length ($F$) of two lenses having focal lengths $f_1$, $f_2$ and separated by a distance $d$ is $\frac{1}{F}=\frac{1}{f_1}+\frac{1}{f_2}-\frac{d}{f_1f_2}$
	Dispersion of light	Refractive index of prism is $\mu=\frac{\sin(A+\delta_m/2)}{\sin (A/2)}$ Here, $A$ = angle of prism and $\delta_m$ = minimum deviation angle. Dispersive power ($\omega$) is given as $\omega=\frac{d\mu}{\mu-1}$ Here, $d\mu$ = difference in refractive indices of prism for violet and red colour.
	Optical instruments	A simple microscope's magnification power ($m$) equals to $m=(1+\frac{d}{f})$ The magnifying power of compound microscope is $m=\frac{L}{\|f_o\|}(1+\frac{d}{f_e})$ Magnifying power of astronomical telescope is $m=\frac{f_o}{-f_e}$ Magnifying power of the a reflecting type telescope is $m=\frac{R/2}{f_e}$
	Huygens Principle and interference of light	In Young’s double slit experiment, the condition for bright fringe is given as $x=n\lambda(\frac{D}{d})$ Here, $n$ = 0,1,2,3……… The condition for dark fringe is $x=(2n-1)\frac{\lambda}{2}\frac{D}{d}$ Here, $n$ = 1,2,3………. Fringe width ($\beta$) is $\beta=\frac{\lambda D}{d}$
6.	Diffraction and polarisation of light	The $n^{th}$ secondary minima in diffraction is $\sin\theta_n=\frac{n\lambda}{a}$ The $n^{th}$ secondary maxima in diffraction is $\sin\theta_n=\frac{(2n+1)\lambda}{2a}$ Law of Malus is $I\varpropto\cos^2\theta$

JEE Main Optics Solved Examples

1. When a glass plate with a refractive index of 1.3 is put in the path of one of the beams in Young's double slit experiment, the fringes are displaced by a distance $x$. The fringe shift is $(5/2)x$ when this plate is replaced by another plate of the same thickness. The second plate's refractive index will be_______.

Sol:

Given:

Refractive index of glass plate, $\mu_1 = 1.3$

Shift in the fringes is $(5/2)x$.

To find, $\mu_2 = ?$

To solve this problem, we have to use the relation between the thickness of a plate, path difference and its refractive index. Also, we have to use the relation of fringe width.

The relation between the path difference($\Delta$), the thickness of the plate ($t$) and its refractive index ($\mu$) is given as

$\Delta=(\mu-1)t$

The path difference of $\lambda$ has introduced the phase difference of $\beta$. Therefore, a path difference of $(\mu-1)t$ that will introduce a shift of $x$ is given as

$x=\frac{(\mu-1)t\beta}{\lambda}$

In the case of the first plate, we can write:

$x=\frac{(\mu_1-1)t\beta}{\lambda}$.........(1)

In the case of the second plate, we can write:

$\dfrac{5x}{2}=\frac{(\mu_2-1)t\beta}{\lambda}$.........(2)

After dividing eq. (2) by (1), we get:

$\frac{(\mu_2-1)}{(\mu_1-1)}=\frac{5}{2}$

On further solving, we get:

$\mu_2-1 = \frac{5}{2} (\mu_1-1)$

Now, after putting the value of $\mu_1$, we get:

$\mu_2-1 = \frac{5}{2} (1.3-1)$

$\mu_2-1 =2.5 \times 0.3$

$\mu_2-1 = 0.75$

$\mu_2=0.75+1=1.75$

Therefore, the refractive index of the second plate is 1.75.

Key point: The knowledge of the concept of path difference is essential for solving such problems.

2. The slits in Young's double-slit experiment are spaced by 0.3 mm, and the screen is 130 cm distant. To obtain interference fringes on the screen, a laser beam with two wavelengths, 600 nm and 500 nm, is utilised. Calculate the shortest distance between the shared centre maxima and the point where the bright fringes caused by both wavelengths coincide.

Sol:

Given:

Distance between the slits, $d=0.3\,mm=0.3\times 10^{-3}\,m$

Wavelengths of the laser, $\lambda_1=600\,nm=600 \times 10^{-9}\,m$

And, $\lambda_2=500\,nm=500 \times 10^{-9}\,m$

Distance between slits and screen, $D=130\,cm=1.3\,m$

In order to solve this problem, we have to apply the concept of central maxima in interference pattern. Let $x$ be the distance between the centre maxima and the point at which the bright fringes caused by both wavelengths coincide.

Now for $\lambda_1$, we can write the condition for bright fringe as

$x=\frac{n\lambda_1 D}{d}$......(3)

Similarly, for for $\lambda_2$

$x=\frac{m\lambda_2 D}{d}$........(4)

After dividing the equations (1) and (2), we get:

$\frac{m}{n}=\frac{\lambda_1}{\lambda_2}$

$\frac{m}{n}=\frac{600 \times 10^{-9}}{500 \times 10^{-9}}$

$\frac{m}{n}=\dfrac{6}{5}$

From the above relation, we can conclude that the central maxima of $5^{th}$ bright fringe of $\lambda_1$ coincides with the $6^{th}$ bright fringe of $\lambda_2$.

Now, using equation (3), we can find the value of $x$ as

$x=\frac{n\lambda_1 D}{d}$

$x=\frac{5\times 600 \times 10^{-9} \times 1.3 }{0.3\times 10^{-3}}$

$x= 13000 \times 10^{-6}\,m=0.13\,mm$

Therefore, the shortest distance will be 0.13 mm.

Key point: The concept of interference of light and its conditions for bright fringes is important to solve this problem.

Previous Years’ Questions from JEE Paper

1. A beam of white light is incident on the glass air interface from glass to air such that green light just suffers total internal reflection. The colours of the light which will come out to air are

violet, indigo, blue
all colours except green
yellow, orange, red
white light

Sol:

According to the question, it is given that white light is incident on the glass air interface from glass to air and green light suffers total internal reflection. We know that white light consists of seven bands of colours i.e, VIBGYOR whose wavelength increases as we go from violet to red colour.

Therefore, the wavelengths of yellow, orange and red colour light are greater than the wavelength of green colour light, while the wavelengths of violet, indigo and blue are lesser than green colour light.

The refractive index relation under total internal reflection is given as

$\mu=\frac{1}{\sin C}$

And as $\mu \varpropto \frac{1}{\lambda}$

We can conclude that

$\sin C \varpropto \lambda$

Hence, $C$ will be greater for yellow, orange and red colour light. Therefore, these colour lights will come into the air from the glass.

Key point: The concept of total internal reflection and the relation between refractive index and wavelength of light are the important keys to solve this problem.

2.A beam of light consisting of red, green and blue colours is incident on a right angled prism, as shown in figure. The refractive indices of the material of the prism for the above red, green and blue wavelengths are 1.39, 1.44 and 1.47, respectively. The prism will

a. separate part of the red colour from the green and blue colours.

b. separate part of the blue colour from the red and green colours.

c. separate all the three colours from one another.

d. not separate even partially any colour from the other two colours.

Sol:

Let’s consider that critical angle is $C$ and it is equal to $45^\circ$.

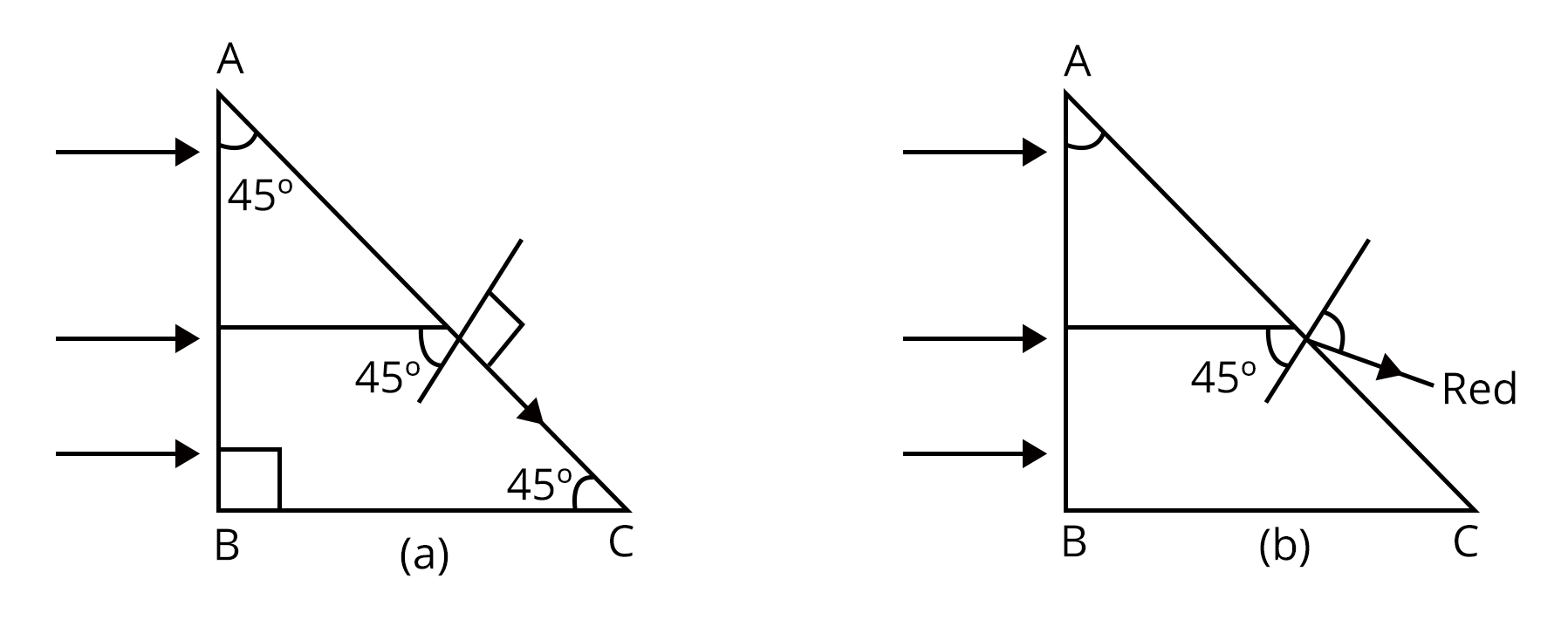
Using the concept of total internal reflection, we can write the refractive index as

$\mu=\frac{1}{\sin C}$

$\mu=\frac{1}{\sin 45^\circ}$

$\mu= 1.414$

It is given that the rays are falling normally on face AB. If the angle of incidence is greater than C, then the rays will suffer total internal reflection. Thus, if $\mu$ is greater than 1.414 then the colour will suffer total internal reflection.

For red colour, the refractive index is 1.39 which is less than 1.414 but for the green and blue colurs, the refractive indices are 1.44 and 1.47, which are more than the 1.414. Hence, red colour will emerge out of the prism while green and blue colours suffer total internal reflection. Hence, option a is correct.

Key point: The phenomena of total internal reflection is the crucial key to solve this problem.

Practice Questions

1. In a single slit diffraction experiment, the first minimum for red light (660 nm) coincides with the first maximum for some other wavelength $\lambda$. Calculate $\lambda$.

Ans: 440 nm

2. Find the minimum thickness of a film which will strongly reflect the light of wavelength 589 nm. The refractive index of the material of the film is 1.25.

Ans: 118 nm

JEE Main Physics Optics Study Materials

Here, you'll find a comprehensive collection of study resources for Optics designed to help you excel in your JEE Main preparation. These materials cover various topics, providing you with a range of valuable content to support your studies. Simply click on the links below to access the study materials of Optics and enhance your preparation for this challenging exam.

JEE Main Optics Study Materials

JEE Main Optics Notes

JEE Main Optics Important Questions

JEE Main Optics Practice Paper

JEE Main Physics Study and Practice Materials

Explore an array of resources in the JEE Main Physics Study and Practice Materials section. Our practice materials offer a wide variety of questions, comprehensive solutions, and a realistic test experience to elevate your preparation for the JEE Main exam. These tools are indispensable for self-assessment, boosting confidence, and refining problem-solving abilities, guaranteeing your readiness for the test. Explore the links below to enrich your Physics preparation.

JEE Main Physics Study and Practice Materials

JEE Main Physics Previous Year Question Papers

JEE Main Physics Mock Test

JEE Main Physics Formula

JEE Main Sample Paper

JEE Main Physics Difference Between

Conclusion

In this article, we'll dive into the fascinating world of optics, a crucial chapter in JEE Main physics. We'll explore the fundamental concepts and tackle questions related to optics. Discover the secrets behind the behavior of light, reflection, refraction, and the wonders of lenses and mirrors. You'll find all you need in one convenient place. With free downloadable PDFs, students can easily grasp these concepts and problem-solving techniques. This resource will be a valuable asset for your JEE Main preparation, helping you excel in your exams.

FAQs on Optics Chapter - Physics JEE Main

1. What is the weightage of the chapter Optics in the JEE exam?

Optics is perhaps one of the most often questioned topics in JEE main, particularly in JEE Advanced. So, certainly, it is heavily weighted in the JEE examinations. Every year, approximately 3-4 questions are asked from this chapter, which leads to approximately 4-5 % weightage.

2. Is it crucial to prepare the Optics chapter for JEE?

If someone wants to score well and achieve a better rank in JEE competitive exams, then it is very important to prepare for this chapter. Moreover, the weightage of this chapter is more as compared to the others; hence it has to be given more importance.

3. Is it truly beneficial to practise previous years’ questions based on this Optics chapter for this exam?

We must practise previous years’ questions in order to score well and become familiar with the exam's difficulty level. It not only enhances our self-esteem but also exposes us to areas where we may need practice. Solving previous 10 to 15 years’ question papers can help you better comprehend a concept and can also give you an indication of how many times a concept or topic has been repeated in the test. You can prepare for physics chapters and measure JEE notes by practising previous years’ questions, which will turn out to be beneficial.