
A 24 volt battery of internal resistance 4 ohm is connected to a variable resistor at what value of the current from the battery is the rate of heat produced in the resistor maximum ?
Answer
415.5k+ views
Hint: To answer this question we will first find current using voltage, resistance and internal resistance. Then we will find power In the circuit, and then we will find the heat in the circuit using power and time. Then we will apply a condition to get the current required to get maximum heat in the circuit.
Formula used:
where
Where
Where
Complete step by step answer:
Look at the following diagram:
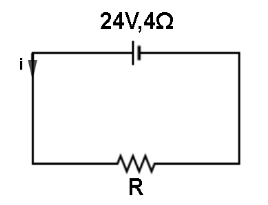
Using the formula for the current we get:
Now we will find power: we know that electric power is the rate per unit time at which electrical energy is transferred by an electric circuit. Hence it is given mathematically as a product of square of current and resistance through the circuit,
Now we will find heat: we know that heat is the form of energy that is transferred between two substances at different temperatures. But in terms of power heat is mathematically expressed as a product of power and time.
Now we want this heat to be maximum. Hence the condition will be that
Hence
Hence a 24V battery will produce maximum heat when current through it is 3 amperes.
Note: We usually find current in a circuit by dividing potential by resistance, but here the cell itself has an internal resistance. Hence it should also be considered. hence Do not forget to consider internal resistance while finding current.
Formula used:
where
Where
Where
Complete step by step answer:
Look at the following diagram:

Using the formula for the current we get:
Now we will find power: we know that electric power is the rate per unit time at which electrical energy is transferred by an electric circuit. Hence it is given mathematically as a product of square of current and resistance through the circuit,
Now we will find heat: we know that heat is the form of energy that is transferred between two substances at different temperatures. But in terms of power heat is mathematically expressed as a product of power and time.
Now we want this heat to be maximum. Hence the condition will be that
Hence
Hence a 24V battery will produce maximum heat when current through it is 3 amperes.
Note: We usually find current in a circuit by dividing potential by resistance, but here the cell itself has an internal resistance. Hence it should also be considered. hence Do not forget to consider internal resistance while finding current.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




