
A 3p orbital has:
A.two non-spherical nodes
B.two spherical nodes
C.one spherical and non-spherical nodes
D.one spherical and two non-spherical nodes
Answer
147.6k+ views
Hint: Node is a point or space around the nucleus where the probability of finding an electron is zero. This point where the wave function that describes the respective orbital is zero. There are 2 types of nodes, radial nodes also called spherical nodes and angular nodes which can be called non-spherical nodes. Spherical nodes can be found out by equation \[n - 1 - 1\] and there are a number of non-spherical nodes.
Complete step by step solution:
We have to find the number of nodes present in the 3p orbital. For that we want to know the principal quantum number (n) and azimuthal quantum number (l) of 3p orbital. The table given below gives the value of n l and ml value of different orbitals,
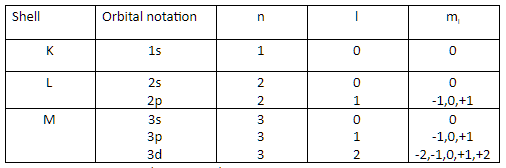
For 3p orbital we have \[n = 3,l = 1\]
Total number of nodes= \[n - 1\] , that is 3-1=2.
Number of spherical nodes= \[n - 1 - 1\] , that is 3-1-1=1
Number of non-spherical node or angular node= \[l = 1\]
There are a total of two nodes, they are one spherical node and one non-spherical node.
Hence, the answer is option (C) i.e One Spherical and non-spherical nodes.
Note: Electrons are arranged in an atom. The energy and the location of every electron in an atom is determined by a set of quantum numbers. The Principal quantum number is the shell number itself which represents the energy level. Azimuthal quantum number l is found by \[n - 1\] and the ml value varies from –l to +l. Azimuthal quantum number gives the orbital angular momentum and describes the shape of the orbital. Magnetics quantum denotes the different orientation possible for the different orbits. Those numbers describe different atomic orbits. Atomic orbital is the region of probability where the electron can be found. a spherical node can be defined as the spherical surface where the probability of finding an electron is zero. Nodes other than spherical nodes are considered as the non-spherical nodes.
Complete step by step solution:
We have to find the number of nodes present in the 3p orbital. For that we want to know the principal quantum number (n) and azimuthal quantum number (l) of 3p orbital. The table given below gives the value of n l and ml value of different orbitals,

For 3p orbital we have \[n = 3,l = 1\]
Total number of nodes= \[n - 1\] , that is 3-1=2.
Number of spherical nodes= \[n - 1 - 1\] , that is 3-1-1=1
Number of non-spherical node or angular node= \[l = 1\]
There are a total of two nodes, they are one spherical node and one non-spherical node.
Hence, the answer is option (C) i.e One Spherical and non-spherical nodes.
Note: Electrons are arranged in an atom. The energy and the location of every electron in an atom is determined by a set of quantum numbers. The Principal quantum number is the shell number itself which represents the energy level. Azimuthal quantum number l is found by \[n - 1\] and the ml value varies from –l to +l. Azimuthal quantum number gives the orbital angular momentum and describes the shape of the orbital. Magnetics quantum denotes the different orientation possible for the different orbits. Those numbers describe different atomic orbits. Atomic orbital is the region of probability where the electron can be found. a spherical node can be defined as the spherical surface where the probability of finding an electron is zero. Nodes other than spherical nodes are considered as the non-spherical nodes.
Recently Updated Pages
Complex Numbers - Concept of Rotation, Rotation Number, and Rotation Theorem for JEE

Entropy of Different Process - Important Concepts and Tips for JEE

Current Loop as Magnetic Dipole Important Concepts for JEE

Birch Reduction and Lindlar Catalyst Important Concepts and Tips for JEE

Introduction to Dimensions - Important Concepts and Tips for JEE

Difference Between Crystalline and Amorphous Solid

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Hydrocarbons Class 11 Notes: CBSE Chemistry Chapter 9

NCERT Solutions for Class 11 Chemistry In Hindi Chapter 1 Some Basic Concepts of Chemistry

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025




