
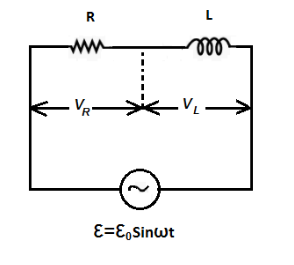
(a) An ac circuit as shown in the figure has an inductor of inductance
(b)The potential difference across the resistor is
(c) What will be the potential difference in the circuit when direct current is passed through the circuit?

Answer
495.9k+ views
Hint: First of all, we will draw the diagrams for which the solution will be easy to proceed. After that for the impedance part, we will individually find out resistance and the impedance. To calculate the effective value of the added voltage, we will use both the voltages of resistor and the inductor.
Complete step by step answer:
In the given question, we are supplied with the following data:
The figure has an inductor of inductance

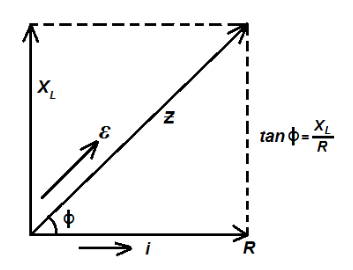
This is due to the fact that
Where,
Potential difference across the inductor is given as
We know from the Ohm’s law,
Where,
Now by substituting the required values in the equation (1), we get:
Potential difference across the resistor is
We know,
Where,
Hence, the effective value of applied voltage is
Now, we will find the net impedance, which is given by the following manipulation:
Hence, the total impedance of the circuit is
For intermediate time, we can write:
In this case, current
Note: This problem can be solved if you have a sound knowledge about phasor diagrams and alternating current. It should be remembered that in a purely capacitive circuit, the current will lead the voltage. Impedance is the net effective resistance offered by an electric circuit which contains resistor and inductor.
Complete step by step answer:
In the given question, we are supplied with the following data:
The figure has an inductor of inductance


This is due to the fact that
Where,
Potential difference across the inductor is given as

We know from the Ohm’s law,
Where,
Now by substituting the required values in the equation (1), we get:
Potential difference across the resistor is
We know,
Where,
Hence, the effective value of applied voltage is
Now, we will find the net impedance, which is given by the following manipulation:
Hence, the total impedance of the circuit is

For intermediate time, we can write:
In this case, current
Note: This problem can be solved if you have a sound knowledge about phasor diagrams and alternating current. It should be remembered that in a purely capacitive circuit, the current will lead the voltage. Impedance is the net effective resistance offered by an electric circuit which contains resistor and inductor.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Physics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




