
(a) An electric dipole of dipole moment
(b) Given the electric field in the region

Answer
492.9k+ views
Hint: Recall the formula for the electric field at a point due to point charge and also the formula for the electric field on the axial line of an electric dipole. Also keep in mind what is the electric flux? What is its formula? And also its nature and characteristics.
Complete step by step solution:
(a) Electric field on axial line of an electric dipole is given by,
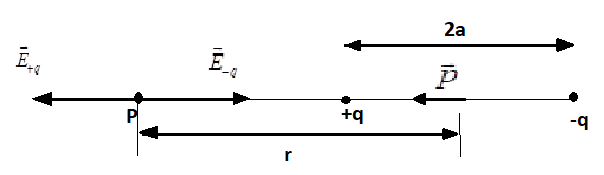
Let us assume that the P is the point at a distance r from the center of the given dipole on the side of charge –q.
Now, the formula for the electric field at point P due to –q is given by,
Now, the electric field at point P due to +q is given by,
Therefore, the total electric field at point P is,
At
In the question it is given the limit, so for
On further solving, we get
Hence, we got the desired result.
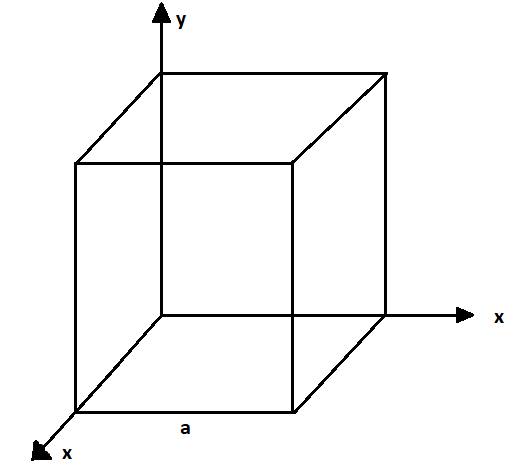
(b) The face of the cube that is perpendicular to the direction of x-axis, generates the flux. The face of the cube is parallel to the xy and xz plane and the electric field is parallel to the face, so the electric flux through them is zero.
Electric flux through the left face of the cube is given by,
Now, the electric flux through the right face of the cube is given by,
On putting the value
So the net flux through the cube is given by
And now the charge enclosed by the cube is given by,
Put the value for the flux from above in this equation, we get
On further solving, we get the charge enclosed by the cube,
Therefore, we get the net flux as
Note: Always remember that the electric flux is proportional to the number of electric field lines. It is basically defined as the measure of flow of the electric field through a given area. Also remember that the electric flux through a closed surface does not depend on the shape or size of the surface.
Complete step by step solution:
(a) Electric field on axial line of an electric dipole is given by,

Let us assume that the P is the point at a distance r from the center of the given dipole on the side of charge –q.
Now, the formula for the electric field at point P due to –q is given by,
Now, the electric field at point P due to +q is given by,
Therefore, the total electric field at point P is,
At
In the question it is given the limit, so for
On further solving, we get
Hence, we got the desired result.
(b) The face of the cube that is perpendicular to the direction of x-axis, generates the flux. The face of the cube is parallel to the xy and xz plane and the electric field is parallel to the face, so the electric flux through them is zero.
Electric flux through the left face of the cube is given by,
Now, the electric flux through the right face of the cube is given by,
On putting the value
So the net flux through the cube is given by
And now the charge enclosed by the cube is given by,
Put the value for the flux from above in this equation, we get
On further solving, we get the charge enclosed by the cube,
Therefore, we get the net flux as
Note: Always remember that the electric flux is proportional to the number of electric field lines. It is basically defined as the measure of flow of the electric field through a given area. Also remember that the electric flux through a closed surface does not depend on the shape or size of the surface.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




