
A battery having 12V emf and internal resistance
A)
B)
C)
D)
Answer
495k+ views
Hint: Voltage and current are given. So using the relation between internal resistance and emf find the resistance of an unknown resistor. Then, subtracting the total current flowing through
Formula used:
Complete answer:
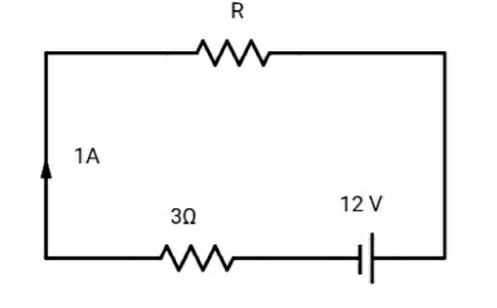
Given: Current in the circuit (I)= 1A
Internal Resistance (r)= 3
EMF (E) = 12V
We know the relation between internal resistance and EMF is given by,
Substituting values in above equation we get,
Lost voltage of the battery=
Substituting values in above equation ee get,
Lost Voltage =
Thus, the resistance of the resistor is
Hence, the correct answer is option C i.e.
Note:
- Lost voltage of the battery when circuit is closed is called the Terminal Voltage. There is an alternate method to solve this problem and find this terminal voltage.
- Using Ohm's law, this terminal voltage can also be calculated. Substituting the value of current and resistance of the resistor connected the terminal. Thus, you can calculate the lost voltage of the battery when the circuit is closed.
Formula used:
Complete answer:

Given: Current in the circuit (I)= 1A
Internal Resistance (r)= 3
EMF (E) = 12V
We know the relation between internal resistance and EMF is given by,
Substituting values in above equation we get,
Lost voltage of the battery=
Substituting values in above equation ee get,
Lost Voltage =
Thus, the resistance of the resistor is
Hence, the correct answer is option C i.e.
Note:
- Lost voltage of the battery when circuit is closed is called the Terminal Voltage. There is an alternate method to solve this problem and find this terminal voltage.
- Using Ohm's law, this terminal voltage can also be calculated. Substituting the value of current and resistance of the resistor connected the terminal. Thus, you can calculate the lost voltage of the battery when the circuit is closed.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




