
A battery of emf 10V and internal resistance
A. 10 V
B. 0 V
C. 8.5 V
D. 1.5 V
Answer
475.5k+ views
Hint: Using Kirchhoff’s voltage, express the current in the circuit and therefore, determine the value of resistance. Using Ohm’s law, express the voltage across the resistance. The voltage across the resistance is the terminal voltage of the battery.
Formula used:
Ohm’s law,
where, V is the voltage, I is the current and R is the resistance.
Complete step by step answer:
We have given that a supply of emf 10V is given to the circuit containing a resistor of
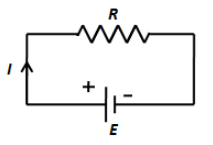
Let the internal resistance of the battery is r. Applying Kirchhoff’s voltage law in the closed loop, we get,
This is the magnitude of the current that will flow through the circuit.
Rearranging the above equation for r, we get,
Substituting 10V for E, 0.5A for I and
Now, we can express the terminal voltage in the circuit as voltage drop across the resistance R.
Substituting 0.5A for I and
Thus, the terminal voltage of the battery when the circuit is closed is equal to 8.5 V.
So, the correct answer is option C.
Note: While using Kirchhoff’s law, if there is drop in the voltage across the component of the circuit, the voltage should be taken as negative and if there is addition of the voltage. The internal resistance of the battery is negligible and therefore, we often neglect its value. The direction of the conventional current in the circuit is always from the positive terminal of the battery towards its negative terminal.
Formula used:
Ohm’s law,
where, V is the voltage, I is the current and R is the resistance.
Complete step by step answer:
We have given that a supply of emf 10V is given to the circuit containing a resistor of

Let the internal resistance of the battery is r. Applying Kirchhoff’s voltage law in the closed loop, we get,
This is the magnitude of the current that will flow through the circuit.
Rearranging the above equation for r, we get,
Substituting 10V for E, 0.5A for I and
Now, we can express the terminal voltage in the circuit as voltage drop across the resistance R.
Substituting 0.5A for I and
Thus, the terminal voltage of the battery when the circuit is closed is equal to 8.5 V.
So, the correct answer is option C.
Note: While using Kirchhoff’s law, if there is drop in the voltage across the component of the circuit, the voltage should be taken as negative and if there is addition of the voltage. The internal resistance of the battery is negligible and therefore, we often neglect its value. The direction of the conventional current in the circuit is always from the positive terminal of the battery towards its negative terminal.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




