
A beaker containing water is placed on the platform of a digital weighing machine. It reads
A)
B)
C)
D)
Answer
147.6k+ views
Hint: When the block is inserted with the help of a string the water in the beaker will be displaced. Volume of water displaced will be equal to the volume of the body. The weight of the water displaced will exert an upthrust on the body so the body will exert an equal and opposite force to this upthrust which will result in the increase in the value of the reading on the weighing machine.
Complete step by step solution:
It is given that a beaker containing water has a mass
A metal body is suspended in the water using a string which is fixed to a support.
The density of the metal body is given as
Mass of the metal body is given as
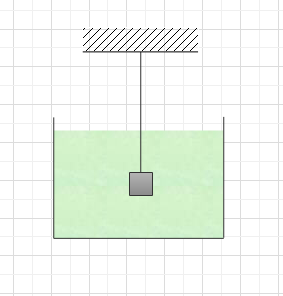
We need to find the new reading of the weighing machine after suspending the metal body in water.
We know that when a body is immersed in water, it will displace an amount of water equal to its volume.
That is, the volume of water displaced
So, the water will exert a thrust on the body which is equal to the weight of the water displaced.
The body will exert an equal and opposite force downward on the water because according to Newton's third law every action has an equal and opposite reaction.
So, this force acting downwards will add to the weight of the total system and hence the reading of the weighing machine will increase.
Let us find the value of the upthrust.
Upthrust is given by the equation
Where,
We know that the density is mass divided by volume.
Where, m is the mass and V is the volume.
Let us substitute the given values of density and volume of the metal block. Then we get
We know the density of water is
The acceleration due to gravity can be taken as
Let us substitute all these values in the equation for upthrust.
Then we get
same amount of force as the upthrust will be acting downwards.
We know that weight is the product of mass and acceleration due to gravity
Hence, the new reading of the weighing machine will be the sum of initial reading and the extra amount added.
Thus, we get new reading as
This is the new reading of the weighing machine.
So, the correct answer is option (B).
Note: If the metal block is inserted without the help of a string such that it does not touch the walls, then the total weight will be the sum of weight of the beaker containing water and the weight of the metal body. But when it is supported by a string then the new weight added will be equal in magnitude to the upthrust given as
Where,
Complete step by step solution:
It is given that a beaker containing water has a mass
A metal body is suspended in the water using a string which is fixed to a support.
The density of the metal body is given as
Mass of the metal body is given as

We need to find the new reading of the weighing machine after suspending the metal body in water.
We know that when a body is immersed in water, it will displace an amount of water equal to its volume.
That is, the volume of water displaced
So, the water will exert a thrust on the body which is equal to the weight of the water displaced.
The body will exert an equal and opposite force downward on the water because according to Newton's third law every action has an equal and opposite reaction.
So, this force acting downwards will add to the weight of the total system and hence the reading of the weighing machine will increase.
Let us find the value of the upthrust.
Upthrust is given by the equation
Where,
We know that the density is mass divided by volume.
Where, m is the mass and V is the volume.
Let us substitute the given values of density and volume of the metal block. Then we get
We know the density of water is
The acceleration due to gravity can be taken as
Let us substitute all these values in the equation for upthrust.
Then we get
same amount of force as the upthrust will be acting downwards.
We know that weight is the product of mass and acceleration due to gravity
Hence, the new reading of the weighing machine will be the sum of initial reading and the extra amount added.
Thus, we get new reading as
This is the new reading of the weighing machine.
So, the correct answer is option (B).
Note: If the metal block is inserted without the help of a string such that it does not touch the walls, then the total weight will be the sum of weight of the beaker containing water and the weight of the metal body. But when it is supported by a string then the new weight added will be equal in magnitude to the upthrust given as
Where,
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




