
A bird is flying at a height of 3.6m above the surface of water and a fish is in the water at a depth of 1.2m. The apparent height of the bird to the fish is (
A. 3.9 m
B. 4.8 m
C. 5.2 m
D. 6.0 m
Answer
466.8k+ views
Hint:When the light ray travels from a rare medium to a denser medium, the path of light bends towards the normal. Due to the bending, the height of the bird will appear raised than the real height. The refractive index of the medium is the ratio of the apparent height to the real height.
Formula used:
Here,
Complete step by step answer:
We know that when the light ray travels from a rare medium to a denser medium, the path of light bends towards the normal. The bending of light in the medium depends on the refractive index of the medium. Due to the bending of the light, the depth of the bottom surface of water appears changed. When the light ray from the bird travels from air to water it bends towards the normal and thus, the height of the bird appears raised to the fish. The following figure will ease our understanding.
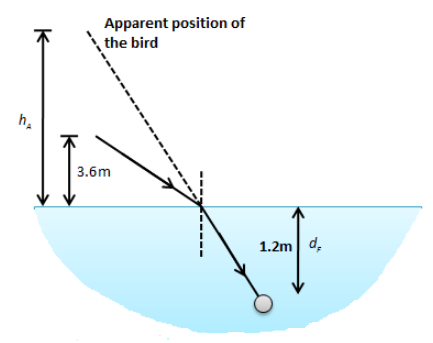
We can express the apparent height of the bird from the surface of water as,
Here,
Substituting
This is the apparent height of the fish above the surface of the water. Therefore, the apparent height of the bird from the fish will be,
Thus, the apparent height of the bird to the fish is 6 m.
So, the correct answer is option D.
Note:If it is for the bird, the depth of the fish would appear different for the bird than the real depth due to the different refractive index of the water. While drawing the ray diagram, if the observer is the fish, the incident ray should be the light ray reflected from the bird towards the eye of the fish. Therefore, the incident ray travels from air to water. The opposite will be for the bird as an observer.
Formula used:
Here,
Complete step by step answer:
We know that when the light ray travels from a rare medium to a denser medium, the path of light bends towards the normal. The bending of light in the medium depends on the refractive index of the medium. Due to the bending of the light, the depth of the bottom surface of water appears changed. When the light ray from the bird travels from air to water it bends towards the normal and thus, the height of the bird appears raised to the fish. The following figure will ease our understanding.

We can express the apparent height of the bird from the surface of water as,
Here,
Substituting
This is the apparent height of the fish above the surface of the water. Therefore, the apparent height of the bird from the fish will be,
Thus, the apparent height of the bird to the fish is 6 m.
So, the correct answer is option D.
Note:If it is for the bird, the depth of the fish would appear different for the bird than the real depth due to the different refractive index of the water. While drawing the ray diagram, if the observer is the fish, the incident ray should be the light ray reflected from the bird towards the eye of the fish. Therefore, the incident ray travels from air to water. The opposite will be for the bird as an observer.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE




