Question

A body is projected vertically upwards. What is the relation between the magnitudes of velocity (v) of the body on reaching the point of projection in its downward motion to velocity of projection (u)?
A) v< u
B) v>u
C) v=u
D) none of these

Answer 1

Hint:In this question we can use conservation of energy or can use equations of motion in vertical direction
We are going to use equations of motion to solve this question.

Complete step by step answer:
First we make a diagram of the question to understand clearly we assume a ball through upward direction with initial velocity $u$ in upward direction it goes to $h$ height and get rest for a while and started fall down due to gravity at the highest point the velocity of ball must be zero an when it start falling down its gain its velocity zero to $v$ when it strike at the earth.

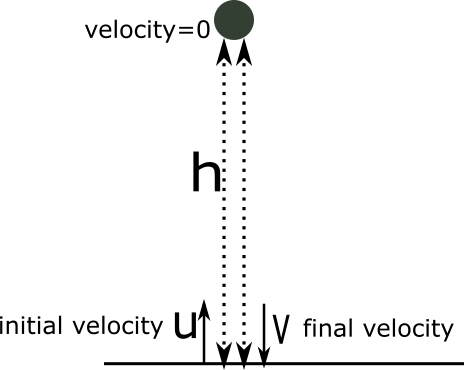
Ball through with velocity $u$ it can gain maximum height $h$ where its velocity becomes zero
Then from equation of motion ${v^2} = {u^2} - 2gh$
$ \Rightarrow 0 = {u^2} - 2gh$
By solving this can find value of maximum height it gains
$ \Rightarrow h = \dfrac{{{u^2}}}{{2g}}$ ....... (1)
At the highest point it start falling and gain velocity $v$ so again apply equation of motion now initial velocity zero and final velocity is $v$
$ \Rightarrow {v^2} = {0^2} + 2gh$
Put the value of h from equation (1)
${v^2} = 2g\left( {\dfrac{{{u^2}}}{{2g}}} \right)$
Again solving
$ \Rightarrow {v^2} = {u^2}$
So we can say
$\therefore v = u$
Initial velocity in upward direction at starting point =final velocity downward direction at starting point

So option C is correct

Note:In such types of questions the velocity at a point remains same in magnitude but opposite in direction.
Means if a ball through upward direction and we assume a point on its path then when the ball is going upward and coming downward both times it has the same velocity at the same point in the opposite direction.