Question

A bucket open at the top is in the form of frustum of a cone with a capacity of 12308.8 $c{{m}^{3}}$. The radius of the top and bottom of circular ends of the bucket are 20 cm and 12 cm respectively. Find the height of the bucket and also the area of the metal sheet used in making it? (use $\pi =3.14$).

Answer 1

Hint: We started solving the problem by assuming the height of the frustum as ‘h’. We solve for the height ‘h’ by using the given value of volume and volume of frustum formula. Once the height is found, we find the slant height of frustum. We now find the sum of curved surface area and area of bottom surface of frustum to get the area of the metal sheet used in making it.

Complete step by step answer:
Given that we have a bucket which is open at the top is in the form of frustum of a cone with a capacity of 12308.8 $c{{m}^{3}}$. The radius of top and bottom of circular ends of buckets are 20 cm and 12 cm. We need to find the height of the bucket and also the area of the metal sheet used in making it.
Let us draw the frustum showing all the dimensions to get a better view.

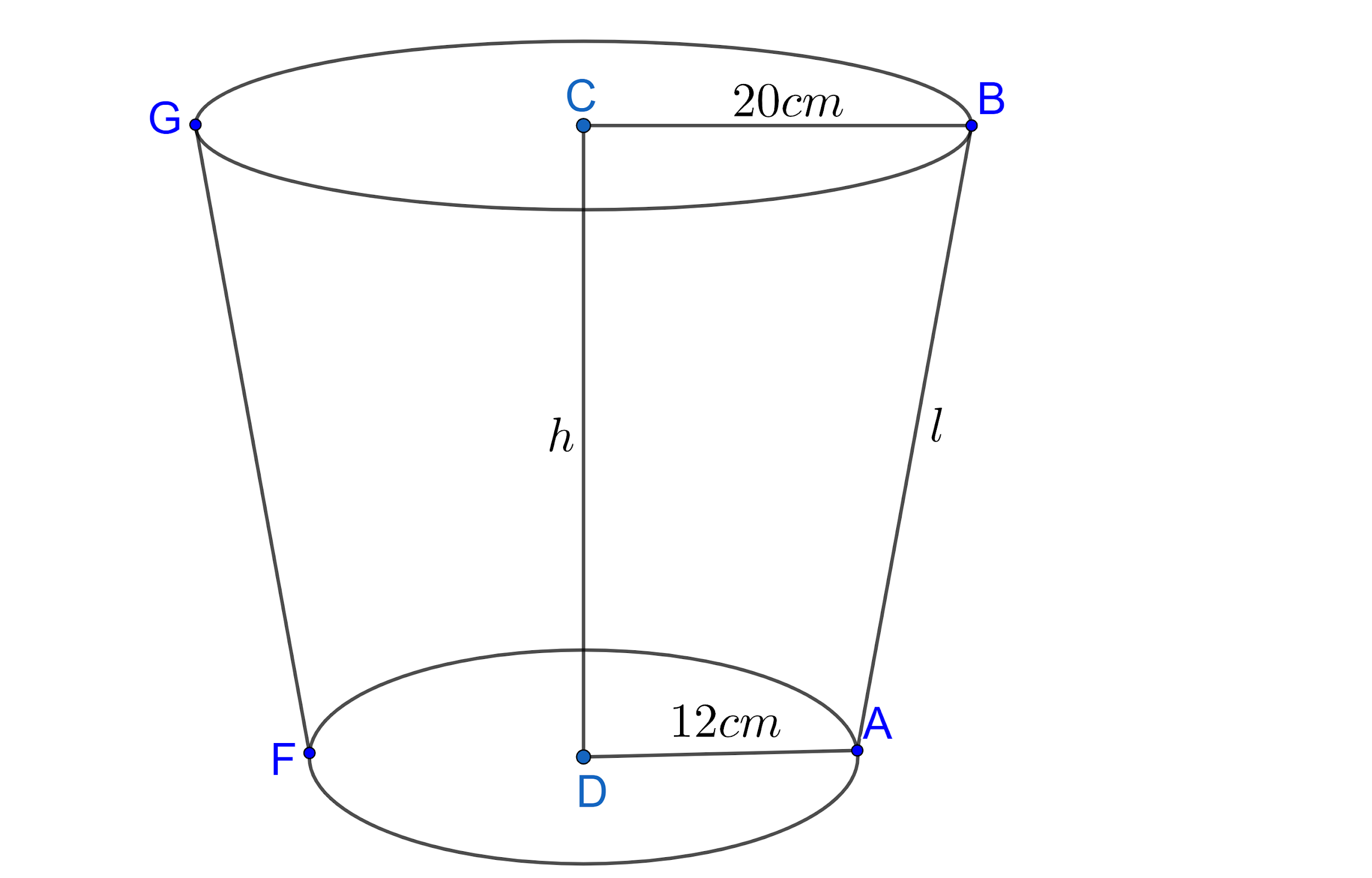
We have a bucket ABGF in the shape of frustum. Let us assume the height of the cone be ‘h’.
We know that the volume of the frustum(V) is defined as $V=\dfrac{\pi h}{3}\times \left( {{R}^{2}}+{{r}^{2}}+Rr \right)$. Where R is the radius of top surface and r is the radius of the bottom surface.
So, we have got $12308.8c{{m}^{3}}=\dfrac{\pi h}{3}\times \left( {{\left( 20 \right)}^{2}}+{{\left( 12 \right)}^{2}}+\left( \left( 20 \right).\left( 12 \right) \right) \right)$.
We have got $12308.8c{{m}^{3}}=\dfrac{3.14\times h}{3}\times \left( 400+144+240 \right)$.
We have got $12308.8c{{m}^{3}}=\dfrac{3.14\times h}{3}\times \left( 784 \right)$.
We have got $12308.8c{{m}^{3}}=\dfrac{h}{3}\times \left( 2461.76 \right)$.
We have got $12308.8c{{m}^{3}}=h\times 820.59$.
We have got $h=15cm$.
∴ We have got the height of the bucket as 15 cm.
Now, we find the area of the metal sheet that is used to make this bucket. Metal sheet is used only to make curved surface of bucket and base of the bucket. As the top is open and inside of bucket is empty to fill any material, we just need to find them.
So, we have area of the metal sheet = curved surface area of frustum + Area of the base circle.
We have area of the metal sheet = $\left( \pi \left( R+r \right)l \right)+\pi {{r}^{2}}$, where l is slant height, R is the radius of top surface and r is the radius of the bottom surface.
We find the value of slant height as $l=\sqrt{{{\left( R-r \right)}^{2}}+{{h}^{2}}}$.
We have got slant height $l=\sqrt{{{\left( 20-12 \right)}^{2}}+{{15}^{2}}}$.
We have got slant height $l=\sqrt{{{8}^{2}}+{{15}^{2}}}$.
We have got slant height $l=\sqrt{64+225}$.
We have got slant height $l=\sqrt{289}$.
We have got slant height $l=17$cm.
We have got area of metal sheet = $\left( \left( 3.14\times \left( 20+12 \right) \right)\times 17 \right)+\left( 3.14\times {{\left( 12 \right)}^{2}} \right)$.
We have an area of metal sheet = $\left( \left( 3.14\times 32 \right)\times 17 \right)+\left( 3.14\times \left( 144 \right) \right)$.
We have an area of metal sheet = 1708.16 + 452.16.
We have got an area of metal sheet = \[2160.32c{{m}^{2}}\].

∴ The area of the metal sheet used is \[2160.32c{{m}^{2}}\].

Note: We should not always use the value 3.14 for $\pi $, unless it is mentioned in the problem. We can solve the problem by extending the given problem into a cone and assigning the variables for height of cone. We get quadratic equations to solve for the value of height of frustum. We subtract the volume of cone with base top radius with volume of cone with bottom radius to get the volume of frustum.