Question

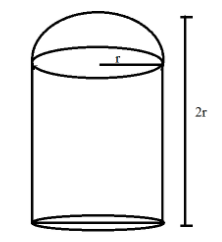
A building is in the form of a cylinder surmounted by a hemispherical vaulted dome and contains \[41\dfrac{{19}}{{21}}{m^3}\]of air. If the internal diameter of the dome is equal to its total height above the floor, find the height of the building.

Answer 1

Hint: Let us solve the problem by approaching the diagram
Volume of total building \[ = \]volume of cylinder \[ + \]volume of hemisphere
Now,
Volume of cylinder \[ = \pi {r^2}h\]
Volume of hemisphere \[ = \dfrac{2}{3}\pi {r^3}\]

Complete answer:
Given that
   

Volume of building \[ = 41\dfrac{{19}}{{21}}{m^3}...(1)\]
Internal diameter of dome is equal to its total height above the floor
Let radial of cylinder \[ = r\]
\[ \Rightarrow \] volume of building \[ = \]volume of air
\[ \Rightarrow \] Height of the building \[ = 2r\]
Volume of total building \[ = \]volume of cylinder \[ + \]volume of hemisphere… (2)
Now, Volume of cylinder \[ = \pi {r^2}h\]
\[ = \pi {r^2}(r) = {\pi ^3}{m^3}\]
Volume of hemisphere \[ = \dfrac{2}{3}\pi {r^3}{m^3}\]
Now from \[e{q^n}...(1)\]
Volume of total building \[ = (\pi {r^3} + \dfrac{2}{3}\pi {r^3}){m^3}\]
\[ = \pi {r^3}(2 + \dfrac{2}{3}){m^3}\]
\[ = \dfrac{5}{3}\pi {r^3}{m^3}.....(2)\]
From equation (1) and (3)
\[41\dfrac{{19}}{{21}}{m^3} = \dfrac{5}{3}\pi {r^3}{m^3}\]
\[(\because \pi = \dfrac{{22}}{7})\]
\[ \Rightarrow \dfrac{{176}}{7} \times \dfrac{7}{{27}} = {r^3}\]
\[ \Rightarrow {r^3} = 8\]
\[ \Rightarrow r = {({2^3})^{\dfrac{1}{3}}}\]
\[ \Rightarrow r = 2m\]
From equation (b)
Height of building \[ = 2r\]
\[ = 2(2m) = 4m\]

Note: The volume of a cylinder is the density of the cylinder which signifies the amount of material it can carry or how much amount of any material can be immersed in it. It is given by the formula, \[\pi {r^2}h,\] where r, is the radius of the circular base and \[h\]is the height of the cylinder. The material could be a liquid quantity or any substance which can be filled in the cylinder uniformly.
The volume of the sphere is the capacity it has. The sphere present in the building is in the form of a hemisphere.
In this case use cylinder & sphere’s volume formulas.