
A candle flame 3 cm high is placed at a distance of 3 m from the wall. How far from the wall must a concave mirror be placed so that it may form a 9 cm image of the flame on the same wall? Also, find the focal length of the mirror.
Answer
439k+ views
Hint: The distance of the wall from the mirror will become their distance of the image from the mirror v. We can then find this v by using the formula for magnification of a concave mirror as we are given image and object heights.
Formula used:
The magnification produced by a concave mirror is given as:
Complete step-by-step solution:
We are given;
Height of the object
Height of the image
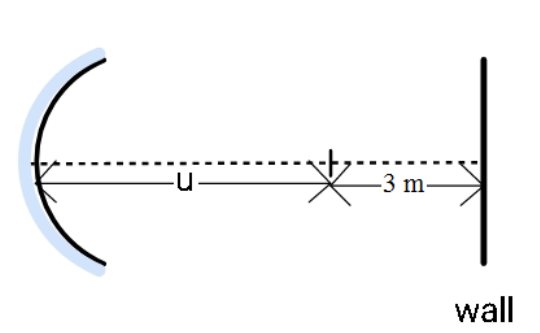
The distance of the candle from the wall is 3 m. We assume the distance of the mirror from the wall is v meters, which is also where we want our image to form so it is basically image distance. Therefore object distance from the mirror can be written as v - 3 meters.
Substituting all of the above in the magnification equation we get:
Therefore v = 4.5 m is the distance of the wall from the mirror.
The focal length of a spherical mirror is given as:
As we already kept u = v -3 then, we can substitute u = 1.5 m as v = 4.5 m.
or we can write:
Therefore, as we place a concave mirror of focal length 1.125 m in front of the wall at a separation of 4.5 m from the wall, we will find the size of the image of the flame to be 9 cm on the wall.
Note: By sign conventions we must take v and u to be negative so that the focal length found will also be negative. Since we're given no information about whether the image formed is inverted or erect, we should not bother to place a negative sign for image height. In case if the image formed is inverted the answer will be completely different. Therefore we assume that the image formed is erect.
Formula used:
The magnification produced by a concave mirror is given as:
Complete step-by-step solution:
We are given;
Height of the object
Height of the image

The distance of the candle from the wall is 3 m. We assume the distance of the mirror from the wall is v meters, which is also where we want our image to form so it is basically image distance. Therefore object distance from the mirror can be written as v - 3 meters.
Substituting all of the above in the magnification equation we get:
Therefore v = 4.5 m is the distance of the wall from the mirror.
The focal length of a spherical mirror is given as:
As we already kept u = v -3 then, we can substitute u = 1.5 m as v = 4.5 m.
or we can write:
Therefore, as we place a concave mirror of focal length 1.125 m in front of the wall at a separation of 4.5 m from the wall, we will find the size of the image of the flame to be 9 cm on the wall.
Note: By sign conventions we must take v and u to be negative so that the focal length found will also be negative. Since we're given no information about whether the image formed is inverted or erect, we should not bother to place a negative sign for image height. In case if the image formed is inverted the answer will be completely different. Therefore we assume that the image formed is erect.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




