
A charged particle of specific charge (charge/mass)
Answer
486.6k+ views
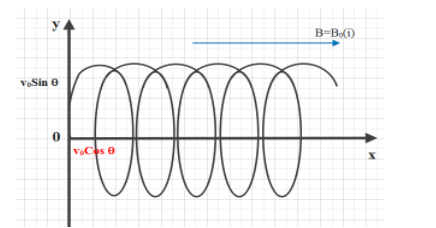
Hint: We must know that any charged particle, in the presence of a magnetic field, experiences a force in the direction perpendicular to both the velocity and the magnetic field. In this case, there are two kinds of motion, translational and rotational, which results in a combined helical motion of the particle. Here, the particle will be having translational motion through the x-axis and circular motion through the z-axis.
Formula used:
Complete step by step answer:
In this question, a particle of specific charge
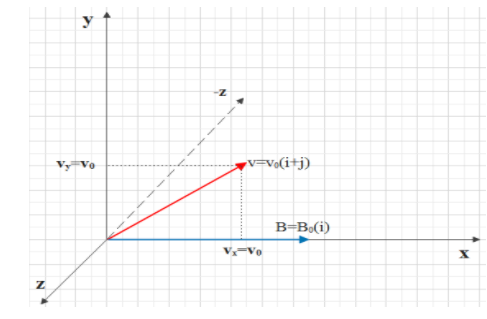
Now, it is given that specific charge,
We know, the particle will follow a helical path with a time period,
We are asked to find the position of the particle at time
Now, in order to find the coordinates of the particle at
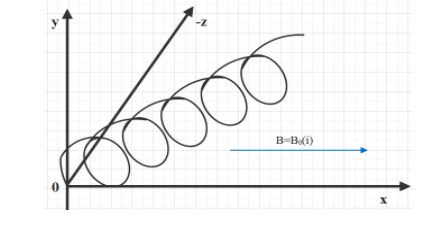
So, as we are considering the position of the particle at
Then, the x coordinate will be equal to the linear distance covered by the particle which will be given by,
Where s is the displacement along x axis, v is the velocity of the particle and t is the time.
So, the x coordinate will be given by,
The helix will be forming in the z-x plane and the y coordinate will be zero. Or we can interpret the y coordinate becoming zero as, the angle between velocity of the particle and the direction of magnetic field will be 90 degrees. So the force becomes zero and no force will be acting in y-direction. So the particle will travel in a helical path through the z-x plane. That is,
Now, at
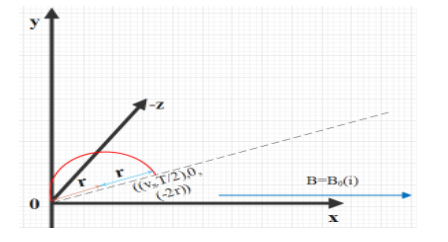
So, at
Where r is the radius of the semi-circle.
Therefore, the coordinates of the particle at time
Now, to find the radius of the circle, we can use the concept,
The force due to the magnetic field will supply the necessary centripetal force for the circular motion. That is,
So, we can get the coordinates as,
That is,
So, option D is the correct answer.
Note: We must know that the helical path of the changed particle can be traced from Fleming’s right-hand rule by applying it at either end of the diameter of the circle. Here, we can observe that the velocity of the charged particle is not subject to change. Understanding the direction of propagation of the waves is most important.
Formula used:
Complete step by step answer:
In this question, a particle of specific charge

Now, it is given that specific charge,
We know, the particle will follow a helical path with a time period,
We are asked to find the position of the particle at time
Now, in order to find the coordinates of the particle at

So, as we are considering the position of the particle at
Then, the x coordinate will be equal to the linear distance covered by the particle which will be given by,
Where s is the displacement along x axis, v is the velocity of the particle and t is the time.
So, the x coordinate will be given by,
The helix will be forming in the z-x plane and the y coordinate will be zero. Or we can interpret the y coordinate becoming zero as, the angle between velocity of the particle and the direction of magnetic field will be 90 degrees. So the force becomes zero and no force will be acting in y-direction. So the particle will travel in a helical path through the z-x plane. That is,

Now, at

So, at
Where r is the radius of the semi-circle.
Therefore, the coordinates of the particle at time
Now, to find the radius of the circle, we can use the concept,
The force due to the magnetic field will supply the necessary centripetal force for the circular motion. That is,
So, we can get the coordinates as,
That is,
So, option D is the correct answer.
Note: We must know that the helical path of the changed particle can be traced from Fleming’s right-hand rule by applying it at either end of the diameter of the circle. Here, we can observe that the velocity of the charged particle is not subject to change. Understanding the direction of propagation of the waves is most important.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




