
A circular racecourse track has a radius of $500\;m$ and is banked to $10^{\circ}$. If the coefficient of friction between tyres of a vehicle and the road surface is $0.25$, compute:
A). The maximum speed to avoid slipping
B). The optimum speed to avoid wear and tear
Answer
480.6k+ views
Hint: Try to illustrate the situation which may help you account for all the components of forces contributing to the equilibrium of the vehicle. In other words, resolve the normal reaction, frictional force, and weight into their respective components and deduce which of the components act in the vertical and horizontal directions. Play around with the equations thus obtained to arrive at an expression for velocity. Do not forget to account for the banking of the road, the frictional force, as well as the centrifugal force acting on the vehicle as it moves in a circular path.
Formula Used:
Maximum speed to avoid slipping: $ v_{max} = \sqrt {\dfrac{rg(tan \theta + \mu)}{ 1 – \mu tan\theta }}$
Optimum speed to avoid wear and tear: $v_{optimum} = \sqrt{rg\;tan\theta}$
Here, r is the track radius, g is the acceleration due to gravity, $\theta$ is the banking angle, and $\mu$ is the coefficient of friction.
Complete step-by-step solution:
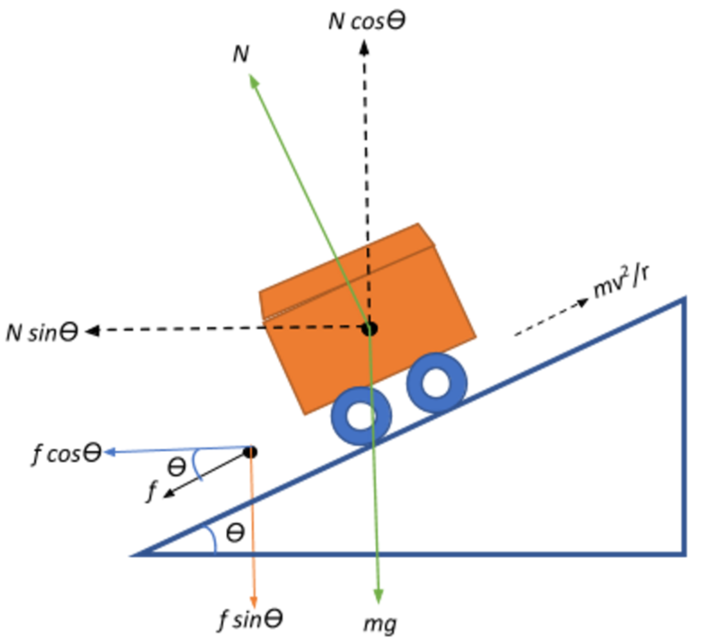
Let us deconstruct the question to derive an expression for a vehicle moving on this banked racetrack in general
Let that mass of the vehicle be $m$, and let it move with a velocity $v$. Since its moving in a circular racetrack with radius $r$, it experiences a centrifugal force of magnitude $F_{c} = \dfrac{mv^2}{r}$ directed outwards.
Let $\theta$ be the angle of banking and let $f$ be the frictional force acting between the road and the tyres.
Note that we divide the forces in play into their respective components to ease our understanding and simplify calculations. These are shown in the diagram.
Now, for a balanced system, the
total upward force = total downward force
$\Rightarrow N\;cos\theta = mg + f\;sin\theta \Rightarrow mg = N\;cos\theta – f\;sin\theta$
Similarly, we look at the lateral forces, which for a balanced system:
$\dfrac{mv^2}{r} = N\;sin\theta + f\;cos\theta$.
On dividing the above two equations we get:
$\dfrac{\dfrac{mv^2}{r}}{mg} = \dfrac{ N\;sin\theta + f\;cos\theta }{N\;cos\theta – f\;sin\theta} \Rightarrow \dfrac{v^2}{rg} = \dfrac{ N\;sin\theta + f\;cos\theta }{N\;cos\theta – f\;sin\theta} $
Now, substituting frictional force $f = \mu N$ in the above equation we get:
$\dfrac{v^2}{rg} = \dfrac{ N\;sin\theta + \mu N\;cos\theta }{N\;cos\theta – \mu N\;sin\theta} \Rightarrow \dfrac{v^2}{rg} = \dfrac{ sin\theta + \mu cos\theta }{cos\theta – \mu sin\theta} $
Now, dividing both the numerator and the denominator by $cos\theta$:
$\dfrac{v^2}{rg} = \dfrac{ tan \theta + \mu }{1 – \mu tan\theta} \Rightarrow v = \sqrt {\dfrac{rg(tan \theta + \mu)}{ 1 – \mu tan\theta }}$
a). We can now calculate the maximum speed to avoid slipping:
This is basically the maximum speed that the vehicle can travel under the influence of friction. This is given by the expression we derived above:
($tan 10^{\circ} = 0.1763$)
$ v_{max} = \sqrt {\dfrac{rg(tan \theta + \mu)}{ 1 – \mu tan\theta }} = \sqrt {\dfrac{500
\times 9.8 (0.1763 + 0.23)}{ 1 – (0.25 \times 0.1763)}} = \sqrt{2185.182} $
$\Rightarrow v_{max} = 46.75\;ms^{-1}$
b). The optimum speed to avoid wear and tear can be given by disregarding friction and taking $\mu = 0$, in which case:
$v_{optimum} = \sqrt{rg\;tan\theta} = \sqrt{500 \times 9.8 \times 0.1763} = \sqrt{863.87}$
$\Rightarrow v_{optimum} = {29.39 \;ms^{-1}} $
Note: It is always essential to deduce which component of the forces are acting in which direction. By resolving our influencing forces to their respective components, we are able to isolate only those components of forces that contribute to the equilibrium of the body in that direction.
Also, remember that for the first part we considered an expression for velocity when friction was prevalent. This is because to avoid slipping the vehicle must get a grip, and this grip is provided by the friction between the road and the tyres.
For the second part, we discount friction only because the major source of wear and tear of the tyres is due to frictional forces that act between the tyres and the road.
Formula Used:
Maximum speed to avoid slipping: $ v_{max} = \sqrt {\dfrac{rg(tan \theta + \mu)}{ 1 – \mu tan\theta }}$
Optimum speed to avoid wear and tear: $v_{optimum} = \sqrt{rg\;tan\theta}$
Here, r is the track radius, g is the acceleration due to gravity, $\theta$ is the banking angle, and $\mu$ is the coefficient of friction.
Complete step-by-step solution:

Let us deconstruct the question to derive an expression for a vehicle moving on this banked racetrack in general
Let that mass of the vehicle be $m$, and let it move with a velocity $v$. Since its moving in a circular racetrack with radius $r$, it experiences a centrifugal force of magnitude $F_{c} = \dfrac{mv^2}{r}$ directed outwards.
Let $\theta$ be the angle of banking and let $f$ be the frictional force acting between the road and the tyres.
Note that we divide the forces in play into their respective components to ease our understanding and simplify calculations. These are shown in the diagram.
Now, for a balanced system, the
total upward force = total downward force
$\Rightarrow N\;cos\theta = mg + f\;sin\theta \Rightarrow mg = N\;cos\theta – f\;sin\theta$
Similarly, we look at the lateral forces, which for a balanced system:
$\dfrac{mv^2}{r} = N\;sin\theta + f\;cos\theta$.
On dividing the above two equations we get:
$\dfrac{\dfrac{mv^2}{r}}{mg} = \dfrac{ N\;sin\theta + f\;cos\theta }{N\;cos\theta – f\;sin\theta} \Rightarrow \dfrac{v^2}{rg} = \dfrac{ N\;sin\theta + f\;cos\theta }{N\;cos\theta – f\;sin\theta} $
Now, substituting frictional force $f = \mu N$ in the above equation we get:
$\dfrac{v^2}{rg} = \dfrac{ N\;sin\theta + \mu N\;cos\theta }{N\;cos\theta – \mu N\;sin\theta} \Rightarrow \dfrac{v^2}{rg} = \dfrac{ sin\theta + \mu cos\theta }{cos\theta – \mu sin\theta} $
Now, dividing both the numerator and the denominator by $cos\theta$:
$\dfrac{v^2}{rg} = \dfrac{ tan \theta + \mu }{1 – \mu tan\theta} \Rightarrow v = \sqrt {\dfrac{rg(tan \theta + \mu)}{ 1 – \mu tan\theta }}$
a). We can now calculate the maximum speed to avoid slipping:
This is basically the maximum speed that the vehicle can travel under the influence of friction. This is given by the expression we derived above:
($tan 10^{\circ} = 0.1763$)
$ v_{max} = \sqrt {\dfrac{rg(tan \theta + \mu)}{ 1 – \mu tan\theta }} = \sqrt {\dfrac{500
\times 9.8 (0.1763 + 0.23)}{ 1 – (0.25 \times 0.1763)}} = \sqrt{2185.182} $
$\Rightarrow v_{max} = 46.75\;ms^{-1}$
b). The optimum speed to avoid wear and tear can be given by disregarding friction and taking $\mu = 0$, in which case:
$v_{optimum} = \sqrt{rg\;tan\theta} = \sqrt{500 \times 9.8 \times 0.1763} = \sqrt{863.87}$
$\Rightarrow v_{optimum} = {29.39 \;ms^{-1}} $
Note: It is always essential to deduce which component of the forces are acting in which direction. By resolving our influencing forces to their respective components, we are able to isolate only those components of forces that contribute to the equilibrium of the body in that direction.
Also, remember that for the first part we considered an expression for velocity when friction was prevalent. This is because to avoid slipping the vehicle must get a grip, and this grip is provided by the friction between the road and the tyres.
For the second part, we discount friction only because the major source of wear and tear of the tyres is due to frictional forces that act between the tyres and the road.
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Trending doubts
10 examples of friction in our daily life

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

State and prove Bernoullis theorem class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

Write down 5 differences between Ntype and Ptype s class 11 physics CBSE




