
A convexo-concave diverging lens is made of glass of refractive index 1.5 and focal length 24 cm. Radius of curvature for one surface is double that of the other. Then, the radii of curvature for the two surfaces are (in cm):
A.6,12
B.12,14
C.3,6
D.18,36

Answer
502.5k+ views
Hint: Recall that for a diverging lens, the focal length is negative. Use the lens maker’s formula to establish a relation between the focal length, the refractive index of the lens and the radii of curvature of both the surfaces. Keep in mind that the radius of curvature of one surface is twice the other. Then, accounting for the curvature sign conventions, arrive at a relationship between the two radii and plug in the numbers to get the actual value of the lens surfaces’ radii of curvature.
Formula Used:
Lens maker’s formula:
Complete answer:
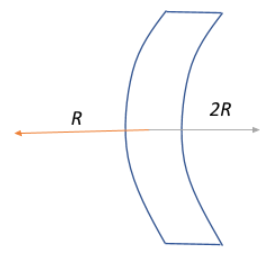
We have a convexo-concave lens as shown in the diagram. This means that one surface is derived from a convex lens and the other surface from a concave lens, and together, they have like a diverging lens, as mentioned in the question.
According to the Cartesian Sign Convention, the focal length of a diverging lens is negative, therefore, focal length of our convexo-concave lens will be
The refractive index of the lens is given to be
Let the radius of curvature of the concave surface be
Then the radius of curvature of the convex surface will be
These are in accordance with the sign convention since positive radii of curvature is associated with those surfaces that appear to be convex when seen from the left.
We know that the Lens Maker’s Formula relates all the above quantities together as follows:
Substituting the values:
Therefore, the correct choice would be: A. 6,12.
Note:
Keep in mind that all values assume the Cartesian sign convention. For converging lenses, the focal length is positive whereas for diverging lenses focal length is negative.
The sign convention for the optical radius of curvature of lens surfaces:
If the surface seems convex when seen from the left, or when the centre of curvature is to the right of the surface, the radius of curvature is taken as positive, whereas if the surface seems concave when viewed from the left, or when the centre of curvature is to the left of the surface, then the radius of curvature is taken as negative.
Formula Used:
Lens maker’s formula:
Complete answer:
We have a convexo-concave lens as shown in the diagram. This means that one surface is derived from a convex lens and the other surface from a concave lens, and together, they have like a diverging lens, as mentioned in the question.
According to the Cartesian Sign Convention, the focal length of a diverging lens is negative, therefore, focal length of our convexo-concave lens will be
The refractive index of the lens is given to be
Let the radius of curvature of the concave surface be
Then the radius of curvature of the convex surface will be
These are in accordance with the sign convention since positive radii of curvature is associated with those surfaces that appear to be convex when seen from the left.
We know that the Lens Maker’s Formula relates all the above quantities together as follows:
Substituting the values:
Therefore, the correct choice would be: A. 6,12.
Note:
Keep in mind that all values assume the Cartesian sign convention. For converging lenses, the focal length is positive whereas for diverging lenses focal length is negative.
The sign convention for the optical radius of curvature of lens surfaces:
If the surface seems convex when seen from the left, or when the centre of curvature is to the right of the surface, the radius of curvature is taken as positive, whereas if the surface seems concave when viewed from the left, or when the centre of curvature is to the left of the surface, then the radius of curvature is taken as negative.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




