
A current loop ABCD is held fixed on the plane of the paper as shown in the figure. The arc BC
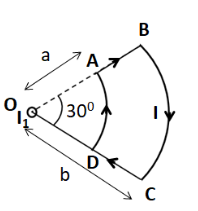
(A) The forces on AB and DC are zero
(B) The forces on AD and BC are zero
(C) The magnitude of the net force on the loop is given by
(D) The magnitude of the net force on the loop is given by

Answer
477.9k+ views
1 likes
Hint : To solve this question, we need to draw magnetic field lines due to the current
Formula Used: The formula used in solving this question is given by
Here
Complete step by step answer
As the straight thin wire carrying current
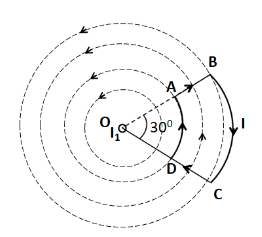
Now, as we can clearly see that the magnetic field lines are in the form of concentric circles, which are parallel to the circular portions AD and BC. We know that the magnetic force on a current carrying conductor is given by
As the magnetic field is parallel and anti-parallel to the whole circumference of the wires AD and BC respectively, so the magnetic force is equal to zero on both the wires.
So option B is correct.
Now, for the wires AB and CD, the magnetic field lines are not parallel to them. So, the force will not be equal to zero.
Therefore, option A is incorrect.
As only the straight wires AB and CD experience magnetic force due to the current
Therefore both the options C and D are incorrect.
Hence, the correct answer is option B.
Note
There is no need to obtain the value of the force experienced by the wires AB and CD. Though we can obtain its value, but it will require extra time and effort.
Formula Used: The formula used in solving this question is given by
Here
Complete step by step answer
As the straight thin wire carrying current

Now, as we can clearly see that the magnetic field lines are in the form of concentric circles, which are parallel to the circular portions AD and BC. We know that the magnetic force on a current carrying conductor is given by
As the magnetic field is parallel and anti-parallel to the whole circumference of the wires AD and BC respectively, so the magnetic force is equal to zero on both the wires.
So option B is correct.
Now, for the wires AB and CD, the magnetic field lines are not parallel to them. So, the force will not be equal to zero.
Therefore, option A is incorrect.
As only the straight wires AB and CD experience magnetic force due to the current
Therefore both the options C and D are incorrect.
Hence, the correct answer is option B.
Note
There is no need to obtain the value of the force experienced by the wires AB and CD. Though we can obtain its value, but it will require extra time and effort.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹34,851 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




