
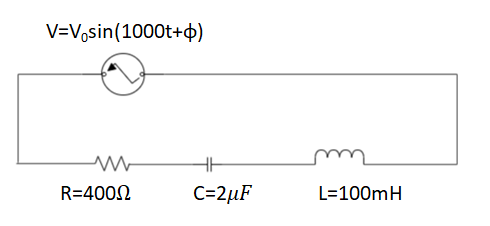
(a) Determine the value of phase difference between the current and the voltage in the given series LCR circuit.
(b) Calculate the value of the additional capacitor which may be joined suitably to the capacitor C that would make the power factor unity.

Answer
504.3k+ views
Hint: In this problem, we must remember the formula of phase difference in a series LCR circuit
refer to the impedances of inductor and capacitor respectively. The power factor of a series LCR circuit is given by
Basic knowledge of net capacitance, when capacitors are in series case and as well as when the capacitors are in parallel case must be known too.
Step by step solution:
The problem consists of a series LCR circuit, where the alternating voltage in the circuit is given by,
Since, the alternating voltage source already shows a phase difference, hence, the impedances
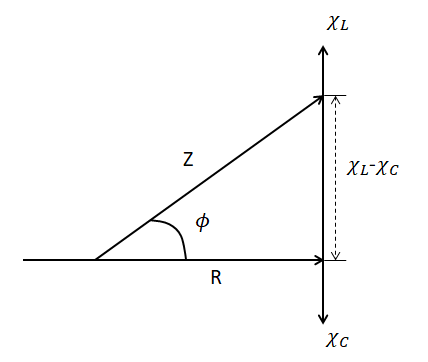
Since, it is a right angled triangle, with the hypotenuse being total impedance of the circuit Z.
Therefore,
We know the impedance of inductance is,
Therefore, the phase angle
The most preferred way to find out the value of the phase angle is using the formula,
We are given that
Substituting in these values of inductive impedance and capacitive impedance, along with the known value of resistor’s resistance (
We know that
Let’s find the power factor now. The power factor is the ratio of the resistor’s resistance (R) and the net impedance of the series LCR circuit (Z). This ratio can also be found out using the impedance triangle as,
In the question, it is given that the power factor is unity (1), therefore R=Z. This implies,
Since, the new capacitance value is greater than the capacitance value already in the circuit, we will have to put an additional capacitor of capacitance
We know that the net capacitance of 2 capacitors in parallel is given by,
That is the additional capacitance to be added in parallel to the initial one is,
Note:
The phase angle
Hence, for the phase angle that we found out,
refer to the impedances of inductor and capacitor respectively. The power factor of a series LCR circuit is given by
Basic knowledge of net capacitance, when capacitors are in series case and as well as when the capacitors are in parallel case must be known too.
Step by step solution:
The problem consists of a series LCR circuit, where the alternating voltage in the circuit is given by,
Since, the alternating voltage source already shows a phase difference, hence, the impedances

Since, it is a right angled triangle, with the hypotenuse being total impedance of the circuit Z.
Therefore,
We know the impedance of inductance is,
Therefore, the phase angle
The most preferred way to find out the value of the phase angle is using the formula,
We are given that
Substituting in these values of inductive impedance and capacitive impedance, along with the known value of resistor’s resistance (
We know that
Let’s find the power factor now. The power factor is the ratio of the resistor’s resistance (R) and the net impedance of the series LCR circuit (Z). This ratio can also be found out using the impedance triangle as,
In the question, it is given that the power factor is unity (1), therefore R=Z. This implies,
Since, the new capacitance value is greater than the capacitance value already in the circuit, we will have to put an additional capacitor of capacitance
We know that the net capacitance of 2 capacitors in parallel is given by,
That is the additional capacitance to be added in parallel to the initial one is,
Note:
The phase angle
Hence, for the phase angle that we found out,
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




