
A dipole is said to be in stable equilibrium when angle between electric field and dipole moment is
A). Zero
B). 180 degree
C). 45 degree
D). 90 degree
Answer
430.5k+ views
3 likes
Hint: In this question, the dipole is kept in a uniform electric field. And we have to write the expressions for them in two different cases. So for solving this, we will keep the dipole at two positions and then, will find the dipole at
Formula used:
The potential energy of the dipole will be equal to the
And for torque, the formula will be
Here,
Complete step-by-step solution:
Let us assume that the dipole we are using is kept at two positions namely (a) and (b) which is placed in a constant electric field
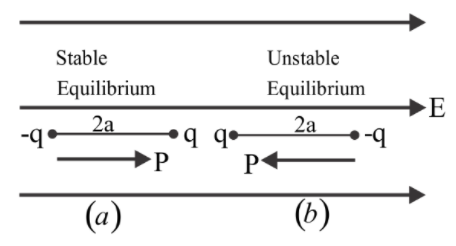
In the first case: When the angle between the dipole and electric field will be zero
Then, the Potential energy of the dipole will be equal to the
Therefore,
And on simplifying the equation, we get
since
Therefore, we can say that the dipole will be in stable equilibrium.
Now we will find the torque,
And for torque, the formula will be
Hence, the option A is correct.
In the second case: When the angle between the dipole and electric field will be
Then, the Potential energy of the dipole will be equal to the
Therefore, we can say that the dipole will be in unstable equilibrium.
Now we will find the torque,
And for torque, the formula will be
Note: Stable equilibrium means the lowest potential energy at the equilibrium point. Therefore the stable equilibrium the torque should be zero and the potential energy of the dipole should be minimum. Whereas the unstable equilibrium torque will be zero and the potential energy should be maximum or positive.
Formula used:
The potential energy of the dipole will be equal to the
And for torque, the formula will be
Here,
Complete step-by-step solution:
Let us assume that the dipole we are using is kept at two positions namely (a) and (b) which is placed in a constant electric field

In the first case: When the angle between the dipole and electric field will be zero
Then, the Potential energy of the dipole will be equal to the
Therefore,
And on simplifying the equation, we get
since
Therefore, we can say that the dipole will be in stable equilibrium.
Now we will find the torque,
And for torque, the formula will be
Hence, the option A is correct.
In the second case: When the angle between the dipole and electric field will be
Then, the Potential energy of the dipole will be equal to the
Therefore, we can say that the dipole will be in unstable equilibrium.
Now we will find the torque,
And for torque, the formula will be
Note: Stable equilibrium means the lowest potential energy at the equilibrium point. Therefore the stable equilibrium the torque should be zero and the potential energy of the dipole should be minimum. Whereas the unstable equilibrium torque will be zero and the potential energy should be maximum or positive.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




