
(a) Draw the plot of binding energy per nucleon (BE/A) as a function of mass number. Write two important conclusions that can be drawn regarding the nature of nuclear sources.
(b) Use this graph to explain the release of energy in both the process of nuclear fusion and fission.
(c) Write the basic nuclear process and the neutron undergoing decay. Why is the detection of neutrinos found very difficult?
Answer
509.1k+ views
Hint: By using the relationship between mass-energy and nuclear binding energy, it is easy to find or understand the concepts of nuclear fission, nuclear fusion and basic nuclear processes like alpha-decay, beta-decay, etc.
Complete step by step answer:
Before going to draw a plot of binding energy per nucleon (BE/A), try to know what is the binding energy.
If a certain number of neutrons and protons are brought together to form a nucleus of a certain charge and mass, energy E will be released in the process. The energy E is called ‘Binding Energy’ of the nucleus.
A more useful measure of the binding energy between the constituents of the nucleus is binding energy per nucleon
Graph of
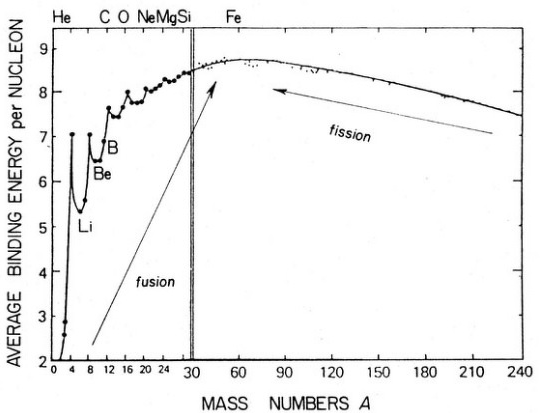
Conclusions from the graph:
From the above graph, Eb is practically constant i.e. independent of the atomic number for nuclei of middle-class numbers (30 < A < 170). The curve is a maximum at about 8.75 MeV for A=56 and 7.6 MeV for A=138.
Eb is lower for both light nuclei (A < 30) and heavy nuclei (A > 170).
The force is attractive and sufficiently strong to produce a binding energy of a few MeV per nucleon.
If a nucleon is at a distance more than a nuclear force from a particular nucleon, it will have no influence on the binding energy of the nucleon under consideration.
A very heavy nucleus, say A=240 has lower binding energy per nucleon compared with another say A=120. If A=240 breaks into two A=120 nuclei, nucleons get more tightly bound.
Consider, very light nuclei joining to form a heavier nucleus. The binding energy per nucleon of a fused heavier nuclei is more than that of the lighter nuclei.
In
example: for
i.e.
Here, conversion of neutrons into protons is done.
for
Here conversion of proton into neutron is done. The emission of electrons in
Neutrinos are neutral particles with very small even zero mass compared to electrons. They have only weak interaction with other particles. Therefore, they are very difficult to detect.
Note:
In
Complete step by step answer:
Before going to draw a plot of binding energy per nucleon (BE/A), try to know what is the binding energy.
If a certain number of neutrons and protons are brought together to form a nucleus of a certain charge and mass, energy E will be released in the process. The energy E is called ‘Binding Energy’ of the nucleus.
A more useful measure of the binding energy between the constituents of the nucleus is binding energy per nucleon
Graph of

Conclusions from the graph:
From the above graph, Eb is practically constant i.e. independent of the atomic number for nuclei of middle-class numbers (30 < A < 170). The curve is a maximum at about 8.75 MeV for A=56 and 7.6 MeV for A=138.
Eb is lower for both light nuclei (A < 30) and heavy nuclei (A > 170).
The force is attractive and sufficiently strong to produce a binding energy of a few MeV per nucleon.
If a nucleon is at a distance more than a nuclear force from a particular nucleon, it will have no influence on the binding energy of the nucleon under consideration.
A very heavy nucleus, say A=240 has lower binding energy per nucleon compared with another say A=120. If A=240 breaks into two A=120 nuclei, nucleons get more tightly bound.
Consider, very light nuclei joining to form a heavier nucleus. The binding energy per nucleon of a fused heavier nuclei is more than that of the lighter nuclei.
In
example: for
i.e.
Here, conversion of neutrons into protons is done.
for
Here conversion of proton into neutron is done. The emission of electrons in
Neutrinos are neutral particles with very small even zero mass compared to electrons. They have only weak interaction with other particles. Therefore, they are very difficult to detect.
Note:
In
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




