Question

A half meter rule was a pivoted 20cm mark. If a mass of 40g is hung at 5cm, find the mass of the ruler.

Answer 1

Hint: As a first step, you could represent the given conditions in a diagram. The scale is balanced about the pivot means that there is no torque acting on it. Now you could find the torque due to the linear forces acting on the scale and then by balancing them, you will get the mass of the ruler.
Formula used:
Torque,
$\tau =F\times r\times \sin \theta $

Complete answer:
In the question we are given a half meter scale, (that is, the length of the scale is 50cm) which is pivoted at the 20cm mark. We are said that a mass of 40g is hung at 5cm and then we are asked to find the mass of the given ruler using the given information.
The given information can be represented as,

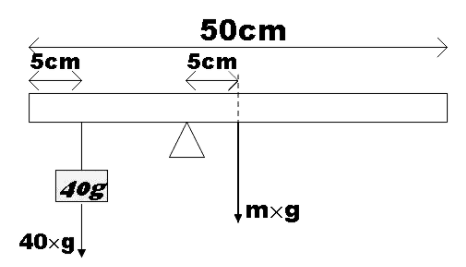
In order for the scale to be in equilibrium, the torque should be balanced about the point at which the scale is pivoted. We have a total of two forces acting on the scale, one is the force due to gravity at the centre point and the other due to the 40g mass hung at 5cm from the end. So, we have torque due to both these forces about the pivoted point.
Now, let us recall the expression for torque. The twisting force that could result in rotation is called torque and it is given by,
$\tau =F\times r\times \sin \theta $
Where, F is the linear force, r is the distance from the axis of rotation to the point at which the linear force is applied and $\theta $ is the angle between the force F and distance r.
The axis of rotation here is the point at which the scale is pivoted. So for the linear force due to the weight of the body downwards the torque is given by,
${{\tau }_{1}}=mg\times \left( 5cm \right)\sin 90{}^\circ $
$\therefore {{\tau }_{1}}=5mg$ ……………………………………… (1)
Now for the linear force due to the 40g mass hung,
${{\tau }_{2}}=40g\left( 15cm \right)\sin 90{}^\circ $
$\therefore {{\tau }_{2}}=40g\times \left( 15 \right)$ ……………………………………. (2)
Since there is no rotation due to these torques about the pivoted point,
${{\tau }_{1}}={{\tau }_{2}}$
$\Rightarrow 5mg=40g\times \left( 15 \right)$
$\Rightarrow 5m=40\times 15$
$\therefore m=120g$
So we found the mass of the given ruler to be 120g.

Note:
The unit of torque is Newton-meter (Nm). You may have seen that while finding the torque, we have taken the angle between r and F to be $90{}^\circ $ as you can clearly see that the linear force due to gravity for both cases lies perpendicular to the distance measured. It is also important for this angle to take into account the direction from the linear force being applied.