
A heavy brass sphere is hung from a spiral spring and it executes vertical vibration with a period T. The ball is now immersed in non viscous liquid with a density one-tenth that of brass. When set into vertical vibration with the sphere remaining inside the liquid all the time, the period will be
Answer
492k+ views
Hint: The vibrations produced in the sphere is a motion called a simple harmonic motion. The time period of a body under simple harmonic motion is given as
Formula used:
Complete step-by-step solution:
Suppose a body is attached to a spring hanging from a ceiling. This is called a spring body system. If we cause a small disturbance in the body, due to the spring force the body will undergo continuous vibrations. This motion of vibrations of the body is called a simple harmonic motion.
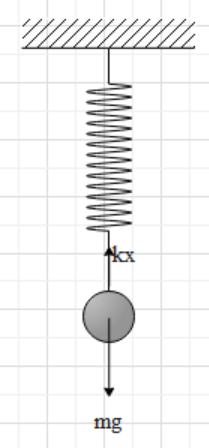
A simple harmonic motion is a period motion. Hence, it has a time period of motion. The time period is defined as the time taken by the body to complete one complete cycle. In a simple harmonic motion, the time period is the time taken by the body to complete one oscillation (to and fro motion).
The time period of the body depends on the spring constant of the spring and the mass of the body.
Suppose the spring constant of the spring is k and the mass of the body is m.
Then the time period of the body is given as
Therefore, the time period of the spring depends on the spring constant and the mass of the body only.
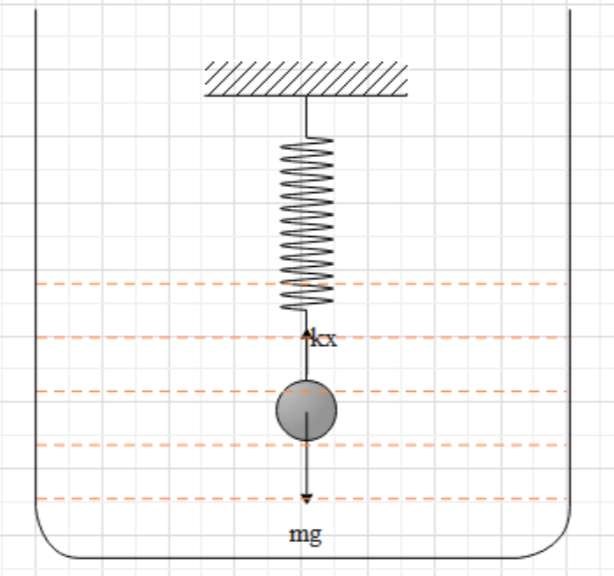
When the system is immersed into a liquid. Neither the spring constant nor the mass of the body changes. Both of the quantities remain the same as they were before immersing in the liquid.
Hence, the time period of the body will remain unchanged.
Therefore, the correct option is C.
Note: Suppose we remove the spring and attach the sphere an inextensible string and produce horizontal vibrations in the sphere. Then the time period of the sphere is given as
Here, l is the length of the string, and
In air,
When we immerse the system in a liquid, due the buoyant force exerted by the liquid on the sphere, the value of
Hence, the time period is more in a liquid than that of in the air.
Formula used:
Complete step-by-step solution:
Suppose a body is attached to a spring hanging from a ceiling. This is called a spring body system. If we cause a small disturbance in the body, due to the spring force the body will undergo continuous vibrations. This motion of vibrations of the body is called a simple harmonic motion.

A simple harmonic motion is a period motion. Hence, it has a time period of motion. The time period is defined as the time taken by the body to complete one complete cycle. In a simple harmonic motion, the time period is the time taken by the body to complete one oscillation (to and fro motion).
The time period of the body depends on the spring constant of the spring and the mass of the body.
Suppose the spring constant of the spring is k and the mass of the body is m.
Then the time period of the body is given as
Therefore, the time period of the spring depends on the spring constant and the mass of the body only.

When the system is immersed into a liquid. Neither the spring constant nor the mass of the body changes. Both of the quantities remain the same as they were before immersing in the liquid.
Hence, the time period of the body will remain unchanged.
Therefore, the correct option is C.
Note: Suppose we remove the spring and attach the sphere an inextensible string and produce horizontal vibrations in the sphere. Then the time period of the sphere is given as
Here, l is the length of the string, and
In air,
When we immerse the system in a liquid, due the buoyant force exerted by the liquid on the sphere, the value of
Hence, the time period is more in a liquid than that of in the air.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




