
A library has to accommodate two different types of books on a shelf. The books are 6cm and 4cm thick and weigh 1kg and 1.5kg respectively. The shelf is 96cm long and at most can support a weight of 21kg. How should the shelf be filled with the books of two types in order to include the greatest number of books? Make it as an LLP and solve it graphically.
Answer
491.4k+ views
Hint: We will first assume the first type of book as x and the second type of book as y. As the thickness of the books are 6cm and 4cm and the width of the shelf is 96cm, we get,
Complete step-by-step solution
It is given in the question that a library has to accommodate two different types of books on a shelf. The books are 6cm and 4cm thick and weigh 1kg and 1.5kg respectively. The shelf is 96cm long and at most can support a weight of 21kg. We should find the maximum number of books that can be arranged on the shelf.
Let us assume that the two types of books are x and y. We have been given that, the thickness of the books are 6cm and 4cm and the width of the shelf is 96cm, so we get,
On dividing the obtained expression with 2, we get,
It is also given that the weight of the books are 1kg and 1.5kg and the maximum capacity of the shelf is 21kg. So, we get,
On multiplying the inequality with 2, we get,
As the values of x and y cannot be less than 0, we can write,
So, we get the maximum LPP as z = x + y.
To, find the points, through which the line 3x + 2y = 48 passes, we will assume some values for x and y, so we can write,
So, for x = 0, we get, (0 + 2y = 48), that is, y = 24 and for y = 0, we get, (3x + 0 = 48), that is, x = 16. Hence, we can write the points through which the line 3x + 2y = 48 passes as (0,24) and (16,0).
Now, to check the feasible region of this line, we will put (0,0) in the equation,
And this condition is true, thus the required feasible region is towards the origin.
Now, to find the points, through which the line 2x + 3y = 42 passes, we will assume some values for x and y, so we can write,
So, for x = 0, we get, (0 + 3y = 42), that is, y = 14 and for y = 0, we get, (2x + 0 = 42), that is, x = 21. Hence, we can write the points through which the line 2x + 3y = 42 passes as (0,14) and (21,0).
Now, to check the feasible region of this line, we will put (0,0) in the equation,
And this condition is true, thus the required feasible region is towards the origin.
Now, let us find the point of intersection of the lines 2x + 3y = 42 and 3x + 2y = 48.
So, we substitute
So, we get
Now, we find the value of y. So, we get
So, the point of intersection of the lines
Now, we will plot the obtained points on the graph and also find the feasible region.
So, first, we will draw the line
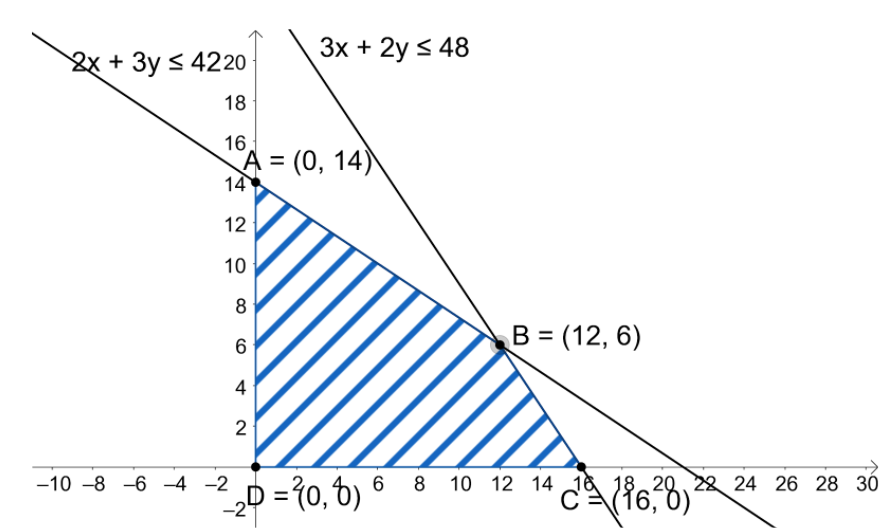
Thus, the feasible region is ABCDA.
So, we get the corner points as A(0,14), B(12,6), C(16,0) and D(0,0).
Now, we will put the values of the corner points in the equation z = x + y, to get the maximum value of z.
Thus, from the above table, we can see that the value of z is maximum at B(12,6), that is 18.
Therefore, the maximum number of books is 18 and the number of books of the first type is 12 and the number of books of the second type is 6.
Note: Majority of the students make mistakes while finding the feasible region, and as a result, they may select the wrong portion of the graph, for calculation, which results in the formation of wrong answers. For checking the feasible region, we have to put the values of x and y as (0,0) in the obtained equations and if the equation is like,
Complete step-by-step solution
It is given in the question that a library has to accommodate two different types of books on a shelf. The books are 6cm and 4cm thick and weigh 1kg and 1.5kg respectively. The shelf is 96cm long and at most can support a weight of 21kg. We should find the maximum number of books that can be arranged on the shelf.
Let us assume that the two types of books are x and y. We have been given that, the thickness of the books are 6cm and 4cm and the width of the shelf is 96cm, so we get,
On dividing the obtained expression with 2, we get,
It is also given that the weight of the books are 1kg and 1.5kg and the maximum capacity of the shelf is 21kg. So, we get,
On multiplying the inequality with 2, we get,
As the values of x and y cannot be less than 0, we can write,
So, we get the maximum LPP as z = x + y.
To, find the points, through which the line 3x + 2y = 48 passes, we will assume some values for x and y, so we can write,
| x | 0 | 16 |
| Y | 24 | 0 |
So, for x = 0, we get, (0 + 2y = 48), that is, y = 24 and for y = 0, we get, (3x + 0 = 48), that is, x = 16. Hence, we can write the points through which the line 3x + 2y = 48 passes as (0,24) and (16,0).
Now, to check the feasible region of this line, we will put (0,0) in the equation,
And this condition is true, thus the required feasible region is towards the origin.
Now, to find the points, through which the line 2x + 3y = 42 passes, we will assume some values for x and y, so we can write,
| x | 0 | 21 |
| Y | 14 | 0 |
So, for x = 0, we get, (0 + 3y = 42), that is, y = 14 and for y = 0, we get, (2x + 0 = 42), that is, x = 21. Hence, we can write the points through which the line 2x + 3y = 42 passes as (0,14) and (21,0).
Now, to check the feasible region of this line, we will put (0,0) in the equation,
And this condition is true, thus the required feasible region is towards the origin.
Now, let us find the point of intersection of the lines 2x + 3y = 42 and 3x + 2y = 48.
So, we substitute
So, we get
Now, we find the value of y. So, we get
So, the point of intersection of the lines
Now, we will plot the obtained points on the graph and also find the feasible region.
So, first, we will draw the line

Thus, the feasible region is ABCDA.
So, we get the corner points as A(0,14), B(12,6), C(16,0) and D(0,0).
Now, we will put the values of the corner points in the equation z = x + y, to get the maximum value of z.
| Corner points | z = x + y |
| A(0,14) | z = 0 + 14 = 14 |
| B(12,6) | z = 12 + 6 = 18 |
| C(16,0) | z = 16 + 0 = 16 |
| D(0,0) | z = 0 + 0 = 0 |
Thus, from the above table, we can see that the value of z is maximum at B(12,6), that is 18.
Therefore, the maximum number of books is 18 and the number of books of the first type is 12 and the number of books of the second type is 6.
Note: Majority of the students make mistakes while finding the feasible region, and as a result, they may select the wrong portion of the graph, for calculation, which results in the formation of wrong answers. For checking the feasible region, we have to put the values of x and y as (0,0) in the obtained equations and if the equation is like,
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE




