
A line AB is parallel to the line CD. This is symbolically written as
Answer
422.1k+ views
Hint: To write the statement given in symbolically form we will use the sign used to denote a line and two parallel lines. Firstly we will write both the lines given with symbols used to denote a line. Then we will use the parallel sign between the two lines and get our desired answer.
Complete step by step solution:
So it is given to us that
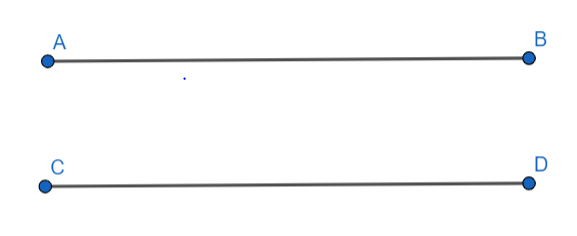
We know that a line is dented by
Line
Line
Next we know that the sign used to denote parallel line is
Hence A line
Note: A line is a one-dimensional figure which has length but doesn't have a width. It is made up of a set of points which is extended in opposite directions infinitely. There are many lines in geometry such as horizontal lines, vertical lines, parallel lines and perpendicular lines. These lines are widely used to construct different polygons. Example- Square is made up of four lines with same length; Rectangle is made up of four lines where the opposite lines are same in length. Parallel lines are those lines that do not intersect or meet each other at any point in the plane or we can say that parallel lines meet at infinity.
Complete step by step solution:
So it is given to us that

We know that a line is dented by
Line
Line
Next we know that the sign used to denote parallel line is
Hence A line
Note: A line is a one-dimensional figure which has length but doesn't have a width. It is made up of a set of points which is extended in opposite directions infinitely. There are many lines in geometry such as horizontal lines, vertical lines, parallel lines and perpendicular lines. These lines are widely used to construct different polygons. Example- Square is made up of four lines with same length; Rectangle is made up of four lines where the opposite lines are same in length. Parallel lines are those lines that do not intersect or meet each other at any point in the plane or we can say that parallel lines meet at infinity.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

How many crores make 10 million class 7 maths CBSE

Fill in the blanks with appropriate modals a Drivers class 7 english CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

What were the major teachings of Baba Guru Nanak class 7 social science CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE





