
A line perpendicular to the line segment joining the points (1,0) and (2,3) divides it in the ratio 1: n. Find the equation of the line.
Answer
499.5k+ views
Hint: In order to solve this problem, we need to understand that the line is divided in a certain ratio and the coordinates of that point are given by section formula. Section formula says that if the segment is divided in ratio m:n between the two coordinates namely
Complete step-by-step answer:
We are given a with endpoints (1,0) and (2,3) divided in the ratio of 1: n.
We need to find the coordinates of the point that divides the segment.
We can use the section formula for this.
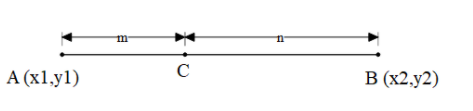
Section formula says that if the segment is divided in ratio m:n between the two coordinates namely
Now for this question let
Applying the formula, we get the coordinates of C as follows,
Solving this the coordinates of points C will be,
Now we need to find the slope of this line.
The formula for the slope with the same two coordinates
Substituting the values, we get,
Now we need to find the slope of the line perpendicular to this line.
But, the product of the slopes of two lines perpendicular to each other is -1.
Hence the slope of other line
Now we have to slope and the one point of the line that we have to calculate.
The formula to the equation of the line is as follows,
Substituting
Solving this equation, we get,
Therefore, the equation of the line is
Note: In this problem, while using the section formula the order is very important as the ratio is not the same, therefore, it is very important of which number multiplies to which number. While calculating the slope can start from any point because as it is the ratio the sign gets neutralized.
Complete step-by-step answer:
We are given a with endpoints (1,0) and (2,3) divided in the ratio of 1: n.
We need to find the coordinates of the point that divides the segment.
We can use the section formula for this.

Section formula says that if the segment is divided in ratio m:n between the two coordinates namely
Now for this question let
Applying the formula, we get the coordinates of C as follows,
Solving this the coordinates of points C will be,
Now we need to find the slope of this line.
The formula for the slope with the same two coordinates
Substituting the values, we get,
Now we need to find the slope of the line perpendicular to this line.
But, the product of the slopes of two lines perpendicular to each other is -1.
Hence the slope of other line
Now we have to slope and the one point of the line that we have to calculate.
The formula to the equation of the line is as follows,
Substituting
Solving this equation, we get,
Therefore, the equation of the line is
Note: In this problem, while using the section formula the order is very important as the ratio is not the same, therefore, it is very important of which number multiplies to which number. While calculating the slope can start from any point because as it is the ratio the sign gets neutralized.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




