Questions & Answers
Question
Answers
Related Questions
A man on the top of a vertical observation tower observes a car moving at a
uniform speed coming directly towards it. If it takes 12 minutes for the angle of
depression to change from \[{30^0}\] to ${45^0}$. How soon after this will the car reach the
observation tower?
\[
(A){\text{ 14min 3 sec}} \\
(B){\text{ 15min 49sec}} \\
(C){\text{ 16min 23sec}} \\
(D){\text{ 18min 5sec}} \\
\]
Answer
![Verified]()
Verified
Verified
Hint: Draw figure and then use trigonometry identity $\tan \theta = \dfrac{{Perpendicular}}{{Base}}$.
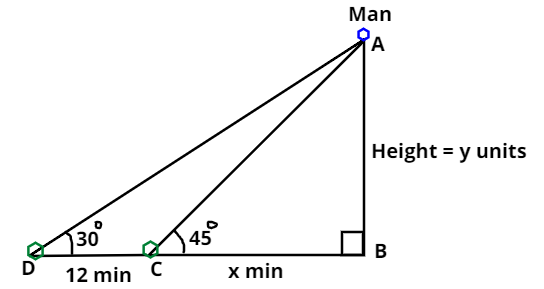
Above figure is drawn with respect to the given conditions in question.
As we can see from the above figure that,
Man is on the top of a vertical tower.
And to change angle of depression from \[{30^0}\] to ${45^0}$ i.e. \[\angle {\text{ADB}}\] to \[\angle {\text{ACB}}\].
It takes 12 minutes,
And it is obvious that when the car will reach the observation tower,
then the angle of depression will be ${90^0}$.
Let the height of the tower be $y$ units.
As we are given that the time taken to travel DC (see in figure) is 12 minutes.
Let the time taken to travel CB will be $x$ minutes.
Here we are known with perpendicular and base of \[\Delta {\text{ABC}}\] and \[\Delta {\text{ABD}}\].
So, we will only use that trigonometric functions, that include perpendicular and base
So, as we know that, $\tan \theta = \dfrac{{Perpendicular}}{{Base}}$.
So, as we can see from the above figure, $\tan {45^0} = \dfrac{{AB}}{{CB}} = \dfrac{y}{x}$.
So, $x = y$ ……………………………………….(1)
And, $\tan 30^\circ = \dfrac{{AB}}{{DB}} = \dfrac{{AB}}{{DC + CB}} = \dfrac{y}{{12 + x}}$.
Now, putting the value of $\tan {30^0}$ and $y$ from equation 1. We get,
$\dfrac{1}{{\sqrt 3 }} = \dfrac{x}{{12 + x}} \Rightarrow \left( {\sqrt 3 - 1} \right)x = 12 \Rightarrow x = \dfrac{{12}}{{\left( {\sqrt 3 - 1} \right)}} \approx 16.38$minutes
Now, as we have defined above that time taken to travel CB is x minutes.
So, time taken to reach the observation tower will be x minutes.
So, according to the options given in the question
the most appropriate answer will be 16min 23 sec.
Hence, the correct Option will be C.
Note: Whenever we come up with these types of problems first, we should draw a figure according to the given conditions in question. And then we will assume time taken to reach the tower as x and then after using trigonometric functions like \[{\text{tan}}\theta \], we can get the value of x using angle of depression and time taken to change angle of depression. This will be the easiest and efficient way to reach the required solution of the problem.
Above figure is drawn with respect to the given conditions in question.
As we can see from the above figure that,
Man is on the top of a vertical tower.
And to change angle of depression from \[{30^0}\] to ${45^0}$ i.e. \[\angle {\text{ADB}}\] to \[\angle {\text{ACB}}\].
It takes 12 minutes,
And it is obvious that when the car will reach the observation tower,
then the angle of depression will be ${90^0}$.
Let the height of the tower be $y$ units.
As we are given that the time taken to travel DC (see in figure) is 12 minutes.
Let the time taken to travel CB will be $x$ minutes.
Here we are known with perpendicular and base of \[\Delta {\text{ABC}}\] and \[\Delta {\text{ABD}}\].
So, we will only use that trigonometric functions, that include perpendicular and base
So, as we know that, $\tan \theta = \dfrac{{Perpendicular}}{{Base}}$.
So, as we can see from the above figure, $\tan {45^0} = \dfrac{{AB}}{{CB}} = \dfrac{y}{x}$.
So, $x = y$ ……………………………………….(1)
And, $\tan 30^\circ = \dfrac{{AB}}{{DB}} = \dfrac{{AB}}{{DC + CB}} = \dfrac{y}{{12 + x}}$.
Now, putting the value of $\tan {30^0}$ and $y$ from equation 1. We get,
$\dfrac{1}{{\sqrt 3 }} = \dfrac{x}{{12 + x}} \Rightarrow \left( {\sqrt 3 - 1} \right)x = 12 \Rightarrow x = \dfrac{{12}}{{\left( {\sqrt 3 - 1} \right)}} \approx 16.38$minutes
Now, as we have defined above that time taken to travel CB is x minutes.
So, time taken to reach the observation tower will be x minutes.
So, according to the options given in the question
the most appropriate answer will be 16min 23 sec.
Hence, the correct Option will be C.
Note: Whenever we come up with these types of problems first, we should draw a figure according to the given conditions in question. And then we will assume time taken to reach the tower as x and then after using trigonometric functions like \[{\text{tan}}\theta \], we can get the value of x using angle of depression and time taken to change angle of depression. This will be the easiest and efficient way to reach the required solution of the problem.
×
Sorry!, This page is not available for now to bookmark.
Like this
Liked
.png)
-
LIVECourses
-
Crash Courses 2021
-
Vedantu Pro
-
Vedantu Assist
-
Class 12
-
-
JEE
-
JEE Main & Advanced 2021
-
JEE Main & Advanced 2022
-
JEE Main & Advanced 2023
-
-
NEET
-
NEET 2021
-
NEET 2022
-
NEET 2023
-
-
NDA
-
CBSE
-
Commerce
-
Class 11
-
Class 12
-
-
ICSE
-
Maharashtra Board
-
Micro Courses
-
-
Reading & Spoken English -
FREEMaster Classes
-
PREMIUM1-on-1 Classes
-
Study Material
-
NCERT Solutions
-
Previous Year Papers
-
Mock Tests
-
Sample Papers
-
Reference Book Solutions
-
ICSE Solutions
-
School Syllabus
-
Revision Notes
-
Important Questions
-
Math Formula Sheets
-
-
More
Book your FREE Online counselling session
Share your contact information
Your FREE Online counselling session has been booked!
Vedantu academic counsellor will be calling you shortly for your Online Counselling session.
DONE
Please try again later
OK
NCERT Book Solutions
Reference Book Solutions
Toll Free:
1800-120-456-456
Mobile:
+91 988-660-2456
Toll Free:
1800-120-456-456
Mobile:
+91 988-660-2456
(Mon-Sun: 9am - 11pm IST)
Questions?
Chat with us
