Elastic Collisions in One Dimension - JEE Important Topic

In our lives, we have witnessed many kinds of collisions like when two vehicles bump into each other causing an accident, a striker hitting the coins on a carrom board and so forth. A collision could be defined as a phenomenon where any two distinct and separate bodies come into contact with each other for a short span of time. Any bodies which are colliding with each other possess momentum due to their mass and velocity. Along with the momentum, every mass has its own energy within it. During the collision, the momentum and the energy of the bodies that collide will change. 

Suppose let’s say there are two bodies of masses, m1 and m2  in which m2  is at rest and also m1 > m2. When $m_1$ is travelling in a one-dimensional motion and eventually hits m2 which is at rest then the two bodies m1 and m2 move in different directions. Here the m2 particle moves in one dimension from rest in a different direction. 

Let us understand how these collisions are divided into categories like an elastic collision in one dimension and an inelastic collision in one dimension and how to explain elastic collision in one dimension with proper derivation. 

Elastic Collision in One Dimension 

There is an important law called the law of conservation of momentum in which the kinetic energy is conserved before and after collisions. Based on whether this law is satisfied or not, the collisions are divided into two types and those are elastic and inelastic collisions. 

Now, let us discuss elastic collision in one dimension. In this kind of collision, the total momentum of the system and the total energy of the system is conserved. The mechanical energy of the two bodies is not converted to any other type of energy during or after the collision. Let us assume there are two bodies which are travelling in one-dimensional motion. Say their masses are m1 and m2, where the initial velocities of m1 and m2 are u1 and u2 whereas the velocities after an elastic collision of both bodies are v1 and v2 as shown in the figure.

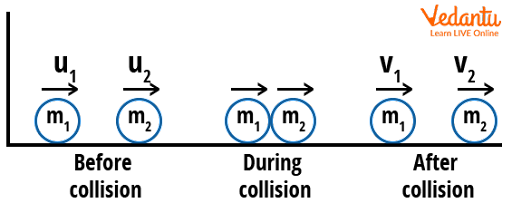
Two masses before, during and after collision

Therefore, the momentum of m1 and m2 before collision are m1u1 and m2u2 whereas the momentum of those bodies after the collision are m1v1 and m2v2. Therefore, according to the law of conservation of momentum, the total momentum of the two bodies before and after the collision should remain constant without any change. 

I.e.,  m1u1 + m2u2 = m1v1 + m2v2  

Grouping the $m_1$ and $m_2$ constituents together, we obtain 

m1u1 – m1v1 = m2v2  – m2u2

I.e.,  m1(u1 – v1) = m2(v2 – u2)  - - - - - - - (1)  

And we know that the kinetic energy of an object with mass m and a velocity v is 

given by $\dfrac{1}{2} \mathrm{mv}^{2}$. Therefore, as the kinetic energy is also conserved, the kinetic energies of the system before and after the collision would remain constant. 

I.e., $\dfrac{1}{2} \mathrm{~m}_{1} \mathrm{u}_{1}^{2}+\dfrac{1}{2} \mathrm{~m}_{2} \mathrm{u}_{2}^{2}=\dfrac{1}{2} \mathrm{~m}_{1} \mathrm{v}_{1}^{2}+\dfrac{1}{2} \mathrm{~m}_{2} \mathrm{v}_{2}^{2}$     

Therefore, we can rewrite the equation as follows. 

m1u12 +  m2u22  =   m1v12 + m2v22 

Getting the m1 constituents and m2 constituents on two sides,  

m1u12  – m1v12 = m2v22 – m2u22 

m1(u12  –  v12)  =   m2(v22 –  u22)  

This can be expanded as shown below, 

m1(u1 + v1)(u1 – v1) = m2(v2 + u2)(v2 – u2)  - - - - - - - (2)

We obtained the final equations after using both the laws of conservation of momentum and kinetic energy in an elastic collision in one dimension. Now, Let’s divide the equation 2 by equation 1 we get, 

$\dfrac{m_{1}\left(u_{1}+v_{1}\right)\left(u_{1}-v_{1}\right)}{m_{1}\left(u_{1}-v_{1}\right)}=\dfrac{m_{2}\left(v_{2}+u_{2}\right)\left(v_{2}-u_{2}\right)}{m_{2}\left(v_{2}-u_{2}\right)}$

By solving the above equation, we get 

u1 + v1 = u2 + v2 

I.e.,  v1  = u2 + v2 - u1 - - - - - - (3) 

From the law of conservation of momentum, we can write that 

As       m1u1 + m2u2 = m1v1 + m2v2  

Substituting equation three, i.e. u2 + v2 - u1  in place of v1 

m1u1 + m2u2  = m1(u2 + v2 - u1) + m2v2  

By solving it we get, 

2m1u1 + (m2-m1)u2   = (m1 + m2)v2 

Therefore, $v_{2}=\dfrac{2 m_{1} u_{1}+\left(m_{2}-m_{1}\right) u_{2}}{\left(m_{1}+m_{2}\right)}$ - - - - - (4) 

This is the value of v2 that we obtained. 

Now let us substitute this value in equation 3 to obtain v1

v1  = v2 + u2 - u1 

I.e., $\mathrm{v}_{1}=\dfrac{2 m_{1} u_{1}+\left(m_{2}-m_{1}\right) u_{2}}{\left(m_{1}+m_{2}\right)}+\mathrm{u}_{2}-\mathrm{u}_{1}$ 

Therefore, $v_{1}=\dfrac{2 m_{1} u_{1}+\left(m_{2}-m_{1}\right) u_{2}+\left(m_{1}+m_{2}\right) u_{2}-\left(m_{1}+m_{2}\right) u_{1}}{\left(m_{1}+m_{2}\right)}$ 

After further solvation of the above equation, we obtain 

$\mathrm{v}_{1}=\dfrac{2 m_{2} u_{2}+\left(m_{1}-m_{2}\right) u_{1}}{m_{1}+m_{2}}$                                              - - - - - - (5) 

Therefore, we obtained the two equations for v1 and v2 which are equation 4 and equation 5. This is the elastic collision in one dimension derivation. If we assume that the masses of the two bodies that are moving in one dimension are equal, then when we substitute, m1 = m2 in the above equations (4) and (5). We get that  v1 = u2 and v2 = u1

We can notice that the velocities of the masses have been exchanged after the collision. Say there are two bodies with the same masses where one body is at rest and one body is travelling with a velocity v. When these two collide, the velocity v is passed on the body which is at rest and the body which was travelling with the v velocity comes to rest.  Therefore, let us discuss the inelastic collision in one dimension.

Inelastic Collision in One Dimension

In this collision, the law of momentum is conserved while the kinetic energy is not conserved. Also, the mechanical energy of the two bodies can be transformed into other types of energy like heat or light. The bodies which are colliding will move in the same direction by either sticking to each other or in a separate manner. 

Now, we shall apply the laws of conservation of momentum and energy here. Say m1 and m2 are the two masses whose initial velocities are u1 and u2  but they move along with the same velocity say ‘v’. 

The Law of conservation of momentum gives us,  

m1u1 + m2u2 = (m1 + m2)v 

Therefore the equation of v is given by,
$v=\dfrac{m_{1} u_{1}+m_{2} u_{2}}{m_{1}+m_{2}}$ 

By the law of conservation of energy, along with the kinetic energy after the collision, there is a release of heat energy. Therefore, the equation is written as follows, 

$\dfrac{1}{2} \mathrm{~m}_{1} \mathrm{u}_{1}^{2}+\dfrac{1}{2} \mathrm{~m}_{2} \mathrm{u}_{2}^{2}=\dfrac{1}{2}\left(\mathrm{~m}_{1}+\mathrm{m}_{2}\right) \mathrm{v}^{2}+\mathrm{Q}$

Where Q is the heat energy.

Conclusion

Therefore we learned how to discuss elastic collision in one dimension and inelastic collision in one dimension with proper formulae and also the elastic collision in one dimension derivation. The confusion about how to explain elastic collision in one dimension will be cleared out through this article.