
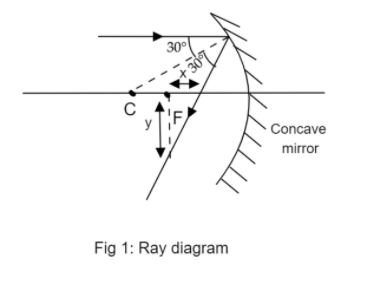
A marginal ray is falling on a concave spherical mirror as shown in the figure below. The ratio of longitudinal (x) and transverse (y) spherical aberration is:
(a)
(b)
(c)
(d) can’t be determined

Answer
498.6k+ views
Hint: This question doesn’t involve much physics, it can be solved by applying concepts of geometry and trigonometry. The reflected ray acts as a transverse for the parallel lines (incident ray and optical axis).
Formula used:
1. Ratio of perpendicular and base for a right angled triangle:
Where,
Complete step by step answer:
The given ray diagram is:
To find: The ratio of longitudinal (x) and transverse (y) spherical aberration.
Step 1 of 2:
The marginal ray given in the diagram is parallel to the optical axis. The reflected ray cuts a pair of these parallel lines. Therefore, the angle it makes with the marginal ray will be equal to the angle it makes with the optical axis.
The angle made by reflected ray with the marginal ray is the summation:
Step 2 of 2:
Consider the triangle
Inverting this to find the required ratio
The ratio of longitudinal (x) and transverse (y) spherical aberration is
Note:
Here, one should take care that just outside the metal surface, for point P metal plate appears infinitely big, therefore, we can use field produced due to infinite metal plate carrying charge approximation.
Formula used:
1. Ratio of perpendicular and base for a right angled triangle:
Where,
Complete step by step answer:
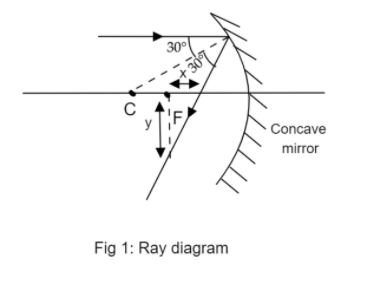
The given ray diagram is:

To find: The ratio of longitudinal (x) and transverse (y) spherical aberration.
Step 1 of 2:
The marginal ray given in the diagram is parallel to the optical axis. The reflected ray cuts a pair of these parallel lines. Therefore, the angle it makes with the marginal ray will be equal to the angle it makes with the optical axis.
The angle made by reflected ray with the marginal ray is the summation:
Step 2 of 2:
Consider the triangle
Inverting this to find the required ratio
The ratio of longitudinal (x) and transverse (y) spherical aberration is
Note:
Here, one should take care that just outside the metal surface, for point P metal plate appears infinitely big, therefore, we can use field produced due to infinite metal plate carrying charge approximation.
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




