
What is a parallel plate capacitor? Deduce the expression for the capacitance when a dielectric slab is inserted between its plates. Assume that slab thickness is less than the plate separation. Also find the expression for energy stored per unit volume of a capacitor.
Answer
439k+ views
Hint: Capacitance of a capacitor is defined as its ability to store electrical energy in form of charge. Mathematically, It's the ratio of charge on one plate to potential difference between conductors.
To deduce expression for the capacitance, calculate voltage between both the plates by determining electric field and substitute in capacitance formula.
Formula Used:
Capacitance,
Complete answer:
A parallel plate capacitor is a system of two conducting plates each of area A, having charges +Q and –Q and separated by a small distance, d. This setup is used to store charge.
Capacitance of a capacitor is its ability to store charge and is given by
Where Q is charged on each conductor and V is the potential between them.
Let us obtain expression for a parallel plate capacitor with a dielectric inserted between its plates.
Consider a parallel plate capacitor with plate area, A and separation between plates, d. Let us assume that a thin dielectric of thickness t is inserted between the plates.
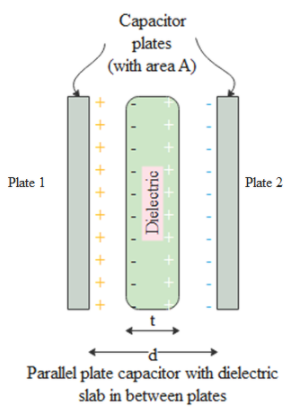
If Q and –Q are the charges on plate 1 and plate 2 respectively, the electric field between the plates outside the dielectric is
Where
Electric field between the plates inside the dielectric is
So the net potential between the plates is,
Since
The capacitance of parallel plate capacitor is,
Now let’s obtain an expression for energy stored per unit volume of the capacitor. The parallel plate capacitor is placed in vacuum, then
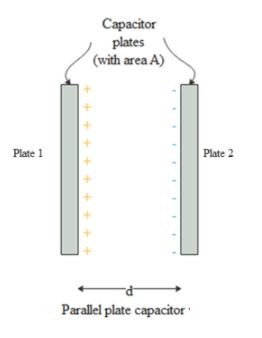
The force on plate 2 due to plate 1 is given by
Work done to displace the plate from its fixed position is,
Work done is equal to increase in energy of the system,
Volume in which electric field is created is given by
So energy stored per unit volume is given by,
Since electric field intensity is
Note:
When a dielectric of thickness equal to separation between the plates of the parallel plate capacitor is inserted, the capacitance of the capacitor increases by a multiple of the dielectric constant. If C and C’ are the capacitance without dielectric and with dielectric respectively then,
To deduce expression for the capacitance, calculate voltage between both the plates by determining electric field and substitute in capacitance formula.
Formula Used:
Capacitance,
Complete answer:
A parallel plate capacitor is a system of two conducting plates each of area A, having charges +Q and –Q and separated by a small distance, d. This setup is used to store charge.
Capacitance of a capacitor is its ability to store charge and is given by
Where Q is charged on each conductor and V is the potential between them.
Let us obtain expression for a parallel plate capacitor with a dielectric inserted between its plates.
Consider a parallel plate capacitor with plate area, A and separation between plates, d. Let us assume that a thin dielectric of thickness t is inserted between the plates.

If Q and –Q are the charges on plate 1 and plate 2 respectively, the electric field between the plates outside the dielectric is
Where
Electric field between the plates inside the dielectric is
So the net potential between the plates is,
Since
The capacitance of parallel plate capacitor is,
Now let’s obtain an expression for energy stored per unit volume of the capacitor. The parallel plate capacitor is placed in vacuum, then

The force on plate 2 due to plate 1 is given by
Work done to displace the plate from its fixed position is,
Work done is equal to increase in energy of the system,
Volume in which electric field is created is given by
So energy stored per unit volume is given by,
Since electric field intensity is
Note:
When a dielectric of thickness equal to separation between the plates of the parallel plate capacitor is inserted, the capacitance of the capacitor increases by a multiple of the dielectric constant. If C and C’ are the capacitance without dielectric and with dielectric respectively then,
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




