Question

A path 2m wide surrounds a circular pond of diameter 40m. How many cubic meters of gravel is required to grave the path to a depth of 20cm?

Answer 1

Hint: In this question first identify the given dimensions and also remember to find the volume hollow cylinder and use the formula $\text{Volume of hollow cylinder} = \pi \left( {{R^2} - {r^2}} \right)h$ here R is the radius of the cylinder by the outer circle formed by the path and r is the radius of the circular pond, use this information to approach the solution.

Complete step-by-step solution:
According to the given information we have a circular pond with 40m diameter which is surrounded by a path of 2m wide and we have to grave the path to the depth of 20 cm
Thus, we have to find the volume of the path to the depth of 20 cm
So, the radius of pond $\left( r \right) = \dfrac{{40}}{2} = 20m$

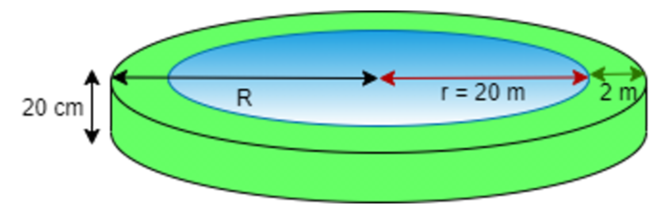
So, as in the above diagram we can see that we have 2 cylinder one with radius R and one with radius r = 20 m
As we have to find the volume of path to 20 cm depth which will be equal to volume of cylinder with radius R – volume of cylinder with radius r
So, we know that thickness of path = 2m
Thickness of path = R – r
Substituting the values in the above we get
$2 m = R – 20 m$
$ \Rightarrow R = 20 + 2 = 22 m$
And depth$\left( h \right) = 20cm = \dfrac{{20}}{{100}}m = 0.2m$
Now we know that volume of cylinder is given as; $\text{Volume} = \pi {r^2}h$ here r is the radius of cylinder and h is the height of the cylinder
So, volume of path = volume of cylinder of radius R – volume of cylinder of radius r
Substituting the values in the above equation we get
Volume of path = $\pi {R^2}h - \pi {r^2}h$
$ \Rightarrow $ Volume of path = $\pi \left( {{R^2} - {r^2}} \right)h$
Substituting the given values in the above equation we get
$ \Rightarrow $ Volume of path = $\dfrac{{22}}{7}\left( {{{\left( {22} \right)}^2} - {{\left( {20} \right)}^2}} \right) \times \left( {0.2} \right)$
$ \Rightarrow $ Volume of path = $\dfrac{{22}}{7} \times \left( {484 - 400} \right) \times \left( {0.2} \right)$
$ \Rightarrow $ Volume of path = $3.14 \times 84 \times 0.2$
$ \Rightarrow $ Volume of path = $52.752{m^3}$
So, the Volume of gravel to require to grave the path is $52.752{m^3}$

Note: The trick behind such question is to first draw a diagram with the given information and then to find the volume of gravel to require to grave we have to find the volume of the path so we just have to divide the diagram into 2 parts such that after subtracting there volume the result would come volume of the path and to do so we have to find the radius of the outer cylinder and then directly substitute the values in the formula of volume of the cylinder and we will get the required result.